Question

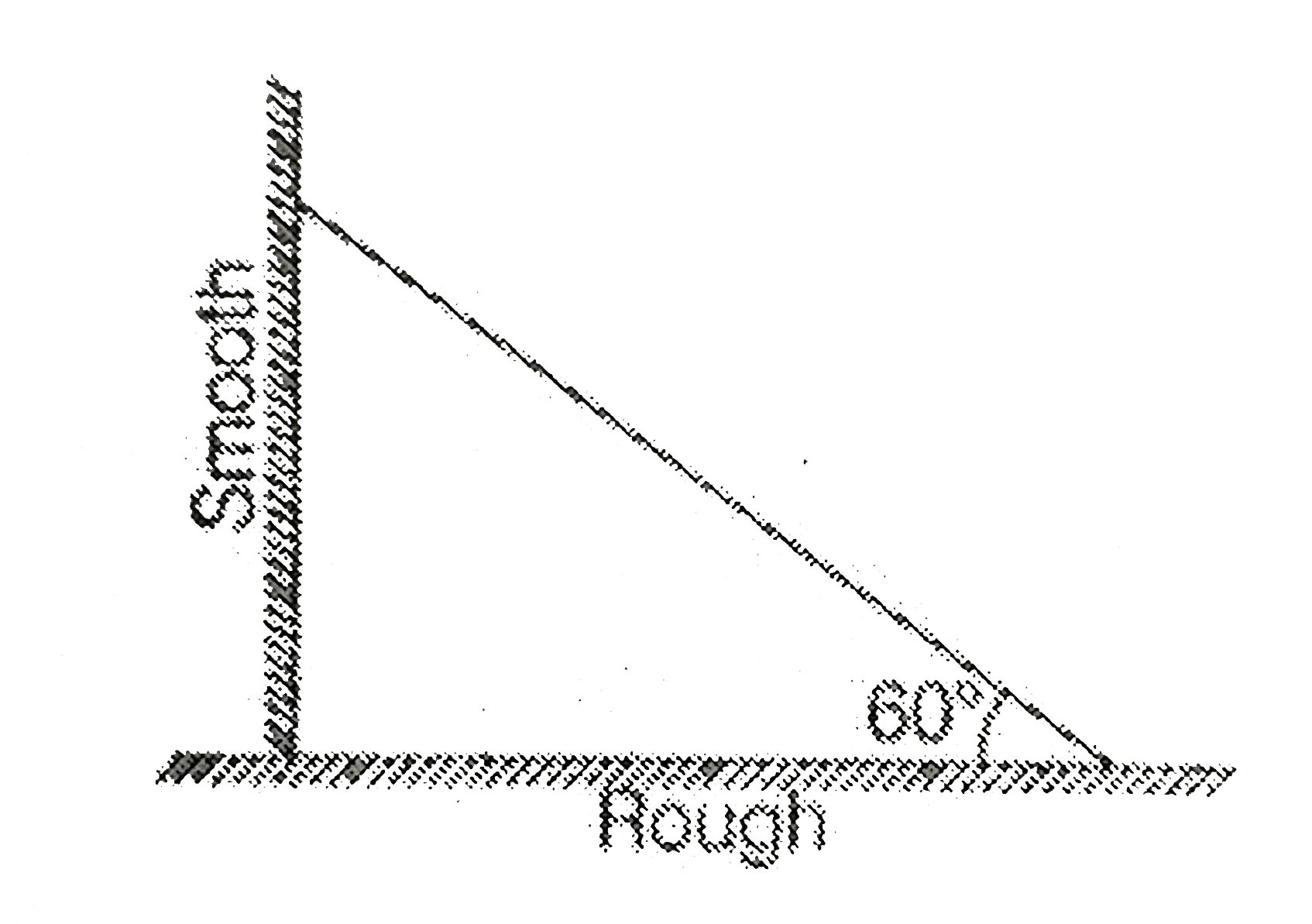
A $4m$ long ladder weighing $25kg$ rests with its upper end against a smooth wall and lower end on rough ground.What should be the minimum coefficient of friction between the ground and the
ladder for it to be inclined at ${60}^{\circ }$ with the horizontal without slipping? $(Takeg=10m/s^2)$

Options

A. a. 0.19
B. b. 0.29
C. c. 0.39
D. d. 0.49

Answer

B

Explanation

To solve the problem of determining the minimum coefficient of friction required for a 4 m long ladder weighing 25 kg to remain stable while inclined at an angle of $${60}^{\circ }$$ with the horizontal, we will follow these steps:

Step 1: Identify the forces acting on the ladder
1. Weight of the ladder (W): This acts vertically downward at the center of the ladder.
\( W = mg = 25 \, \text{kg} \times 10 \, \text{m/s}^2 = 250 \, \text{N} \)
2. Normal forces:
- \(N_1\) acts vertically upward at the base of the ladder (ground).
- \(N_2\) acts horizontally from the wall (since the wall is smooth, it only provides a horizontal reaction).
3. Frictional force (F_r): This acts horizontally at the base of the ladder, opposing the tendency to slip.

Step 2: Set up the equilibrium equations
For the ladder to be in equilibrium:
- The sum of vertical forces must be zero.
- The sum of horizontal forces must be zero.
- The sum of moments about any point must also be zero.

Vertical Forces:
\( N_2 = W \quad \text{(1)} \)

Horizontal Forces:
\( F_r = N_1 \quad \text{(2)} \)

Step 3: Calculate moments about the base of the ladder
Taking moments about the base of the ladder (point O):
- The moment due to the weight of the ladder (acting at its center, 2 m from the base):
\( \text{Moment due to } W = W \cdot \left(2 \cos(60^\circ)\right) = 250 \cdot (2 \cdot 0.5) = 250 \, \text{N} \cdot 1 = 250 \, \text{Nm} \)
- The moment due to the normal force from the wall:
\( \text{Moment due to } N_1 = N_1 \cdot (4 \sin(60^\circ)) = N_1 \cdot (4 \cdot \frac{\sqrt{3}}{2}) = 2\sqrt{3} N_1 \)

Setting the moments equal for equilibrium:
\( N_1 \cdot (2\sqrt{3}) = 250 \quad \text{(3)} \)

Step 4: Substitute \(N_1\) from equation (2) into equation (3)
From equation (2):
\( N_1 = F_r = \mu N_2 \)
Substituting \(N_2 = W\) from equation (1):
\( N_1 = \mu W = \mu \cdot 250 \)
Substituting this into equation (3):
\( \mu \cdot 250 \cdot (2\sqrt{3}) = 250 \)
Dividing both sides by 250:
\( \mu \cdot 2\sqrt{3} = 1 \)
Solving for \(\mu\):
\( \mu = \frac{1}{2\sqrt{3}} = \frac{\sqrt{3}}{6} \)

Step 5: Calculate the numerical value of \(\mu\)
Using \(\sqrt{3} \approx 1.732\):
\( \mu \approx \frac{1.732}{6} \approx 0.2887 \)

Final Answer
The minimum coefficient of friction required is approximately:
\( \mu \approx 0.29 \)