Two batteries of emf e1ande2 having internal resistance r1andr2 respectively are connected in series to an external resistance R. Both the batteries are getting discharged. The above described combination of these two batteries has to produce a weaker current than when any one of the batteries is connected to the same resistor. For this requirement to be fulfilled
ε2ε1must not lie betweenr2r1+Randr1r2+R
ε2ε1must not lie betweenr2r1+Randr2+Rr1
ε2ε1must lie betweenr2r1+Randr1r2+R
ε2ε1must not lie betweenr2r1+Randr2+Rr1
The correct Answer is:B
Let R' be the resistance of the heater of coil.
R'=V2P=(100)21000>10Ω
If the heater has to operature with a power P'=62.5W, the voltage V' across its coil should be
V'=(P'R')1/2=(6.25×10)1/2=25VA
Thus, out of 100V, a valtage drop of 25V occurs across the heater and the rest 100-25=75V occurs across the 10Ω resistor. Therefore current in the cicruit is I=7510=7.5A
Now, current throght the heater =V'R=2510=2.5A
Therefore, current throgh reistor R=7.5-2.5=5.0A
Here,R=V'5.0A=25V5.0=5Ω
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - EXEMPLAR PROBLEMS
6 Videos
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - CORNER
15 Videos
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - POTENTIOMETER
7 Videos
- COMMUNITCATION SYSTEMSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - COMMUNITCATION SYSTEMSEXERCISE - Assertion And Reason
30 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
Similar Questions
The two batteries of emf E1 and E2 having internal resistances r1 and r2 respectively are connected in series to an external resistor R. Both the batteries are gatting discharged. The above described combination of these two batteries has to produce a weaker current then when any one of the battery is connected to same resistor. For this requirement to be fulfilled.
View SolutionTwo batteries of emf E1andE2(E2>E1) and internal resistances r1andr2 respectively are connected in parallel as shown in figure.
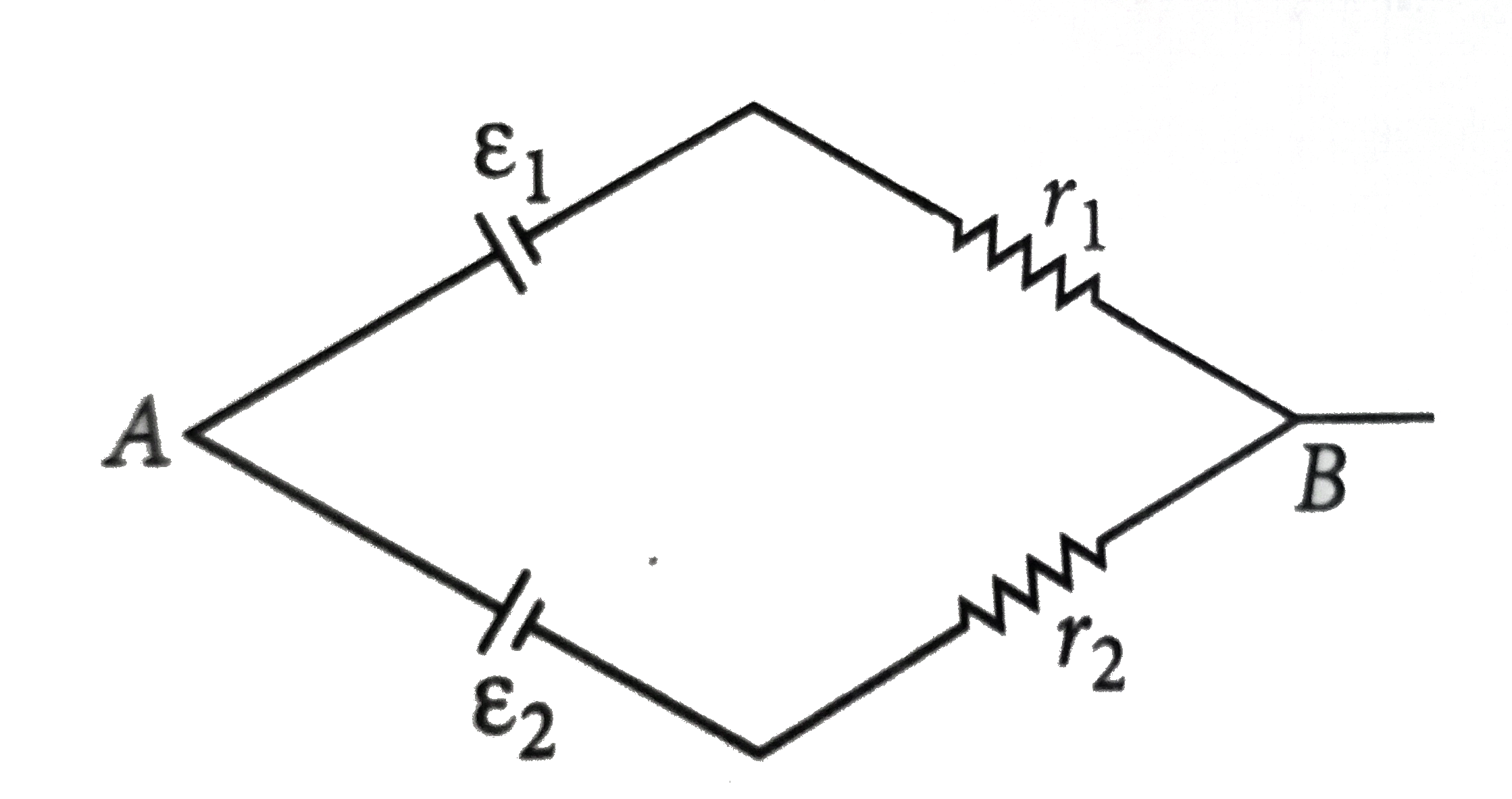
View Solution
Knowledge Check
Two batteris of emf ε1 and ε2 wit respective internal resistance r1 and r2 are connected in parallel. Now
AThe effective emf of this parallel combination is (ε1r1+ε2r2)(r1+r2)BThe effective internal resistance of this combination is 2r1r2(r1+r2)Cthe effective emf of this parallel combination is (ε1r2+ε2r1)(r1+r2)DThe effective internal resistance of this combination is r1r2(r1+r2)Three batteries of emf E1,E2 and E3 and internal resistance r1,r2 and r3 are connected as shown. The current through the battery of emf E3 is zero if.
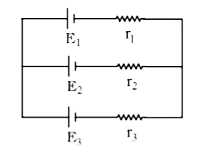 AE1r2+E2r1=E3(r1+r2)BE1r2−E2r1=E3(r1+r2)CE1r2+E2r1=E3(r1+r2)DE1r1+E2r2=E3(r1+r2)
AE1r2+E2r1=E3(r1+r2)BE1r2−E2r1=E3(r1+r2)CE1r2+E2r1=E3(r1+r2)DE1r1+E2r2=E3(r1+r2)N batteries each of emf E and internal resistance r are first connected in series and then in parallel to an external resistance R. if current through R is same in both cases then.
AR=r2/NBR−Nr/2CR=NrDR=r
Two batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionTwo batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionTwo batteries of emf E1 and E2(E2>E1) and internal resistance r1 and r2 respectively are connected in parallel as shown in the figure. Then, which of the followings statements is correct ?
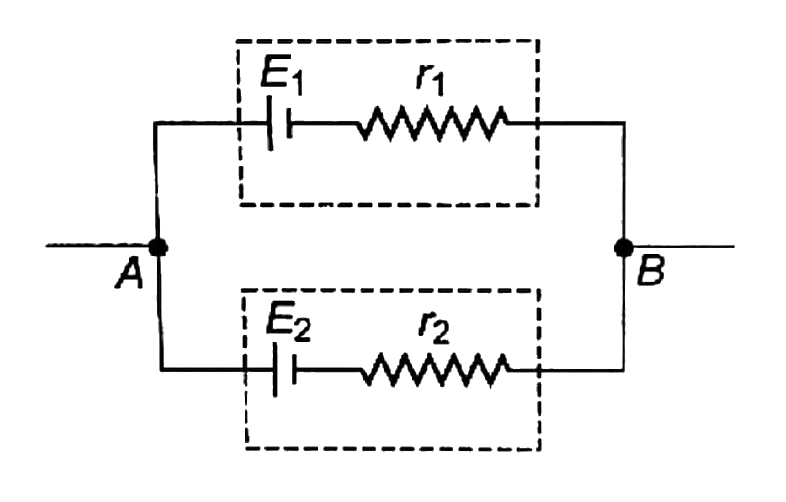
View SolutionTwo batteries of emf E1 and E2(E2>E1) and internal resistance r1 and r2 respectively are connected in parallel as shown in the figure. Then, which of the followings statements is correct ?

View SolutionA battery of EMF E produces currents 4 A and 3 A when connected to external resistance 1Ω and 2Ω respectively. The internal resistance of the battery is
View Solution
