The instantaneous angular position of a point on a rotating wheel is given by the equation
The torque on the wheel becomes zero at
a) t = 1 s
b) t = 0.5 s
c) t = 0.25 s
d) t = 2 s
t = 1 s
t = 0.5 s
t = 0.25 s
t = 2 s
The correct Answer is:a
To find the time at which the torque on the wheel becomes zero, we start with the given equation for the instantaneous angular position:
θ(t)=2t3−6t2
Step 1: Find Angular Velocity
First, we need to find the angular velocity ω, which is the first derivative of θ(t) with respect to time t:
ω(t)=dθdt=ddt(2t3−6t2)
Calculating the derivative:
ω(t)=6t2−12t
Step 2: Find Angular Acceleration
Next, we find the angular acceleration α, which is the derivative of angular velocity ω(t):
α(t)=dωdt=ddt(6t2−12t)
Calculating the derivative:
α(t)=12t−12
Step 3: Set Angular Acceleration to Zero
The torque on the wheel becomes zero when the angular acceleration α is zero. Therefore, we set α(t) to zero:
12t−12=0
Step 4: Solve for t
Now, we solve for t:
12t=12t=1 s
Conclusion
Thus, the torque on the wheel becomes zero at:
1 s
The correct answer is option (a) t=1 s.
First, we need to find the angular velocity ω, which is the first derivative of θ(t) with respect to time t:
Next, we find the angular acceleration α, which is the derivative of angular velocity ω(t):
The torque on the wheel becomes zero when the angular acceleration α is zero. Therefore, we set α(t) to zero:
Now, we solve for t:
Thus, the torque on the wheel becomes zero at:
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - ANGULAR MOMENTUM IN CASE OF ROTATIONS ABOUT A FIXED AXIS
16 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - ROLLING MOTION
16 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - KENEMATICS OF ROTATIONAL MOTION ABOUT A FIXED AXIS
3 Videos
- PRACTICE PAPERSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPERSEXERCISE - All Questions
150 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
Similar Questions
The angular position of a point over a rotating flywheel is changing according to the relation, θ=(2t3−3t2−4t−5) radian. The angular acceleration of the flywheel at time, t = 1 s is
View SolutionA angular positio of a point on the rim of a rotating wheel is given by θ=4t−3t2+t3 where θ is in radiuans and t is in seconds. What are the angualr velocities at
(a).t=2.0 and
(b). t=4.0s
(c). What is the average angular acceleration for the time interval that begins at t=2.0s and ends at t=4.0s?
(d). What are the instantaneous angular acceleration at the biginning and the end of this time interval?
View Solution
Knowledge Check
A current in a wire is given by the equation, . I=2t2−3t+1, the charge through cross section of : wire in time interval t=3s to t=5s is
A32.33CB43.34CC45.5CD42C
The position of a particle is given by the equation f(t)=t3−6t2+9t where t is measured in second and s in meter. Find the acceleration at time t. What is the acceleration at 4 s?
View SolutionMotion of particle is given by equation
s=(5t3+3t2+8t+17)m
The value of acceleration of the particle at t = 2 s is
View SolutionThe angular position of a line of a disc of radius r = 6cm is given by θ=10−5t+4t2 rad , the average angular velocity between 1 and 3s is
View SolutionThe angular position of a particle revolving about an axis is given by Ɵ(t) = t^2 - 3t + 4 radian. Find the acceleration of the point at time t = 2 s. Given radius of circular path is 1 m
View SolutionIn the s−t equations (s=10+20t−5t2) match the following
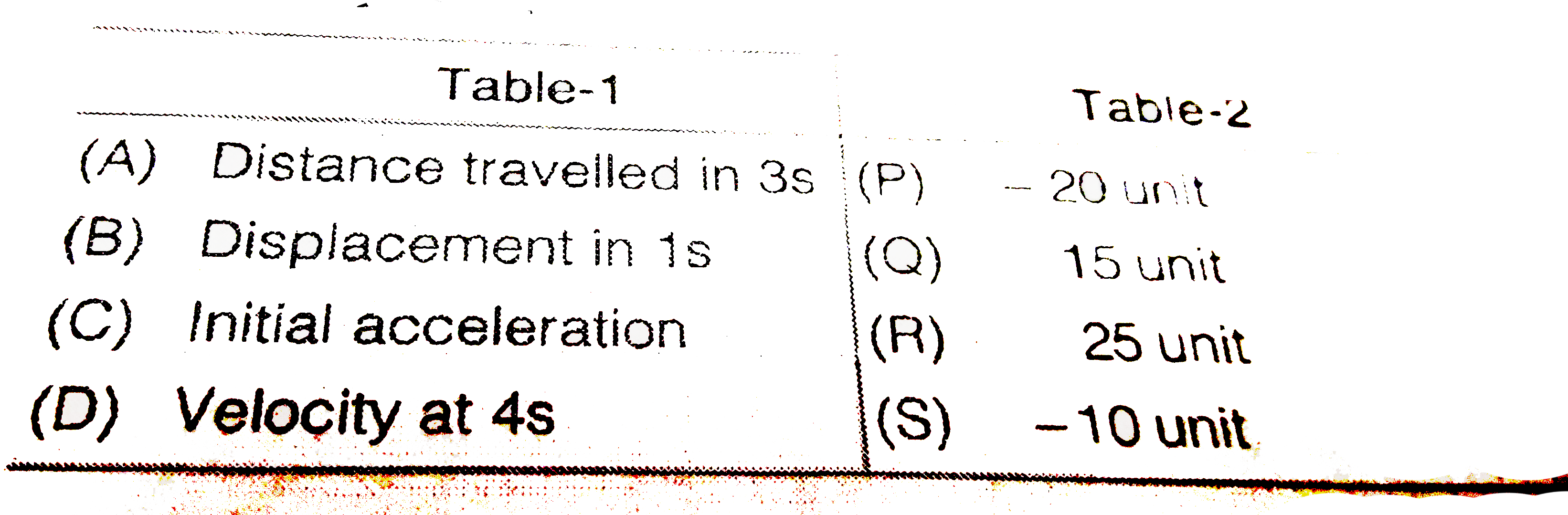
View SolutionThe displacement x of an object is given as a funstion of time, x=2t+3t2. The instantaneous velocity of the object at t = 2 s is
View SolutionThe velocity of an object is given by →v=(6t3ˆi+t2ˆj)m/s) . Find the acceleration at t = 2s.
View Solution
