The surface considered for Gauss's law is called
Closed surface
Sphereical surface
Gaussian surface
Plane surface
The correct Answer is:C
To solve the question regarding the surface considered for Gauss's law, we can follow these steps:
1. Understanding Gauss's Law: Gauss's law relates the electric field through a closed surface to the charge enclosed by that surface. It states that the electric flux through a closed surface is proportional to the charge enclosed within that surface.
2. Identifying the Surface: The surface used in Gauss's law must be a closed surface. This is essential because Gauss's law applies to the total charge enclosed by the surface.
3. Types of Surfaces: While there are various types of surfaces (like spherical, cylindrical, and planar), the specific term used in the context of Gauss's law is important.
4. Defining Gaussian Surface: The term "Gaussian surface" is specifically used to refer to any closed surface that we choose to apply Gauss's law. This can be a spherical surface around a point charge or a cylindrical surface around a line charge.
5. Conclusion: Based on the definitions and the context of Gauss's law, the surface considered for Gauss's law is called the "Gaussian surface."
Final Answer:
The surface considered for Gauss's law is called a Gaussian surface.
---
The surface considered for Gauss's law is called a Gaussian surface.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - ASSERTION & REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Electric Charges
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT Exemplar
6 Videos
Similar Questions
Knowledge Check
Assertion : Total flux through a closed surface is zero if no charge is enclosed by the surface.
Reason : Gauss law is true for any closed surface, no matter what its shape or size is.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
For a given surface the Gauss's law is stated as∮→E.d→A=0. From this we can conclude that
View SolutionThe surface of nerve fibres bear narrow areas called
View SolutionWhat is the nature of Gaussian surface involved in Gauss's law of electrostatics?
View SolutionAssertion : If a dipole is enclosed by a surface, then according to Gauss's law, electric flux linked with it will be zero.
Reason : The charge enclosed by a surface is zero.
View SolutionA : We may have a Gaussian surface in which less number of field lines enter and more field lines come out.
R : The electric field E in the Gauss's law is only due to the enclosed charges.
View SolutionAssertion : Gauss's law shows diversion when inverse square law is not obeyed.
Reason : Gauss's law is a consequence of conservation of charges.
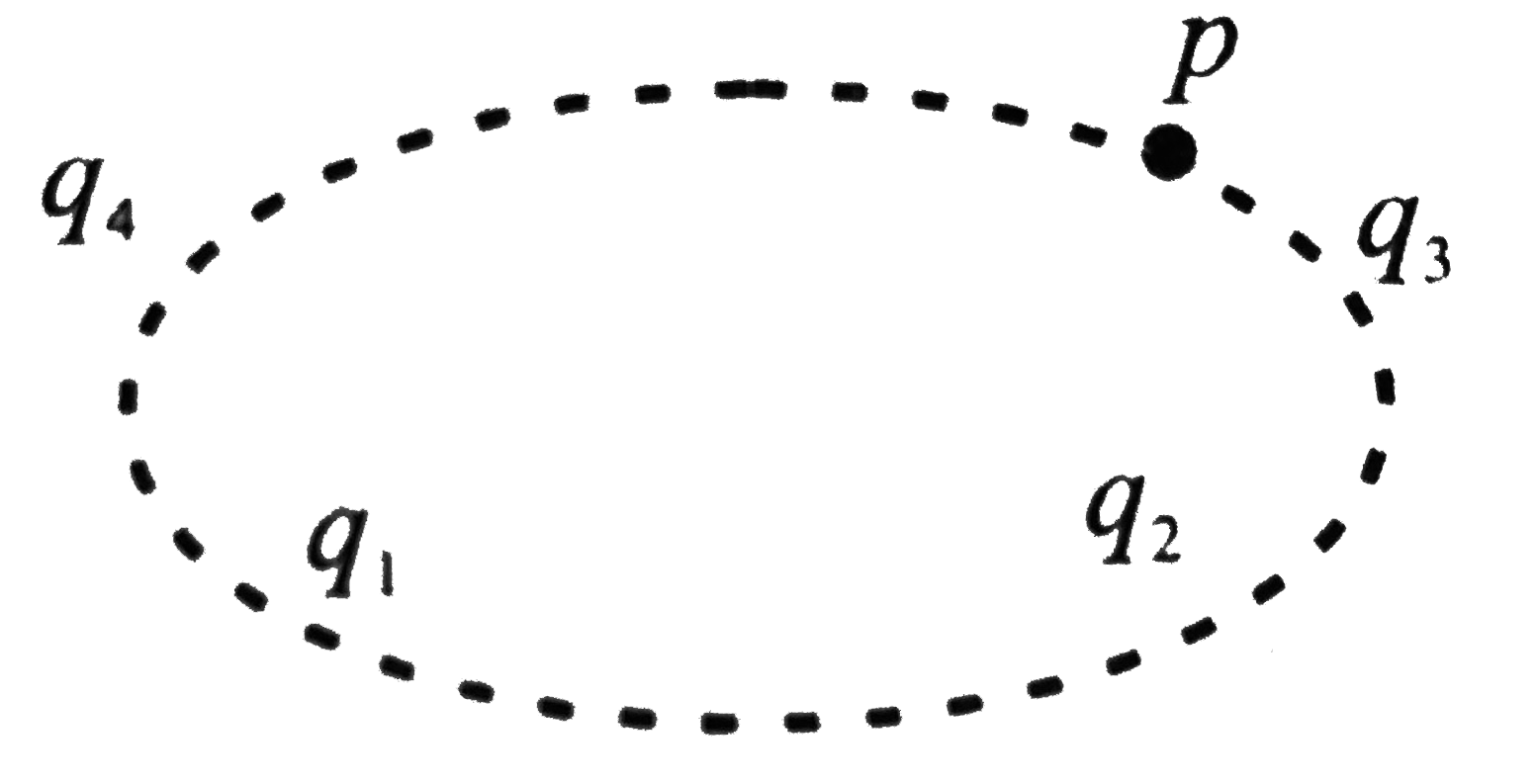
View SolutionGauss's law and Coulomb's law , although expressed in different forms , are equivalent ways of describing the relation between charge and electric field in static conditions . Gauss's law is ε0ϕ=qencl,when q(encl) is the net charge inside an imaginary closed surface called Gaussian surface. The two equations hold only when the net charge is in vaccum or air .
A Gaussian surface encloses two of the four positively charged particles. The particles that contribute to the electric field at a point P on the surface are
View Solution
