The magnetic force →F on a current carrying conductor of length I in an external magnetic field →B is given by
I×→B→l
→l×→BI
I(→l×→B)
l2→l×→B
The correct Answer is:C
To derive the expression for the magnetic force →F on a current-carrying conductor of length L in an external magnetic field →B, we can follow these steps:
1. Understanding the Concept:
The magnetic force on a charged particle moving in a magnetic field is given by the Lorentz force law. For a single electron, the force can be expressed as:
→F=−e→v×→B
where e is the charge of the electron, →v is the velocity of the electron, and →B is the magnetic field.
Hint: Recall the Lorentz force law and its application to charged particles.
2. Considering a Conductor:
In a conductor carrying current I, there are many charge carriers (like electrons). The total force on the conductor can be derived from the force on individual charge carriers.
3. Calculating the Total Force:
Let N be the number of charge carriers per unit volume, A be the cross-sectional area of the conductor, and L be its length. The total number of charge carriers in the conductor is N⋅A⋅L.
4. Relating Current to Charge Movement:
The current I is related to the charge movement as follows:
I=N⋅A⋅e⋅vd
where vd is the drift velocity of the charge carriers.
5. Expressing the Force on the Conductor:
The total magnetic force on the conductor can be expressed as:
→F=N⋅A⋅e⋅→vd×→B
6. Substituting for Current:
From the previous equation, we can substitute N⋅A⋅e⋅vd with I:
→F=I⋅→L×→B
where →L is a vector of length L in the direction of the current.
7. Final Expression:
Thus, the magnetic force on a current-carrying conductor in a magnetic field is given by:
→F=I⋅→L×→B
Conclusion:
The expression for the magnetic force →F on a current-carrying conductor of length L in an external magnetic field →B is:
→F=I⋅→L×→B
The magnetic force on a charged particle moving in a magnetic field is given by the Lorentz force law. For a single electron, the force can be expressed as:
→F=−e→v×→B
where e is the charge of the electron, →v is the velocity of the electron, and →B is the magnetic field.
In a conductor carrying current I, there are many charge carriers (like electrons). The total force on the conductor can be derived from the force on individual charge carriers.
Let N be the number of charge carriers per unit volume, A be the cross-sectional area of the conductor, and L be its length. The total number of charge carriers in the conductor is N⋅A⋅L.
The current I is related to the charge movement as follows:
I=N⋅A⋅e⋅vd
where vd is the drift velocity of the charge carriers.
The total magnetic force on the conductor can be expressed as:
→F=N⋅A⋅e⋅→vd×→B
From the previous equation, we can substitute N⋅A⋅e⋅vd with I:
→F=I⋅→L×→B
where →L is a vector of length L in the direction of the current.
Thus, the magnetic force on a current-carrying conductor in a magnetic field is given by:
→F=I⋅→L×→B
The expression for the magnetic force →F on a current-carrying conductor of length L in an external magnetic field →B is:
→F=I⋅→L×→B
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - HOTS5videos
MOVING CHARGES AND MAGNETISM
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - EXEMPLAR PROBLEMS6videos
MOVING CHARGES AND MAGNETISM
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar5videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason15videos
NUCLEI
Similar Questions
The magnetic field in a straight current carrying conductor wire is:
View SolutionState the Lorenz's force and express it in vector form. Which pair of vectors are always perpendicular to each other? Derive the expression for the force acting on a current carrying conductor of length L in a uniform magnetic field 'B'.
View SolutionThe direction of force on a current carrying conductor in a magnetic field is given by
View SolutionWhen is the force experienced by a current-carrying conductor placed in a magnetic field is largest?
View SolutionThe magnetic field at the centre of the current carrying coil is
View SolutionFind magnetic field at O due to the current carrying conductor as shown in the figure.
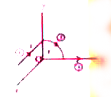 View Solution
View SolutionDraw the pattern of magnetic field lines around a current carrying strainght conductor. How does the strength of the magnetic field produced change.
(i) with the distance from the conductor ?
(ii) with increase in current in a conductor ?View SolutionMagnetic lines of force inside a current carrying solenoid are
View SolutionWrite an expression in a vector form for the Lorentz magnetic force →F on a charge Q moving with velocity →V in a magnetic field →B. What is the direction of the magnetic force?
View SolutionA student while studying the force experienced by a current carrying conductor in a magnetic field records the following observations:
(i) The force experienced by the conductor increases as the current is increased.
(ii) The force experienced by the conductor decreases as the strength of the magnetic field is increased.
Which of the two observations is correct and why?View Solution

