There is a uniform magnetic field directed perpendicular and into the plane of the paper. An irregular shaped conducting loop is slowly changing into a circular loop in the plane of the paper. Then
current is induced in the loop in the anti-clockwise direction
currnet is induced in the loop in the clockwise direction.
ac is induced in the loop.
no current is induced in the loop.
The correct Answer is:A
To solve the problem, we will analyze the situation step by step:
Step 1: Understand the Initial Conditions
We have a uniform magnetic field directed perpendicular and into the plane of the paper. This means that the magnetic field lines are going into the paper.
Hint: Identify the direction of the magnetic field and how it relates to the area of the loop.
Step 2: Analyze the Change in the Loop
An irregularly shaped conducting loop is slowly changing into a circular loop. As the shape of the loop changes, the area of the loop is also changing.
Hint: Consider how the area of the loop affects the magnetic flux through the loop.
Step 3: Determine the Change in Magnetic Flux
The magnetic flux (Φ) through the loop is given by the equation:
Φ=B⋅A
where B is the magnetic field strength and A is the area of the loop. As the area increases while changing from an irregular shape to a circular shape, the magnetic flux through the loop also increases.
Hint: Remember that an increase in area leads to an increase in flux if the magnetic field is constant.
Step 4: Apply Lenz's Law
According to Lenz's Law, the induced electromotive force (EMF) will act in such a way as to oppose the change in magnetic flux. Since the flux is increasing, the induced EMF will create a current that opposes this increase.
Hint: Think about the direction of the induced current that would oppose the increase in flux.
Step 5: Determine the Direction of Induced Current
To oppose the increase in magnetic flux (which is directed into the paper), the induced current must create a magnetic field that is directed out of the paper. Using the right-hand rule, if the induced current is to create a magnetic field out of the paper, it must flow in an anticlockwise direction around the loop.
Hint: Use the right-hand rule to visualize the relationship between current direction and magnetic field direction.
Conclusion
Thus, the current induced in the loop will be in the anticlockwise direction.
Final Answer: The correct option is that current is induced in the loop in the anticlockwise direction.
---
We have a uniform magnetic field directed perpendicular and into the plane of the paper. This means that the magnetic field lines are going into the paper.
An irregularly shaped conducting loop is slowly changing into a circular loop. As the shape of the loop changes, the area of the loop is also changing.
The magnetic flux (Φ) through the loop is given by the equation:
Φ=B⋅A
where B is the magnetic field strength and A is the area of the loop. As the area increases while changing from an irregular shape to a circular shape, the magnetic flux through the loop also increases.
According to Lenz's Law, the induced electromotive force (EMF) will act in such a way as to oppose the change in magnetic flux. Since the flux is increasing, the induced EMF will create a current that opposes this increase.
To oppose the increase in magnetic flux (which is directed into the paper), the induced current must create a magnetic field that is directed out of the paper. Using the right-hand rule, if the induced current is to create a magnetic field out of the paper, it must flow in an anticlockwise direction around the loop.
Thus, the current induced in the loop will be in the anticlockwise direction.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXEMPLAR PROBLEMS3videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION AND REASON15videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assertion And Reason15videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason15videos
ELECTROMAGNETIC WAVES
Similar Questions
Space is divided by the line AD into two regions. Region I is field free and the Region II has a unifrom magnetic field B direction into the plane of the paper. ACD is a simicircular conducting loop of radius r with center at O, hte plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity ω about an axis passing through O and the perpendicular to the plane of the paper. The effective resistance of the loop is R.
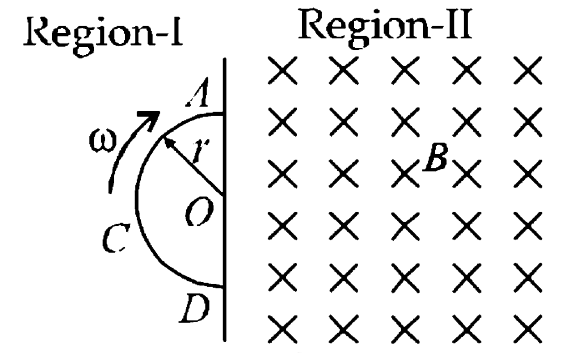
(i) obtain an expression for hte magnitude of the induced cureent in the loop.
(ii) Show the direction of the current when the loop is entering into the Rigion II.
Plot a graph between the induced e.m.f and the time of roation for two periods or rotation.View SolutionSpace is divided by the line AD into two regions. Region I is field free and the Region II has a unifrom magnetic field B direction into the plane of the paper. ACD is a simicircular conducting loop of radius r with center at O, hte plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity ω about an axis passing through O and the perpendicular to the plane of the paper. The effective resistance of the loop is R.

(i) obtain an expression for hte magnitude of the induced cureent in the loop.
(ii) Show the direction of the current when the loop is entering into the Rigion II.
Plot a graph between the induced e.m.f and the time of roation for two periods or rotation.View SolutionThe counductor AD moves to the right in a uniform magnetic field directed into the plane of the paper.
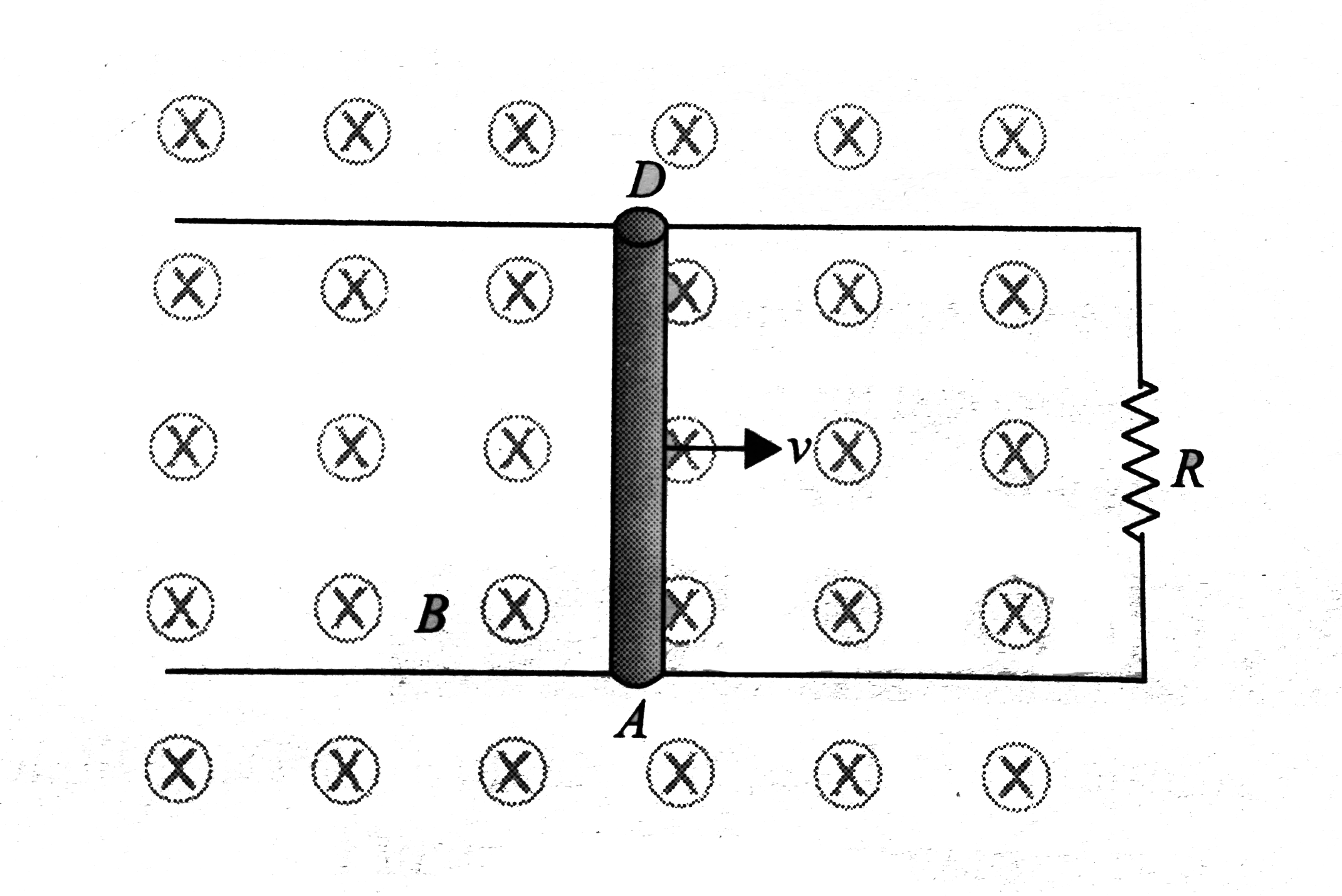 View Solution
View SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=a/4
(i) magnetic flux through the loop,
(ii) induced current in the loop and
(iii) external force required to maintain constant velocity of the loop, will beView SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

External force required to maintain constant velocity of the loop for x=−95a will beView SolutionPQRS is a square region of side 2a in the plane of paper. A uniform magnetic field B, directed perpendicular to the plane of paper and into its plane is confined within this square region. A square loop of side 'a' and made of a conducting wire of resistance R is moved at a constant velocity →v from left to right in the plane of paper as shown. Obviously, the square loop will enter the magnetic field at some time and then leave it after some time. During the motion of loop, whenever magnetic flux through it changes, emf will be induced resulting in induced current. Let the motion of the square loop be along x-axis and let us measure x coordinate of the centre of square loop from the centre of the square magnetic field region (taken as origin). Thus, x coordinate will be positive if the centre of square loop is to the right of the origin O (centre of magnetic field) and negative if centre is to the left.

For x=−9a/5, magnitude of induced current and its direction as seen from above will be:View Solutionthe uniform magnetic field perpendicular to the plane of a conducting ring of radius a change at the rate of α, then
View SolutionThere is a magnetic field acting in a plane perpendicular to this sheet of paper, downward into the paper as shown in the figure. Paticles in acuum move in the plane of the paper from left to right. The path indicated by the arrow could be travelled by
 View Solution
View SolutionThe magnetic field B shown in is directed into the plane of the paper. ACDA is a semicircular conducting loop of radius r with the centre at O. The loop is now made ot rotate clockwise with a constant angular velocity ω about on axis passing through O and perpendicular to the plane of the paper. The resistance of the loop is R. Obtain an expression for the magnitude of the induced current in the loop. Plot a graph between the induced current i and ωt, for two periods of rotation.
 View Solution
View SolutionA conducting loop of radius R is present in a uniform magnetic field B perpendicular to the plane of the ring. If radius R varies as a function of time t, as R=R0+t. The emf induced in the loop is
 View Solution
View Solution

