A 2 m long metallic rod rotates with an angular frequency 200 rod s−1 about on axis normal to the rod passing through its one end. The other end of the rod is in contact with a circular metallic ring. A constant megnetic field of 0.5 T parallel to axis exises everywhere. The emf developed between the centre and the ring is
100V
200V
300V
400V
The correct Answer is:B
To solve the problem of finding the EMF developed between the center and the ring of a rotating metallic rod, we can follow these steps:
Step 1: Identify the given values
- Length of the rod (L) = 2 m
- Angular frequency (ω) = 200 rad/s
- Magnetic field strength (B) = 0.5 T
Step 2: Determine the linear velocity at the end of the rod
The linear velocity (v) of the end of the rod can be calculated using the formula:
v=L⋅ω
Substituting the values:
v=2m⋅200rad/s=400m/s
Step 3: Calculate the average linear velocity
Since we need the average linear velocity between the center and the end of the rod, we can use:
vavg=vend+vcenter2
Here, the linear velocity at the center is 0, so:
vavg=400m/s+02=200m/s
Step 4: Use the formula for EMF
The EMF (E) induced in the rod can be calculated using the formula:
E=B⋅L⋅vavg
Substituting the values:
E=0.5T⋅2m⋅200m/s
Step 5: Calculate the EMF
Now, calculating the EMF:
E=0.5⋅2⋅200=200V
Final Answer
The EMF developed between the center and the ring is 200 V.
---
- Length of the rod (L) = 2 m
- Angular frequency (ω) = 200 rad/s
- Magnetic field strength (B) = 0.5 T
The linear velocity (v) of the end of the rod can be calculated using the formula:
v=L⋅ω
Substituting the values:
v=2m⋅200rad/s=400m/s
Since we need the average linear velocity between the center and the end of the rod, we can use:
vavg=vend+vcenter2
Here, the linear velocity at the center is 0, so:
vavg=400m/s+02=200m/s
The EMF (E) induced in the rod can be calculated using the formula:
E=B⋅L⋅vavg
Substituting the values:
E=0.5T⋅2m⋅200m/s
Now, calculating the EMF:
E=0.5⋅2⋅200=200V
The EMF developed between the center and the ring is 200 V.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXEMPLAR PROBLEMS3videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION AND REASON15videos
ELECTROMAGNETIC INDUCTION
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assertion And Reason15videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason15videos
ELECTROMAGNETIC WAVES
Similar Questions
A 1m long calculating rod rotates with an angular frequency of 400 rad s−1 an axis normal to the rod passing through its one one end. The other end of the rod is contact with a circular metallic ring. A constant magnetic field of 0.5 T parallel to the axis everywhere. Calculate the e.m.f. developed between the centre and the ring.
View SolutionA metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with on end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular at to the plane of the ring. A constant uniform magnetic field of 1 T parallel to the axis is persent eveywhere. what is the e.m.f. between the centre and the metallic ring?
View Solution
Knowledge Check
- Question 1 - Select One
A metallic rod of 1 m length is rotated with a frequency of 50 rev/s, with on end hinged at the centre and the other end at the circumference of a circular metallic ring of radius 1 m, about an axis passing through the centre and perpendicular at to the plane of the ring. A constant uniform magnetic field of 1 T parallel to the axis is persent eveywhere. what is the e.m.f. between the centre and the metallic ring?
A157VB117VC127VD137V
A metallic rod of 'L' length is rotated with angular frequency of 'w' with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius L, about an axis passing through the centre and perpendicular to the plane of the ring. A constant and uniform magnetic field B parallel to the axis is present everywhere. Deduce the expression for the emf between the centre and the metallic ring.
View SolutionA metallic rod of length 'I' is rotated with a frequency v with one end hinged at the centre and the other end at the circumference of a circualr metallic ring of a radius r, about an axis passing through the centre and perpendicular to the plane of the ring. A constant uniform magnetic field B parallel to the axis is present every where. Using Lorentz force, explain how emf is induced between the centre and the metallic ring and hence obtain the expression for it.
View SolutionA ring rotates with angular velocity ω about an axis perpendicula to the plane of the ring passing through the center of the ring (Fig. 3.77). A constant magnetic field B exists parallel to the axis. Find the emf induced in the ring.
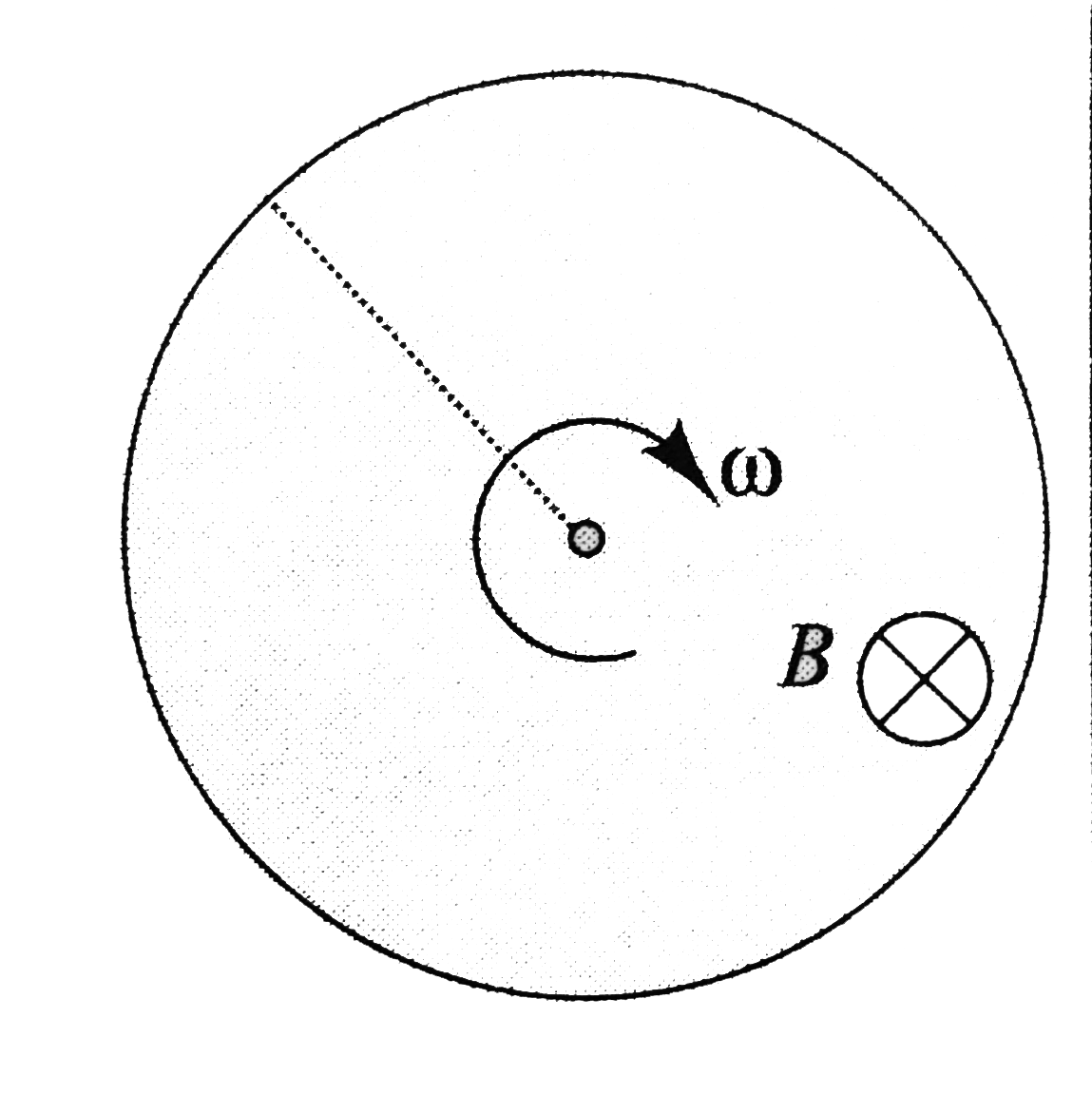 View Solution
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 View Solution
View SolutionA conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 View Solution
View SolutionA ring rotates with angular velocity ω about an axis perpendicular to the plane of the ring passing through the center of the ring.A constant magnetic field B exists parallel to the axis.Find the emf induced in the ring.
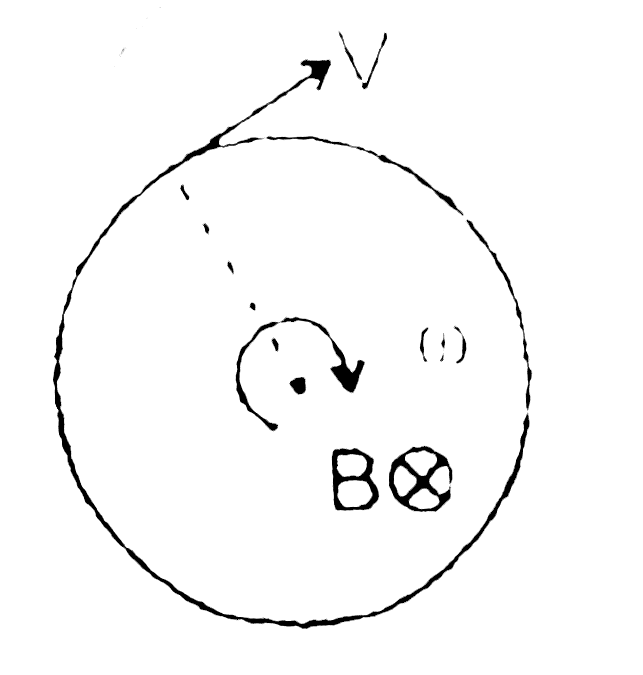 View Solution
View Solution

