The fission properties of .23994Pu are very similar to those of .23592 U. The average energy released per fission is 180 MeV. If all the atoms in 1 kg of pure .23994Pu undergo fission, then the total energy released in MeV is
4.53×1026 MeV
2.21×1014 MeV
1×1013 MeV
6.33×1024 MeV
The correct Answer is:a
To solve the problem, we need to calculate the total energy released when all the atoms in 1 kg of pure Plutonium-239 undergo fission. Here's a step-by-step breakdown of the solution:
Step 1: Determine the number of moles of Plutonium-239 in 1 kg
The molar mass of Plutonium-239 (Pu) is approximately 239 g/mol. To find the number of moles in 1 kg (1000 g) of Pu, we use the formula:
Number of moles=Total mass (g)Molar mass (g/mol)
Substituting the values:
Number of moles=1000 g239 g/mol≈4.18 moles
Step 2: Calculate the number of atoms (molecules) of Plutonium-239
To find the total number of atoms, we multiply the number of moles by Avogadro's number (6.022×1023 atoms/mol):
Number of atoms=Number of moles×Avogadro's number
Substituting the values:
Number of atoms=4.18 moles×6.022×1023 atoms/mol≈2.52×1024 atoms
Step 3: Calculate the total energy released
Given that the average energy released per fission is 180 MeV, the total energy released when all the atoms undergo fission is:
Total energy released (MeV)=Number of atoms×Energy per fission (MeV)
Substituting the values:
Total energy released (MeV)=2.52×1024 atoms×180 MeV
Calculating this gives:
Total energy released (MeV)=4.536×1026 MeV
Final Answer
The total energy released when all the atoms in 1 kg of pure Plutonium-239 undergo fission is approximately 4.54×1026 MeV.
---
The molar mass of Plutonium-239 (Pu) is approximately 239 g/mol. To find the number of moles in 1 kg (1000 g) of Pu, we use the formula:
To find the total number of atoms, we multiply the number of moles by Avogadro's number (6.022×1023 atoms/mol):
Given that the average energy released per fission is 180 MeV, the total energy released when all the atoms undergo fission is:
The total energy released when all the atoms in 1 kg of pure Plutonium-239 undergo fission is approximately 4.54×1026 MeV.
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - HOTS7videos
NUCLEI
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - EXEMPLAR PROBLEMS7videos
NUCLEI
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason15videos
MOVING CHARGES AND MAGNETISM
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 350videos
PRACTICE PAPPER
Similar Questions
The fission properties of .84Pu239 are very similar to those of .92U235. The average energy released per fission is 180MeV. How much energy in MeV is released if all the atoms in 1kg of pure .94Pu239 undergo fission.
View SolutionIn the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
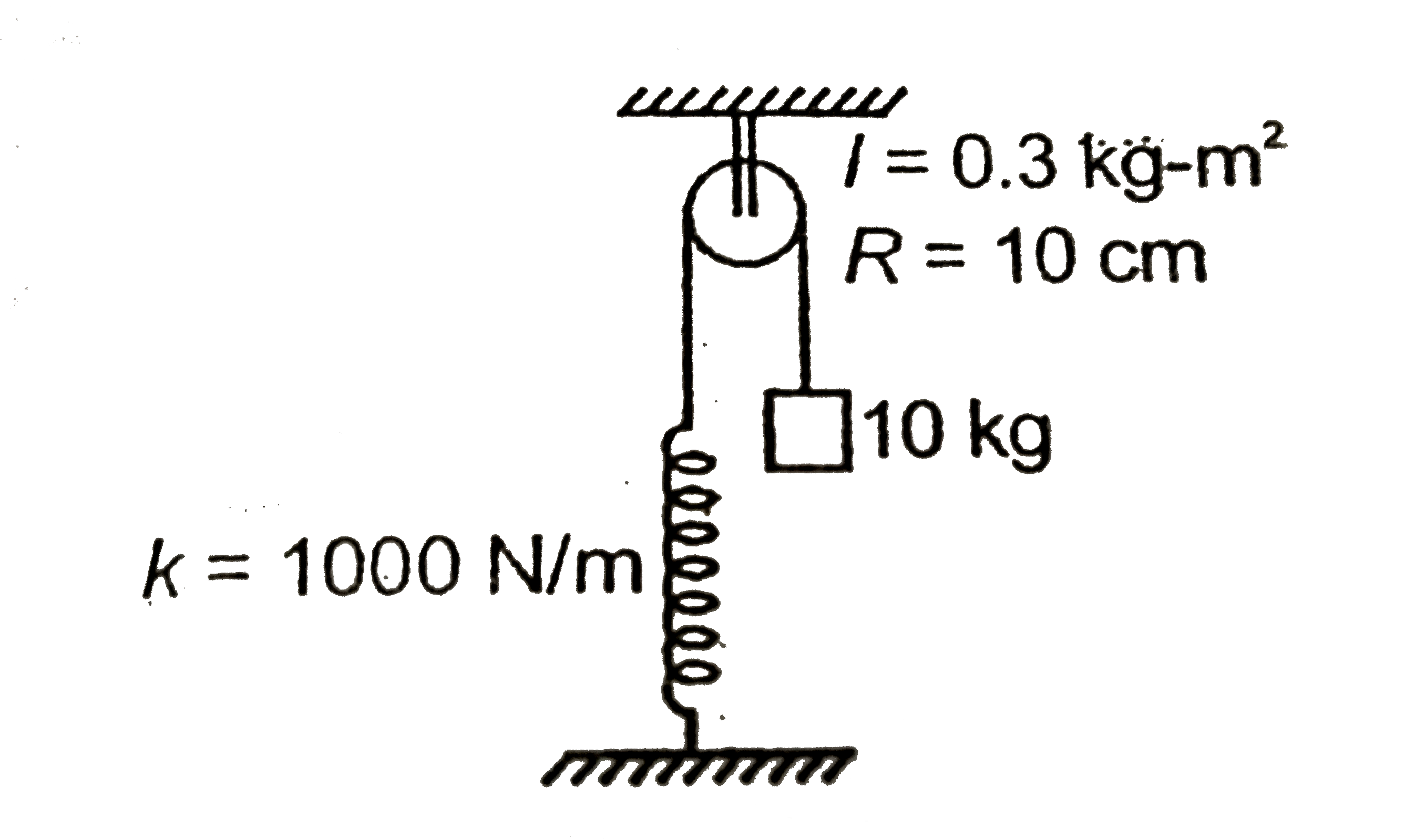 View Solution
View SolutionA nuclear reactor has a power of 16 kW. If the energy released per fission is 200 MeV, the number of fissions per second is
View SolutionCalculate the energy released by fission from 2 g of .23592U in k Wh. Given that the energy released per fission is 200 Mev.
View SolutionCalculate the energy released by fission from 2 gm of .92U235 in KWH. Given that the energy released per fission is 200 Mev.
View SolutionThe energy released by fission of one U235 atom is 200 MeV. Calculate the energy released in kWh, when one gram of uranium undergoes fission.
View Solution1.00 kg of .235U undergoes fission process. If energy released per event is 200MeV, then the total energy released is
View SolutionIf 200 MeV of energy is released in the fission of one nucleus of .92U235, The number of nuclei that must undergo fission to produce energy of 1000J in 1 sec is
View SolutionIn a nuclear reactor, the number of U235 nuclei undergoing fissions per second is 4×1020. If the energy releases per fission is 250 MeV, then the total energy released in 10 h is (1eV=1.6×10−19J)
View SolutionTo generate a power of 3.2 mega watt, the number of fissions of U235 per minute is.
(Energy released per fission =200MeV,1eV=1.6×10−19J).View Solution

