The decomposition of hydrocarbon follows the equation k=(4.5×1011s−1)e−28000K/T
Calculate Ea.
669 kJ mol−1
232. 79 kJ mol−1
4.5×1011kJmol−1
28000 kJ mol−1
The correct Answer is:B
To solve the problem of calculating the activation energy Ea from the given equation for the decomposition of hydrocarbon, we can follow these steps:
Step 1: Understand the given equation
The equation provided is:
k=(4.5×1011s−1)e−28000KT
This equation resembles the Arrhenius equation:
k=Ae−EaRT
where:
- k is the rate constant,
- A is the pre-exponential factor,
- Ea is the activation energy,
- R is the universal gas constant,
- T is the temperature in Kelvin.
Step 2: Compare the two equations
From the given equation, we can see that:
EaR=28000K
This means that:
Ea=28000K×R
Step 3: Substitute the value of R
The universal gas constant R is approximately 8.314J/mol⋅K. Now we can substitute this value into the equation:
Ea=28000K×8.314J/mol⋅K
Step 4: Calculate Ea
Now we perform the multiplication:
Ea=28000×8.314
Calculating this gives:
Ea=232792J/mol
Step 5: Convert to kilojoules per mole
To convert joules to kilojoules, we divide by 1000:
Ea=2327921000=232.792kJ/mol
Step 6: Round off the answer
Rounding off to two decimal places, we get:
Ea≈232.79kJ/mol
Final Answer
Thus, the activation energy Ea is:
232.79kJ/mol
---
The equation provided is:
k=(4.5×1011s−1)e−28000KT
This equation resembles the Arrhenius equation:
k=Ae−EaRT
where:
- k is the rate constant,
- A is the pre-exponential factor,
- Ea is the activation energy,
- R is the universal gas constant,
- T is the temperature in Kelvin.
From the given equation, we can see that:
EaR=28000K
This means that:
Ea=28000K×R
The universal gas constant R is approximately 8.314J/mol⋅K. Now we can substitute this value into the equation:
Ea=28000K×8.314J/mol⋅K
Now we perform the multiplication:
Ea=28000×8.314
Calculating this gives:
Ea=232792J/mol
To convert joules to kilojoules, we divide by 1000:
Ea=2327921000=232.792kJ/mol
Rounding off to two decimal places, we get:
Ea≈232.79kJ/mol
Thus, the activation energy Ea is:
232.79kJ/mol
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - Higher Order Thinking Skills9videos
CHEMICAL KINETICS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT EXEMPLAR PROBLEMS17videos
CHEMICAL KINETICS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - BIOMOLECULESEXERCISE - Assertion And Reason15videos
BIOMOLECULES
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - NCERT Exemplar15videos
CHEMISTRY IN EVERYDAY LIFE
Similar Questions
The decomposition of a hydrocarbon follows the equationk =(4.5×1011s−1)e−28000KT. Calculate Ea.
View SolutionThe decomposition of a hydrocarbon follows the equationk =(4.5×1011s−1)e−28000KT. Calculate Ea.
View SolutionThe decomposition of a hydrocarbon follows the equationk =(4.5×1011s−1)e−28000KT. Calculate Ea.
View SolutionThe rate constant for the first order decomposition of H2O2 is given by the following equation : log K = 14.2 - 1.0×104TK
Calculate E for this reaction and rate constant k if its half life period be 200 minutes. (Given : R = 8.314 JK−1mol−1)View SolutionThe rate constant for a second order reaction is given by k=(5×1011)e−29000k/T. The value of Ea will be:
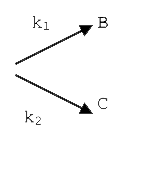
View SolutionA substance undergoes first order decomposition. The decomposition follows two parallel first order reactions as :
k1=1.26×10−4s−1,k2=3.8×10−5s−1
The percentage distribution of B and C are :
 View Solution
View SolutionThe decomposition of A into product has value of k as 4.5×103s−1 at 10∘C and energy of activation of 60kJmol−1. At what temperature would k be 1.5×104s−1?
View SolutionThe rate constant for the decomposition of hydrocarbons is 2.418×10−5s−1 at 546K. If the energy of activation is 179.9kJmol−1, what will be the value of pre−exponential factor?
View SolutionThe rate constant for the decompoistion of a certain reaction is described by the equation:
logk(s−1)=14−1.25×104KT
Pre-exponential factor for this reaction isView SolutionThe rate constant for the decompoistion of a certain reaction is described by the equation:
logk(s−1)=14−1.25×104KT
Energy of activation (in kcal) isView Solution

