A ray of light travelling in a rarer medium, strikes a plane boundary between the rarer medium and a denser medium at an angle of incident 'i' such that the reflected and the refracted rays are mutually perpendicular. Another ray of light of same frequency is incident on the same boundary from the side of denser medium. Find the minimum angle of incidence at the denser-rarer boundary so that the second ray is totally reflected.
θC=sin−1(coti)
Topper's Solved these Questions
- RAY OPTICSBOOK - AAKASH SERIESCHAPTER - RAY OPTICSEXERCISE - PROBLEMS ( LEVEL-I)
37 Videos
- PRACTICAL PHYSICSBOOK - AAKASH SERIESCHAPTER - PRACTICAL PHYSICSEXERCISE - LECTURE SHEET LEVEL-II (ADVANCED) Linked Comprehension Type Questions
6 Videos
- SEMICONDUCTOR DEVICESBOOK - AAKASH SERIESCHAPTER - SEMICONDUCTOR DEVICESEXERCISE - EXERCISE - II
31 Videos
Similar Questions
A ray of light from a denser medium strikes a rarer medium at an angle of incidence i. if the reflected and the refracted rays are mutually perpendicular to each other, what is the value of the critical angle ?
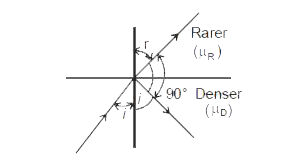
View SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidence i=tan−1(1/2). If the reflected and the refracted rays are mutually perpendicular to each-other, what is the value of the critical angle in degree?
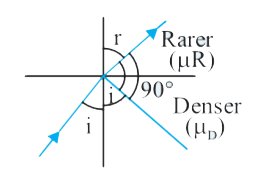
View Solution
Knowledge Check
Light from a denser medium 1 passes to a rarer medium 2. When the angle of incidence is θ the partially reflected and refracted rays are mutually perpendicular. The critical angle will be
 Asin−1(cotθ)Bsin−1(tanθ)Csin−1(cosθ)Dsin−1(secθ)
Asin−1(cotθ)Bsin−1(tanθ)Csin−1(cosθ)Dsin−1(secθ)A ray of light travelling obliquely from denser to rarer medium
Abends towards the normalBbends away from the normalCdoes deviate from its pathDnone of theseA ray of light from a denser medium strikes a rarer medium at an angle of incidence i. if the reflected and the refracted rays are mutually perpendicular to each other, what is the value of the critical angle ?
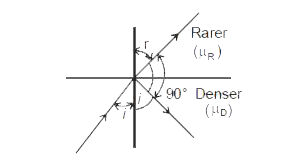 Asin−1(coti)Bcos−1(tani)Csin−1(tani)Dtan−1(sini)
Asin−1(coti)Bcos−1(tani)Csin−1(tani)Dtan−1(sini)
A ray of light from a denser medium strikes a rarer medium at an angle of incidence i=tan−1(1/2). If the reflected and the refracted rays are mutually perpendicular to each-other, what is the value of the critical angle in degree?

View SolutionLight from a denser medium 1 passes to a rarer medium 2. When the angle of incidence is θ the partially reflected and refracted rays are mutually perpendicular. The critical angle will be

View SolutionUnpolarised light passes from a rarer into a denser medium. If the reflected and the refracted rays are mutually perpendicular, the reflected light is linearly polarised ___________ to the plane of incidence.
View SolutionWhen a ray goes from an optically rarer medium to a denser medium, it:
View SolutionA ray of light from a rarer medium strikes a denser medium at angle of incidence 60°. The reflected and refracted rays are perpendicular to each other. The refractive index of denser medium and angle of deviation respectively are
View Solution
