A cannon and a target are 5.10 km apart and located at the same level. How soon will the shell launched with the initial velocity 240 m/s reach the target in the absence of air drag?
Hence v0=240ms−1,R=5.10ikm=5100m,,g=9.8ms−2,θ=?
From formula R=v20sin2θg
We have sin2θ=Rgv20, Putting values we get
sin2θ=5100×9.8240×240=0.8677=√32=sin60∘ or sin120∘⇒θ=30∘ or 60∘
From formula T=2v0sinθg
When θ=30∘,T1=2×240×0.59.8=24.5sec
When θ=60∘,T2=2×d240×0.8679.8=42.14 sec
Similar Questions
A cannon and a target are 5.10Km apart and located at the same level. How soon will the shell launched with the initial velocity 240m/s reach the target in the absence of air drag?
View SolutionA cannon a target are 5.0km apart on the same horizontal plane. How long will the shell launched with a velocity of 240ms−1 take to reach the tanget ? (g=10ms−2)
View Solution
Knowledge Check
- Question 1 - Select One
A cannon of mass 5m (including a shell of mass m) is at rest on a smooth horizontal ground, fires the shell with its barrel at an angle θ with the horizontal at a velocity u relative to cannon. Find the horizontal distance of the point where shell strikes the ground from the initial position of the cannon :-
A4u2sin2θ5gBu2sin2θ5gC3u2sin2θ5gD8u2sin2θ5g - Question 2 - Select One
A bullet of mass 10 g leaves a rifle at an initial velocit of 1000 m/s and strikes a target at the same level with a velocity of 100 m/s. The work done in joule to overcome the resistance of air will be
A5000B3750C500D375 - Question 3 - Select One
A bullet of mass 20 g leaves a riffle at an initial speed 100 m/s and strikes a target at the same level with speed 50 m/s. The amount of work done by the resistance of air will be
A100 JB25 JC75 JD50 J
A cannon and a target are 5.0 km apart on the same horizontal plane. How long will the shell, launched with a velocity of 240ms−1, take to reach the target ? (g=10ms−2)
View SolutionA cannon of mass 1000 kg located at the base of an inclined plane fires a shell of mass 100 kg in a horizontal direction with a velocity 180 km/hr. The angle of inclination of inclined plane with the horizontal is 45∘. The coefficient of friction between the cannon and inclined plane is 0.5. The height in meter to which the cannon ascends the inclined plane as a result of recoil is : (g=10m/s2):
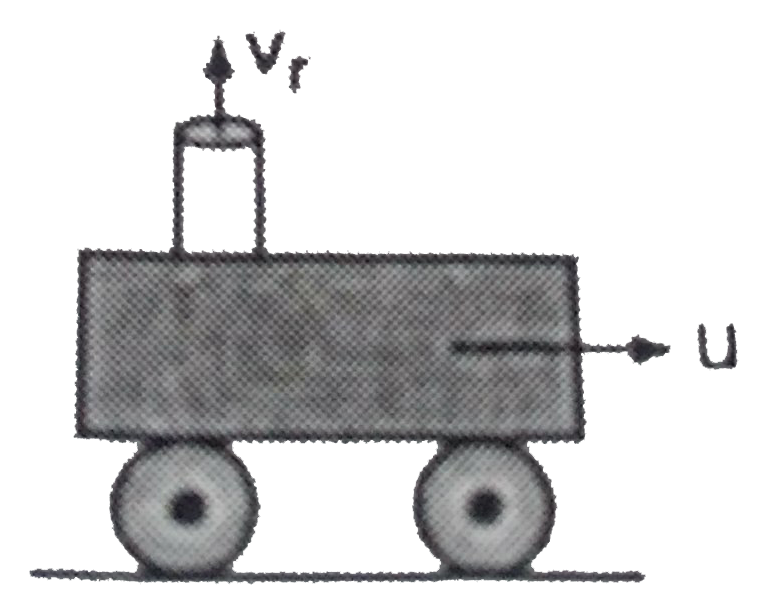
View SolutionA shell is fired vertically from a cannon which is travelling at constant speed u=30km/hr. the projectile leaves the connon with a velocity vr=20 m/s relative to the cannon . Show that the shell will land on the vehicle at the gun location and calcu late the distance by the vehicle during the flight of shell.
 View Solution
View SolutionA cannon of mass 5m (including a shell of mass m) is at rest on a smooth horizontal ground, fires the shell with its barrel at an angle θ with the horizontal at a velocity u relative to cannon. Find the horizontal distance of the point where shell strikes the ground from the initial position of the cannon :-
View SolutionA bullet of mass 20 g leaves a riffle at an initial speed 100 m/s and strikes a target at the same level with speed 50 m/s. The amount of work done by the resistance of air will be
View Solution
