A cannon of mass 1000 kg located at the base of an inclined plane fires a shell of mass 100 kg in a horizontal direction with a velocity 180 km/hr. The angle of inclination of inclined plane with the horizontal is 45∘. The coefficient of friction between the cannon and inclined plane is 0.5. The height in meter to which the cannon ascends the inclined plane as a result of recoil is : (g=10m/s2):
Momentum of cannon after recoil = Momentum of shell
=100×180×518
=5000 kg-m/s
Velocity of recoil of cannon =50001000=5 m/s
Let the cannon ascends a distance s on the rough inclined surface. The effective deceleration is
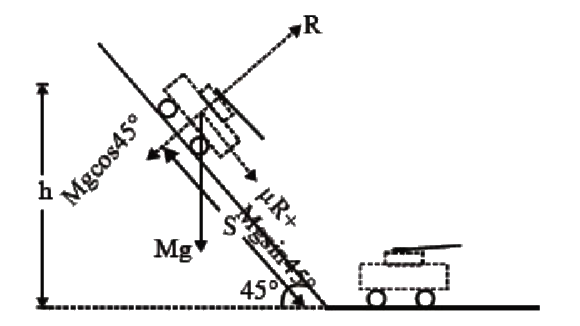
a=μR+Mgsin45∘M
a=μMgcos45∘+Mgsin45∘M
a=g√2[μ+1]
v2=u2−2as
0=(5)2−2×g√2(μ+1)s
or s=25√2×10(0.5+1)=25√2×15=53√2 m
Now, h=s.sin45∘=53√2×1√2=56m
Topper's Solved these Questions
Similar Questions
A cannon of mass 1000 kg located at the base of an inclined of an inclined plane fires a shell of mass 100 kg in a horizontal direction with a velocity 180 km//hr.The angle of inclination of inclined plane with the horizontal is 45∘. The coefficient of friction between the canon and inclined plane is 0.5. The height in meter to which the cannon ascends the inclined plane as a result of recoil is (g=10m/s2)
View SolutionA cannon of mass 1000kg located at the base of an inclined plane fires a shell of mass 50kg in horizontal direction with velocity 180km/h. The angle of inclination of the inclined plane with the horizontal is 45∘. The coefficient of friction between the cannon and inclined plane is 0.5. The maximum height, in metre, to which the cannon can ascend the inclined plane as a result of recoil is
View Solution
Knowledge Check
A cannon of mass 1000kg located at the base of an inclined plane fires a shell of mass 50kg in horizontal direction with velocity 180km/h. The angle of inclination of the inclined plane with the horizontal is 45∘. The coefficient of friction between the cannon and inclined plane is 0.5. The maximum height, in metre, to which the cannon can ascend the inclined plane as a result of recoil is
A56B524C512Dnone of theseA cannon of mass 2m located at the base of an inclined plane shoots a shell of mass m in horizontal direction with velocity v0 The angle of inclination of plane is 45∘ and the coefficient of friction between the cannon and the plane is 0.5 The height to which cannon ascends the plane as a result of recoil is :
Av202gBv2012gCv206gDv20gA block rests on a rough inclined plane making an angle of 30° with the horizontal. The coefficient of static friction between the block and inclined plane is 0.8. If the frictional force on the block is 10 N, the mass of the block is
A2 kgB4 kgC1.6 kgD2.5 kg
A cannon of mass M located at the base of an inclined plane, shoots a shell of mass m in a horizontal direction with velocity v. To what vertial, heigh does the cannon ascend the inclined plane as a result of recoil, if the angle of inclination of the plane if α and the coefficient of friction between the cannon and the plane is μ.
View SolutionA 20kg box is gently placed on a rough inclined plane of inclination 30∘ with horizontal. The coefficient of sliding friction between the box and the plane is 0.5.Find the acceleration of the box down the incline.
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View Solution
