A ball starts falling with zero initial velocity on a smooth inclined plane forming an angle α with the horizontal. Having fallen the distnace h, the ball rebounds elastically off the inclined plane. At what distance from the impact point will the ball rebound for the second time?
The situation is shown in fig
Let the ball rebound from point A and return to the plane at point B, moving along the trajectory shown in diagram.
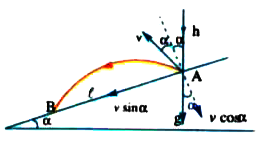
Let AB=l
Velocity of rebound at point A,v=√2gh
Velocity component perpendicular to plane =vcosα
velocity component along the plane =vsinα
Retardation perpendicular to plane =gcosα
Acceleration along the plane =gsinα
If the ball comes to rest at this highest point after time, t then (vcosα−gcosα.t)=0 or t=vg
Time of fight of the ball T=2t=2vg
Range of the ball along the plane
l=(vsinα)T+12(gsinα)T2
=vsinα.2vg+12.gsinα4v2g2
=2v2gsinα+2v2gsinα
=4v2gsinα=4(2gh)gsinα
(∴v=√2gh) ∴l=8hsinα
Similar Questions
A ball starts falling with zero initial velocity on to a smooth inclined plane forming an angle α with the horizontal. Having fallen a distance h, the ball rebounds elastically off the inclined plane. At what distance from the impact point will the ball rebound for the second time ?
View SolutionA ball starts falling with zero initial velocity on to a smooth inclined plane forming an angle α with the horizontal. Having fallen a distance h, the ball rebounds elastically off the inclined plane . At what distance from the impact point will the ball rebound for the second time ?
View Solution
Knowledge Check
- Question 1 - Select One
A ball starts falling freely from a height h from a point on the inclined plane forming an angle α with the horizontal as shown. After collision with the incline it rebounds elastically off the plane. Then it again strikes the incline at
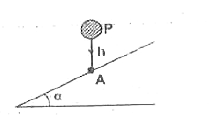 At=√8hg after it strikes the inclined at ABt=√2hgafter it strikes the inclined at A.Ca distance 4h sin α from A along the inclineDa distance 8h sin α from A along the incline
At=√8hg after it strikes the inclined at ABt=√2hgafter it strikes the inclined at A.Ca distance 4h sin α from A along the inclineDa distance 8h sin α from A along the incline - Question 2 - Select One
A ball is projected with velocity u at right angle to the slope which inclined at an angle α with the horizontal. The distance x along the inclined plane that it will travell before again striking the slope is-
A2u2gcosαB2u2gtanαC2u2gtanαcosαD2u2gtanαsinα
A ball starts falling freely from a height h from a point on the inclined plane forming an angle α with the horizontal as shown. After collision with the incline it rebounds elastically off the plane. Then it again strikes the incline at
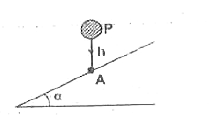 View Solution
View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View Solution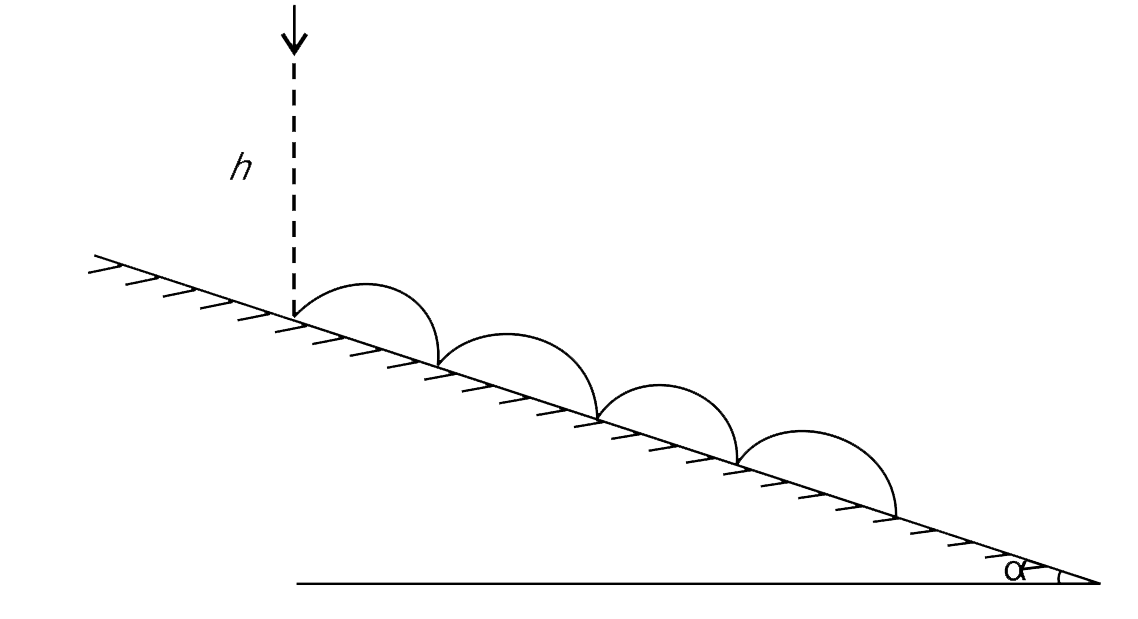
A ball is released in air above an incline plane inclined at an angle α to the horizontal. After falling vertically through a distance h it hits the incline and rebounds. The ball flies in air and then again makes an impact with the incline. This way the ball rebounds multiple times. Assume that collisions are elastic, i.e., the ball rebound without any loss in speed and in accordance to the law of reflection.
(a) Distance between the points on the incline where the ball makes first and second impact is l1 and distance between points where the ball makes second and third impact is l2. Which is large l1 or l2?
(b) Calculate the distance between the points on the incline where the ball makes second and fifth impact.View SolutionA ball is projected horizontally with a speed u from the top of inclined plane of inclination α with horizontal. At what distance along the plane, the ball will strike the plane ?
View SolutionA ball is projected with velocity u at right angle to the slope which inclined at an angle α with the horizontal. The distance x along the inclined plane that it will travell before again striking the slope is-
View Solution

