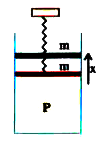
A massless piston which can slide inside a smooth vertical cylinder is attached with a light spring initially in its natural length as shown with force constant k. Now, a mass 'm' is placed on the piston. 'A' is cross-sectional area of the cylinder and the piston. Find the work done by the gas as it expands, if the piston moves up by a distance x (consider P0 as the atmospheric pressure).
As the piston is massless, net force on it at every instant is zero.
=upward force = downward force =PA=kx+mg+P0A
(or)P=P0+kxA+mgA,dW=PdV=P(Adx)=(AP0+kx+mg)dx
∴W=∫x0PdV(or)W=∫x0(AP0+kx+mg)dx=P0Ax+12kx2+mgx (as Ax=ΔV)
(or)W=P0ΔV+12kx2+mgx
The result can be stated in a different manner as under.
The gas does work against the atmospheric pressure P0 (which is constant), the spring force kx (which varies linearly with x) and the gravity force mg (which is again constant).
∴W1=Work done against P0=P0ΔV
W2=work done against kx=12kx2
and W3 = Work done against mg = mgx
So, that Wtotal=W1+W2+W3=P0ΔV+12kx2+mgx
Topper's Solved these Questions
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - TEST YOUR SELF
17 Videos
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - SHORT ANSWER TYPE QUESTIONS
8 Videos
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET ((ADVANCED) INTEGER/SUBJECTIVE TYPE QUESTIONS))
11 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) PRACTICE SHEET (ADVANCED) Integer/Subjective Type Questions
2 Videos
- THERMOMETRY BOOK - AAKASH SERIESCHAPTER - THERMOMETRY EXERCISE - Numerical Exercise (LEVEL- 1)
11 Videos
Similar Questions
Knowledge Check
A fixed container is fitted with a piston which is attached to a spring of spring constant k. The other and of the spring is attached to a rigid wall. Initially the spring is in its natural length and the length of container between the piston and its side wall is L. Now an dideal diatomic gas is slowly filled in the container so that the piston moves quasistatically. It pushed the piston by x so that the spring now is compressed by x. The total rotaional kinetic energy of the gas molecules in terms of the displacement x of the piston is (there is vacuum outside the container)
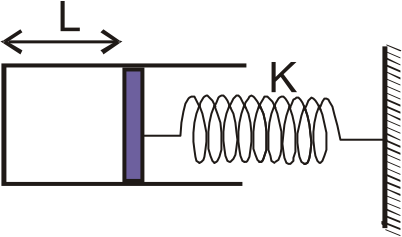 AkxLB4kxLCkx(x+L)D2kx2L
AkxLB4kxLCkx(x+L)D2kx2L
Method 3 of W
Mass of a piston shown in Fig. is m and area of cross-section is A. Initially spring is in its natural length. Find work done by the gas.

View SolutionMethod 3 of W
Mass of a piston shown in Fig. is m and area of cross-section is A. Initially spring is in its natural length. Find work done by the gas.

View SolutionA fixed container is fitted with a piston which is attached to a spring of spring constant k. The other and of the spring is attached to a rigid wall. Initially the spring is in its natural length and the length of container between the piston and its side wall is L. Now an dideal diatomic gas is slowly filled in the container so that the piston moves quasistatically. It pushed the piston by x so that the spring now is compressed by x. The total rotaional kinetic energy of the gas molecules in terms of the displacement x of the piston is (there is vacuum outside the container)

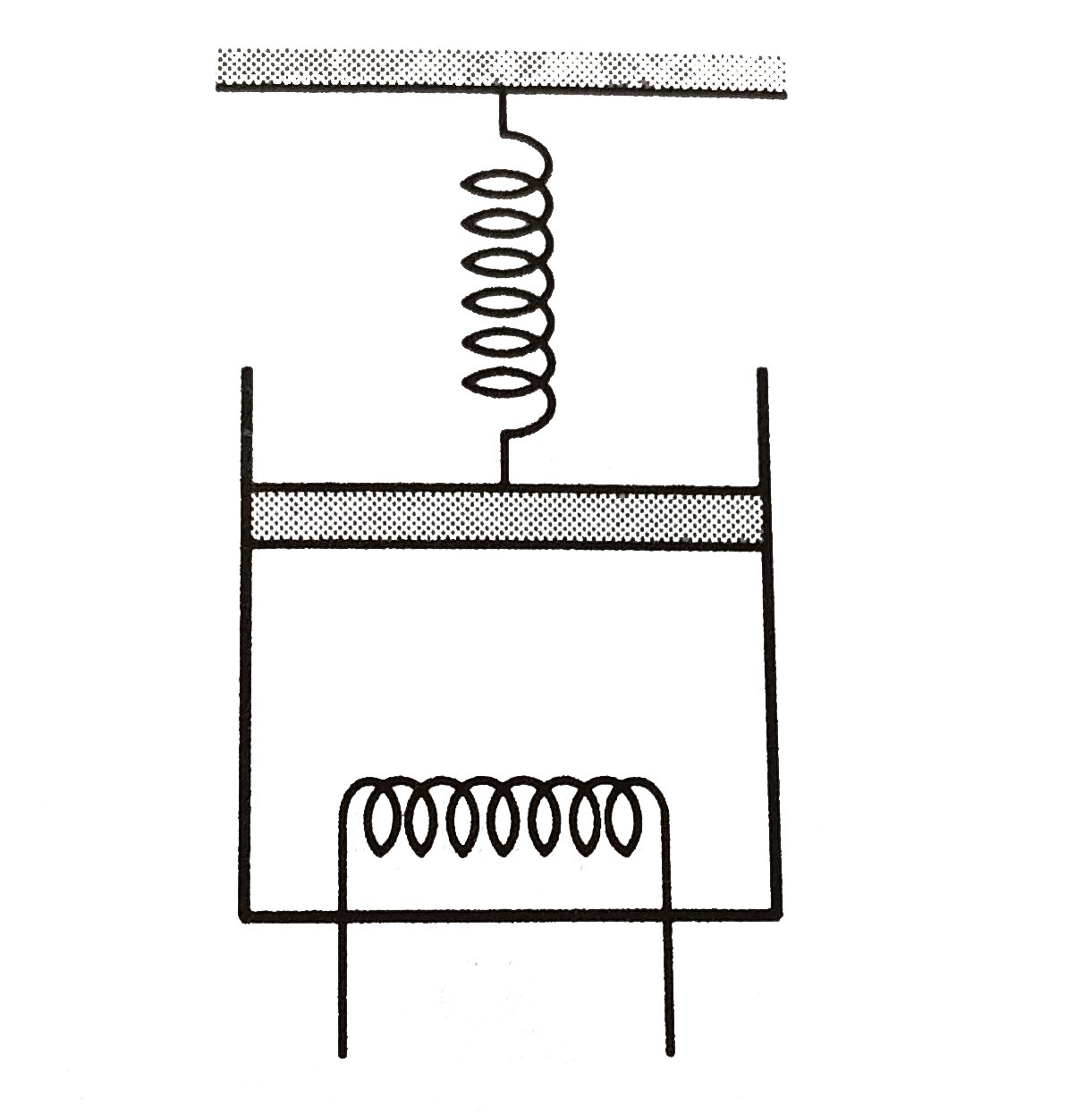
View Solution2.00 mole of a monatomic ideal gas (U=1.5nRT) is enclosed in an adiabatic, fixed , vertical cylinder fitted with a smooth, light adiabatic piston. The piston is connected to a vertical spring of spring constant 200Nm−1 as shown in .the area of cross section of the cylinder is 20.0cm2. initially, the spring is at its natural length and the temperature of the gas is 300K at its natural length. the atmosphere pressure is 100 kPa. the gas is heated slowly for some time by means of an electric heater so as to move the position up through 10 cm. find (a) the work done by the gas (b) the final temperature of the gas and (c ) the heat supplied by the heater.
View SolutionA gas filled in a cylinder fitted with a movable piston is compressed. What is the nature of work done by the applied force?
View SolutionA gas filled in a cylinder fitted with a movable piston is compressed. What is the nature of work done by the applied force?
View SolutionAn ideal gas at NTP is enclosed in an adiabatic vertical cylinder havin an area of cross section A=27cm2 between two light movable pistons as shown in figure. Spring with force constant K=37−−N/m is in a relaxed state initially. Now the lower position is moved upwards a distance h/2, h being the initial length of gas column . It is observed that the upper piston moves up by a distance h/16. Final temperature of gas4/3×273K. Take γ for the gas to be 3/2.

When the lower pistone is moved upwards a distance h/2, the compression.
View Solution
