If the vertices of a feasible region are O(0,0),A(10,0),B(0,20),C(15,15), then minimum value of a objective function Z=10x−20y+30 is….......Solution in Gujrati
−120
130
30
−370
The correct Answer is:D
Step by step video, text & image solution for If the vertices of a feasible region are O(0,0),A(10,0), B(0,20), C(15, 15), then minimum value of a objective function Z= 10 x - 20y + 30 is…....... by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - BOARD'S QUESTION PAPER MARCH - 2020EXERCISE - PART - B (Section - A)4videos
BOARD'S QUESTION PAPER MARCH - 2020
- BOOK - KUMAR PRAKASHANCHAPTER - BOARD'S QUESTION PAPER MARCH - 2020EXERCISE - PART - B (Section - B)7videos
BOARD'S QUESTION PAPER MARCH - 2020
- BOOK - KUMAR PRAKASHANCHAPTER - APPLICATION OF INTEGRALSEXERCISE - PRACTICE PAPER ( SECTION -D)2videos
APPLICATION OF INTEGRALS
- BOOK - KUMAR PRAKASHANCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Practice Paper - 5 (Section-D)4videos
CONTINUITY AND DIFFERENTIABILITY
Similar Questions
Find the values of each of the following :
tan12[sin−12x1+x2+cos−11−y21+y2],|x|<1,y>0andxy<103:14View SolutionConsider f,R+→[−5,∞) given by f(x)=9x2+6x−5. Show that f is invertible with f−1(y)=⎛⎜ ⎜⎝(√y+6)−13⎞⎟ ⎟⎠, where R+ is the set of all non-negative real numbers.
01:21View SolutionFind the area of the regaion enclosed by {(x,y):y2≤4x,4x2+4y2≤9}.
01:32View SolutionIf y=ecos−1x,−1≤x≤1, then prove that (1−x2)d2ydx2−xdydx−y=0
01:46View SolutionThe maximum value of objective function z=2x+3y in the given feasible region is
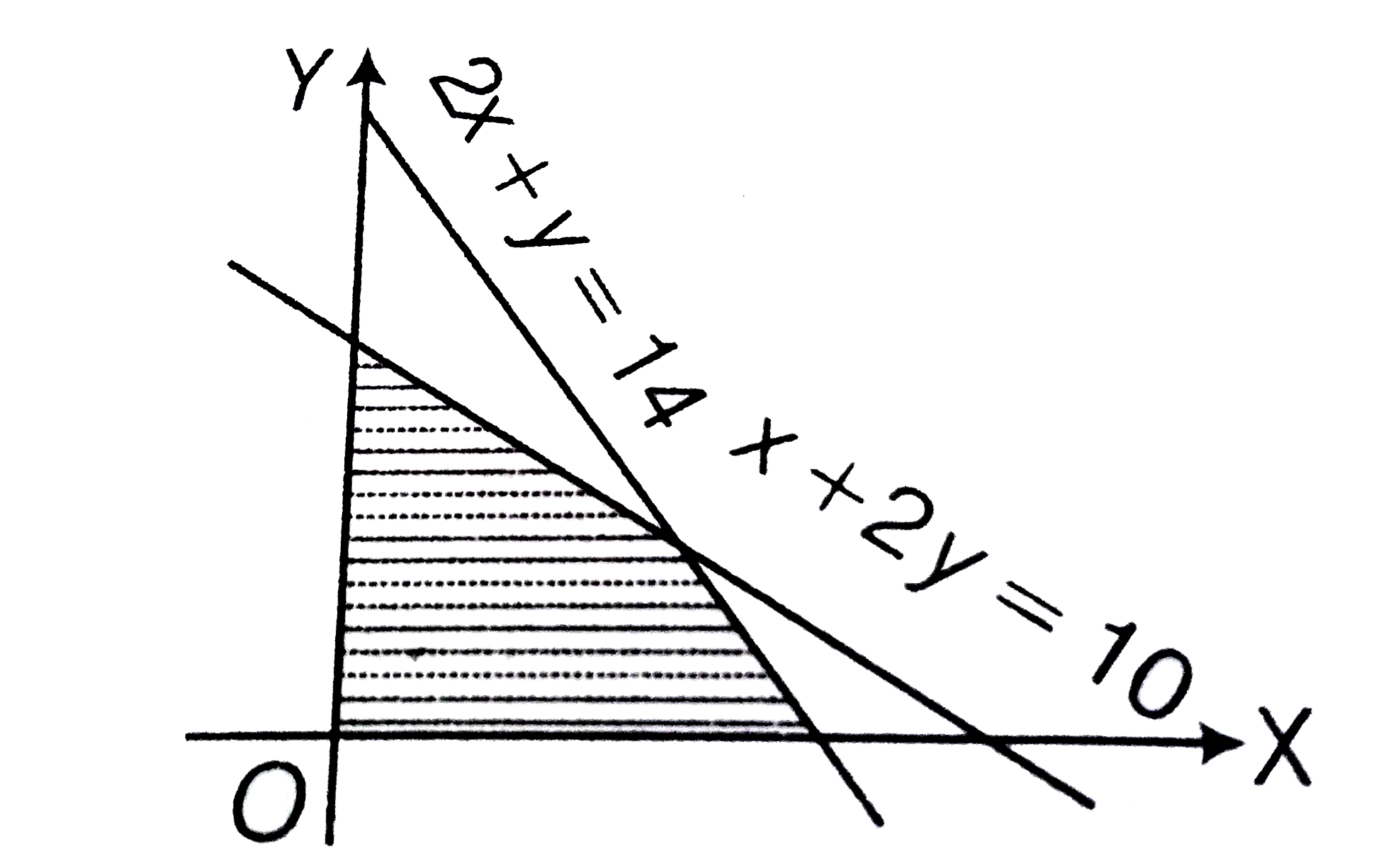 02:20View Solution
02:20View SolutionConsider the following statements
I. If the feasible region of an LPP is undbounded then maximum or minimum value of the obJective function Z = ax + by may or may not exist .
II. Maximum value of the objective function Z = ax + by in an LPP always occurs at only one corner point of the feasible region.
Ill. In an LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
IV. In an LPP, the maximum value of the objective function Z = ax + by is always finite.
Which of the following statements are true?02:51View SolutionThe value of objective function is maximum under linear constraints (a) At the centre of feasible region (b)At (0,0) (c)At any vertex of feasible region (d)The vertex which is maximum distance from (0,0)
02:14View SolutionThe feasible region for an LLP is shown in the Figure. Let z = 3x - 4y be the objective function. Minimum value of z is
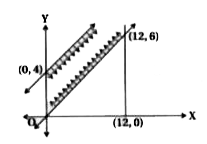 02:15View Solution
02:15View SolutionIn a LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
03:01View Solutionz = 30x - 30y + 1800 is a objective function. The corner points of the feasible region are (15, 0), (15, 15), (10, 20), (0, 20) and (0, 15). z has the minimum value at …….. point.
03:55View SolutionIf the vertices of a feasible region are O(0, 0), A(10, 0), B(0, 20), C(15, 15), then minimum value of a objective function Z = 10x - 20y + 30 is ………
02:05View SolutionThe feasible region for LPP is shown shaded in the figure . Find the minimum value of the objective function z = 11x + 7y.
02:44View SolutionThe minimum value of the expression |x−p+|x−15|+|x−p−15∣ for 'x' in the range p≤x≤15 where x<p<15 is 10 (b) 15 (c) 30 (d) 0
03:21View SolutionThe minimum value of the expression |x−p|+|x−15|+|x−p−15| for 'x' in the range p≤x≤15 where x<p<15 is 10 (b) 15 (c) 30 (d) 0
01:20View SolutionIf the feasible region for a linear programming problem is bounded, then the objective function Z = ax+by has both a maximum and a minimum value on R.
02:10View Solution

