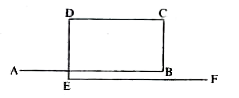
In the shown planar frame made of thin uniform rods, the length of sec

Topper's Solved these Questions

BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF LIQUIDS (LECTURE SHEET) Straight Objective Type Questions
15videos
THERMAL PROPERTIES OF MATTER
BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF LIQUIDS (LECTURE SHEET) More than One correct answer Type Questions
2videos
THERMAL PROPERTIES OF MATTER
BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF SOLIDS (PRACTICE SHEET) LEVEL-II (Matrix Matching Type Questions)
2videos
THERMAL PROPERTIES OF MATTER
BOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE
99videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
BOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - EXERCISE - 3
33videos
THERMODYNAMICS

Knowledge Check

Question 1 - Select One
In the shown planar frame made of thin uniform rods, the length of section $A B$ and $E F$ is $l_{1}$ and its thermal linear coefficient of expansion is $α_{1}$ . The length of section $C D$ is $l_{2}$ and its thermal linear coefficient of expansion is $α_{2}$ . $C B and D E$ are of same length having thermal linear coefficient of expansion $α_{2}$ . point $A, B, E and F$ reside on same line, that is, sections $A B and E F$ overlap. then the ratio of $\frac{l_{1}}{l_{2}}$ for which the distance between end $A$ and end $F$ remains the same at all temperatures, is

A $\frac{α_{2}}{2 α_{1}}$
B $\frac{2 α_{2}}{α_{1}}$
C $\frac{2 α_{1}}{α_{2}}$
D $\frac{α_{1}}{2 α_{2}}$
Question 2 - Select One
Two rods having length $l_{1}$ and $l_{2}$ , made of materials with the linear coefficient of expansion $α_{1}$ and $α_{2}$ were welded togther. The equivalent coefficients of linear expansion for the obtained rod:-

A $\frac{l_{1} α_{2} + l_{2} α_{1}}{l_{1} + l_{2}}$
B $\frac{l_{1} α_{1} + l_{2} α_{2}}{α_{1} + α_{2}}$
C $\frac{l_{1} α_{1} + l_{2} α_{2}}{l_{1} + l_{2}}$
D $\frac{l_{2} α_{1} + l_{1} α_{2}}{α_{1} + α_{2}}$
Question 3 - Select One
A solid rectangular sheet has two different coefficients of linear expansion $α_{1}$ and $α_{2}$ along its length and breadth respectively. The coefficient of surface expansion is (for $α_{1} t < < 1, α_{2} < < 1$ )
A $\frac{α_{1} + α_{2}}{2}$
B $2 (α_{1} + α_{2})$
C $\frac{4 α_{1} α_{2}}{α_{1} + α_{2}}$
D $α_{1} + α_{2}$

The correct Answer is:3

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two rods having length $l_{1}$ and $l_{2}$ , made of materials with the linear coefficient of expansion $α_{1}$ and $α_{2}$ were welded togther. The equivalent coefficients of linear expansion for the obtained rod:-

A solid rectangular sheet has two different coefficients of linear expansion $α_{1}$ and $α_{2}$ along its length and breadth respectively. The coefficient of surface expansion is (for $α_{1} t < < 1, α_{2} < < 1$ )

AAKASH SERIES-THERMAL PROPERTIES OF MATTER-EXPANSION OF SOLIDS (PRACTICE SHEET) LEVEL-II (Integer Type Questions)

The correct Answer is:3

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two rods having length l1 and l2, made of materials with the linear coefficient of expansion α1 and α2 were welded togther. The equivalent coefficients of linear expansion for the obtained rod:-

A solid rectangular sheet has two different coefficients of linear expansion α1and α2 along its length and breadth respectively. The coefficient of surface expansion is (for α1t<<1,α2<<1)

AAKASH SERIES-THERMAL PROPERTIES OF MATTER-EXPANSION OF SOLIDS (PRACTICE SHEET) LEVEL-II (Integer Type Questions)

Two rods having length $l_{1}$ and $l_{2}$ , made of materials with the linear coefficient of expansion $α_{1}$ and $α_{2}$ were welded togther. The equivalent coefficients of linear expansion for the obtained rod:-

A solid rectangular sheet has two different coefficients of linear expansion $α_{1}$ and $α_{2}$ along its length and breadth respectively. The coefficient of surface expansion is (for $α_{1} t < < 1, α_{2} < < 1$ )