z∈C में निम्न का बिंदुपथ ज्ञात कीजिएः
a. 1<|z−2i|<3
b. Re(z+2iiz+2)≤r(z≠2i)
c. Arg(z+i)−Arg(z−i)=π/2
d. Arg(z−a)=π/3 जहां a=3+4i
The correct Answer is:(a) संकेन्द्रीय वृत्त जिसका केन्द्र(0, 2) पर तथा त्रिज्यायें1 व 3 इकाई है। (b) केन्द्र , +25 तथा त्रिज्या के वृत्त पर या बाह्य क्षेत्र (c) अर्द्ध वृत्त x2+32=1 (प्रथम व चतुर्थ चतुर्थांश में (d) बिन्दु (3 +4i) से निकलने वाली किरण जो मूल बिन्दु से दूर जाती है तथा जिसका समीकरण √3x−y+4−3√3=0 है|
Step by step video, text & image solution for zinC में निम्न का बिंदुपथ ज्ञात कीजिएः a. 1lt|z-2i|lt3 b. Re((z+2i)/(iz+2))ler(z!=2i) c. Arg(z+i)-Arg(z-i)=pi//2 d. Arg(z-a)=pi//3 जहां a=3+4i by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLEN HINDICHAPTER - सम्मिश्र संख्या (COMPLEX NUMBER)EXERCISE - EXERCISE-04 [B] (BRAIN STORMING SUBJECTIVE EXERCISE)10videos
सम्मिश्र संख्या (COMPLEX NUMBER)
- BOOK - ALLEN HINDICHAPTER - सम्मिश्र संख्या (COMPLEX NUMBER)EXERCISE - EXERCISE-03 (MISCELLANEOUS TYPE QUESTIONS) गद्यांश आधारित प्रश्न (Comprehension Based Questions) गद्यांश # 2:3videos
सम्मिश्र संख्या (COMPLEX NUMBER)
- BOOK - ALLEN HINDICHAPTER - सम्बन्ध EXERCISE - EXERCISE-II6videos
सम्बन्ध
Similar Questions
माना z= x + iy एक सम्म्मिश्र संख्या है जहाँ x तथा y वास्तविक है। माना A तथा B समुच्चय द्वारा परिभाषित है A={z∣|z|≤2} तथा B={z∣(1−i)z+(1+i)¯z≥4} क्षेत्र A∩B द्वारा दर्शाया गया क्षेत्रफल ज्ञात कीजिये।
01:42View Solutionदिए गए चित्र में |z|=r, त्रिभुज ABC का परिवृत है। D, E व F क्रमशः भुजाओं BC, CA तथा AB के मध्य बिन्दु हैं। AD आगे बढ़ाने पर वृत्त को L पर मिलाता है। यदि ∠CAD=0,AD=x,BD=y और त्रिभुज ABC का बिन्दु A से डाला गया शीर्षलम्ब, वृत्त |z|=r को M पर मिलाता है। यदि बिन्दुओं A,B तथा C के आगम क्रमशः za,zb तथा zc है-
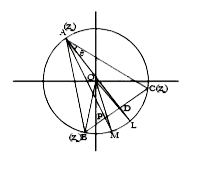
त्रिभुज ABC का क्षेत्रफल है -03:05View Solutionमानाकि R = {(1, 3), (4, 2), (2, 4), (2, 3), (3, 1)} समुच्चय A = {1, 2, 3, 4} में एक संबंध है, तब संबंध R होगा-
04:00View SolutionIf z is a complex number such that |z|=4 and arg(z)=5π6 , then z is equal to −2√3+2i b. 2√3+i c. 2√3−2i d. −√3+i
04:32View SolutionIf arg(z)<0, then arg(−z)−a r g(z) equals π (b) −π (d) −π2 (d) π2
02:32View SolutionThe number of values of z satisfying both theequations Arg(z-1-i)=-(3 pi)/(4) and Arg(z-2-2i)=(pi)/(4) is
03:19View Solutionif arg(z+a)=(pi)/(6) and arg(z-a)=(2 pi)/(3) then
04:08View SolutionIf -pi
01:55View SolutionNumber of z, which satisfy Arg(z-3-2i)=(pi)/(6) and Arg(z-3-4i)=(2 pi)/(3) is/are :
03:10View SolutionIf arg(z+a)=π/6andarg(z−a)=2π/3(a∈R+), then
02:57View SolutionIf Arg (3z-2i) = pi//2 then the locus of z = x +iy is
03:54View SolutionIf arg (z−(10+6t)z−(4+2i))=π5 (where z is a complex number), then the perimeter of the locus of z is
02:27View SolutionThe locus of z such that arg[(1−2i)z−2+5i]=π4 is a
01:37View SolutionIf|z-i|=1 &Arg(z)=π2 then the z is (1) −2i (2) 0 (4) 2i
11:17View SolutionIf z=1+2i1−(1−i)2 then find Arg z
01:31View SolutionIf z=1+2i1−(1−i)2, then arg(z) equals a. 0 b. π2 c. π d. non of these
02:50View Solution

