Two containers of equal volume contain the same gas at pressures and absolute temperatures respectively. On joining the vessels, the gas reaches a common pressure Panda common temperature T. Find the value P/T.
Number of moles before joining =
Number of mo]es after joining the vessels.
i.e.,
(or)
Similar Questions
Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View SolutionTwo containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View Solution
Knowledge Check
Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
AP1T1+P2T2BP1T1+P2T2(T1+T2)2CP1T2+P2T2(T1+T1)2DP12T1+P22T2Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
Ap1T2+p2T1T1×T2Bp1T2+p2T1T1+T2C12[p1T2+p2T1T1T2]Dp1T2−p2T1T1×T2]Two identical vessels contain the same gas at pressure P1 and P2 at absolute temperature T1andT2, respectively. On joining the vessels with a small tube as shown in the figure. The gas reaches a common temperature T and a common pressure P. Determine the ratio P/T
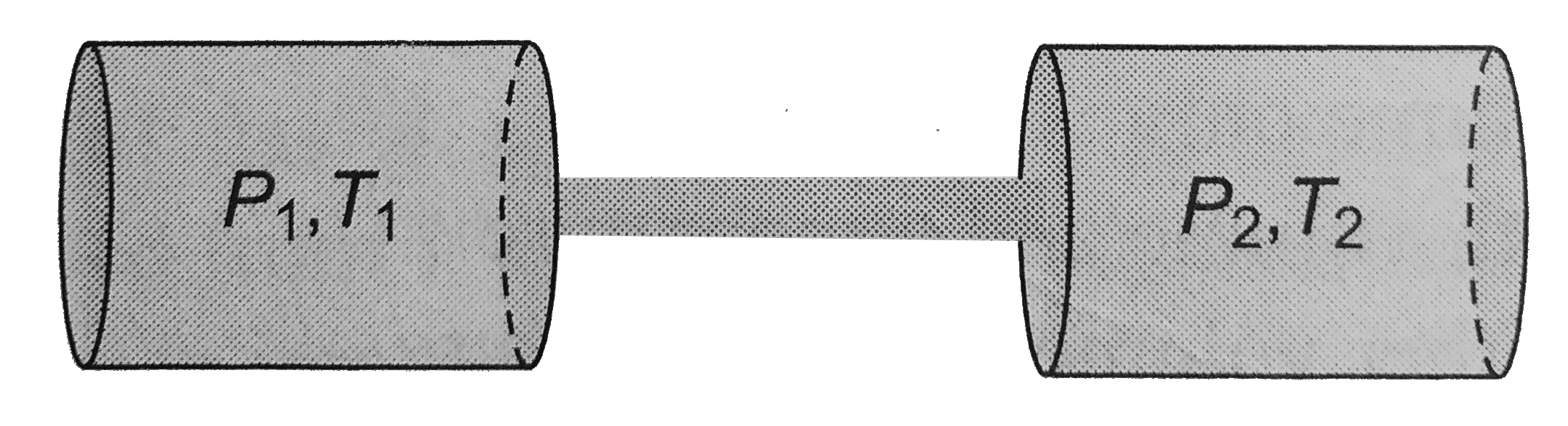 A[P1T1+P2T2T1T2]B12[P1T1+P2T2T1T2]C12[P1T2+P2T1T1T2]D[P1T2+P2T1T1T2]
A[P1T1+P2T2T1T2]B12[P1T1+P2T2T1T2]C12[P1T2+P2T1T1T2]D[P1T2+P2T1T1T2]
Two vessels of volume (V1)and(V2) contain the same ideal gas. The pressures in the vessels are (P1)and(P2) and the temperatures are (T1)and(T2) respectively . The two vessels are now connected to each other through a narrow tube. Assuming that no heat is exchanged between the surrounding and the vessels, find the common pressure and temperature attained after the connection.
View SolutionTwo vessels of volume (V1)and(V2) contain the same ideal gas. The pressures in the vessels are (P1)and(P2) and the temperatures are (T1)and(T2) respectively . The two vessels are now connected to each other through a narrow tube. Assuming thar no heat is exchanged between the surrounding and the vessels, find the common pressure and temperature attained after the connection.
View SolutionTwo idential container joined by a small pipe initially contain the same gas at pressure p0 and absolute temperature T0. One container is now maintained at the same temperature while the other is heated to 2T0. The common pressure of the gas
View SolutionAn ideal gas in a thermally insulated vessel at internal pressure = P1, volume =V1 and absolute temperature =T1 expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P2,V2andT2, respectively. For this expansion.
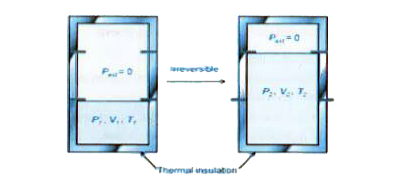
View SolutionTwo idential container joined by a small pipe initially contain the same gas at pressure p0 and absolute temperature T0. One container is now maintained at the same temperature while the other is heated to 2T0. The common pressure of the gas
View Solution
