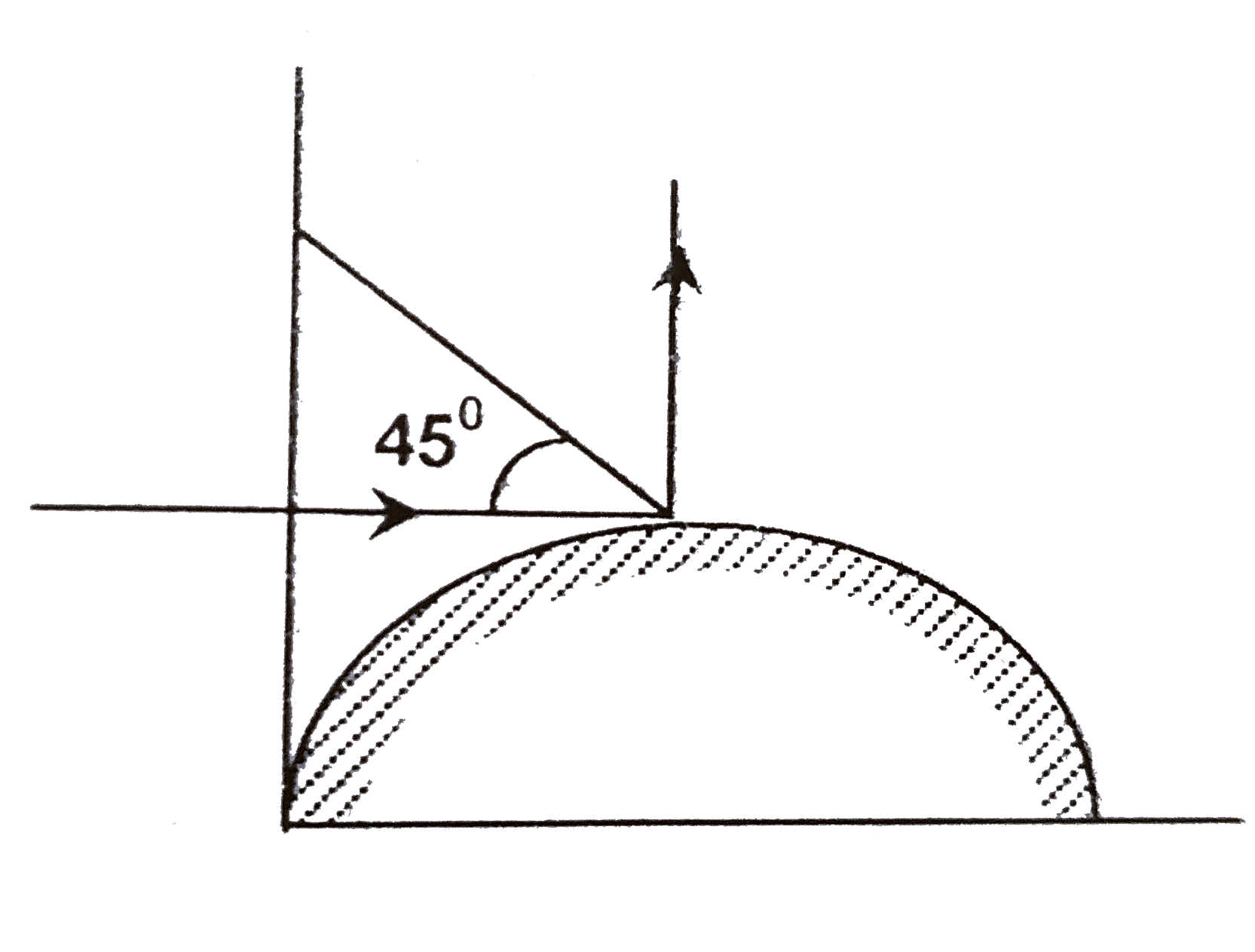
A reflecting surface is represented by the equation Y=2Lπsin(πxL),0≤x≤L A ray travelling horizontally becomes vertical after reflection. The coordinates of the point (s) where this ray is incident is
A horizontal ray becomes vertical after reflection
tanθ=dydx=2cos(πx)L
2θ=90∘θ=45∘
1=2cos(πx/L)⇒x=L/3
∴y=2Lπsin(π/3)=√3Lπ(L3,√3Lπ)&(2L3,√3Lπ)

Topper's Solved these Questions
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE I
95 Videos
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE 1B
45 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
Similar Questions
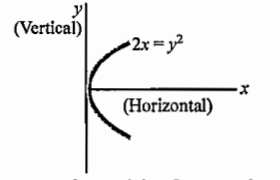
The reflecting surface is represented by the equation 2x=y2 as shown in the figure. A ray travelling horizontal becomes vertical after reflection. The co-ordinates of the point of incidence are
View SolutionA reflecting surface is represented by the equation
y=2Lπsin(πxL), where 0≤x≤L. A ray of light travelling horizontally becomes vertical after reflection with the surface. The co-ordinates of the point where this ray is incident is.
View Solution
Knowledge Check
A reflecting surface is represented by the equation
y=2Lπsin(πxL), where 0≤x≤L. A ray of light travelling horizontally becomes vertical after reflection with the surface. The co-ordinates of the point where this ray is incident is.
 A(L4,√2Lπ)B(L3,√3Lπ)C(3L4,√2Lπ)D(2L3,√3Lπ)
A(L4,√2Lπ)B(L3,√3Lπ)C(3L4,√2Lπ)D(2L3,√3Lπ)A refracting surface is represented by the equation x2+y2=a2. A ray travelling in negative x-directed towards positive y-direction after reflection from the surface at point P. Then co-ordinates of point P are
 A(0.8 a, 0.6 a)B(0.6 a, 0.8 a)C(a2,a2)DNone of these
A(0.8 a, 0.6 a)B(0.6 a, 0.8 a)C(a2,a2)DNone of theseA wave is represented by the equation y=Asin(10πx+15πl+π6) wher x is in metre and t in second. The expression represents
AA wave travelling in negative x - direction with a velocity of 1.5 m/sBA wave travelling in positive x direction with a velocity of 1.5 m/sCA wave travelling in position x - direction with wavelength 0.2 mDA wave travelling in negative x-direction with a velocity of 150 m/s
A refracting surface is represented by the equation x2+y2=a2. A ray travelling in negative x-directed towards positive y-direction after reflection from the surface at point P. Then co-ordinates of point P are
View SolutionSolve the equation for 0≤x≤2π.
sinxcosx=0
View SolutionIf the equation of mirror is given by y=2/πsinπx(y>0,0≤x≤1) then find the point on which horizontal ray should be incident so that the reflected ray become perpendicular to the incident ray
_E01_073_Q01.png)
View SolutionIf a ray travelling the line x = 1 gets reflected the line x+y=1 then the equation of the line along which the reflected ray travels is
View SolutionIf the equation of mirror is given by y=2/πsinπx(y>0,0≤x≤1) then find the point on which horizontal ray should be incident so that the reflected ray become perpendicular to the incident ray
_E01_073_Q01.png)
View Solution
