A mark on the surface of a glass sphere is viewed from a diametrically opposite position. It appears to be at a distance 10cm from its actual position Find the radius of sphere.
As the mark is viewed from the diametrically opposite position, refraction takes place at side II of the surface (the mark being on side I as shown)
Here using
negative sign indicates that the image is formed to the left of refracting surface as shown in figure. Further it is given that, the image of mark is at a distance 10cm from the object. Hence:

Topper's Solved these Questions
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE I
95 Videos
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE 1B
45 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
Similar Questions
A mark on the surface of a glass spehre (μ=1.5) is viewed from a diametrically opposite position. It appears to be at a distance 10 cm from its actual position. The radius of the sphere is
View SolutionA mark of the surface of sphere (μ=32) is viewed from a diametrically opposite position. It appears to be at a distance 15 cm from its actual position.Find the radius of sphere.
View Solution
Knowledge Check
A mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is 10cm and refractive index of glass is 1.5, find the position of the image.
A−20cmB30cmC40cmD−10cm
A small object stuck on the surface of a glass sphere (n=1.5) is viewed from the diametrically opposite position. Find transverse magnification.
View SolutionA small object stuck on the surface of a glass sphere (n=1.5) is viewed from the diametrically opposite position. Find transverse magnification.
View SolutionA small object stuck on the surface of a glass sphere (μ=4/3) is viewed from the diametrically opposite position find transverse magnification.
View SolutionA mark placed on the surface of glass sphere is viewed through glass from an oppositely directed position. If the diameter of the sphere is 30 cm, locate the image of the mark. For glass, μ=1.5
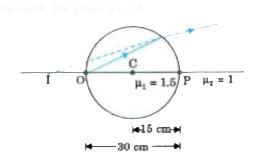
View SolutionA small air bubble is situated at a distance of 3 cm from the center of a glass sphere of radius 9 cm. When viewed from the nearest side, the bubble appears to be at a distance of 5 cm from the surface. Its apparent distance when viewed from the farthest side is n×5 cm where n is?
View SolutionA mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is 10cm and refractive index of glass is 1.5, find the position of the image.
View SolutionIf a mark of size 0.2 cm on the surface of a glass sphere of diameter 10 cm and μ=1.5is viewed through the diametrically opposite point, where will the image be seen and of what size? (in mm)
View Solution
