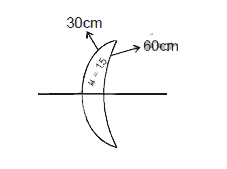
A thin equi-convex lens having radius of curvature 10cm is placed as shown in figure . Calculate the focal length of the lens, if parallel rays are incident as shown

Text Solution

Verified by Experts

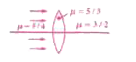
Refraction at 1st surface $u = \infty, μ_{1} = \frac{5}{4} μ_{2} = \frac{5}{3}$
$\frac{μ_{2}}{v} - \frac{μ_{1}}{u} = \frac{μ_{2} - μ_{1}}{R_{1}} \Rightarrow \frac{5}{3 v} = \frac{\frac{5}{3} - \frac{5}{4}}{10} \Rightarrow v = 40 c m$
Refraction at second surface
$μ_{1} = \frac{5}{3}, μ_{2} = \frac{3}{2} μ = + 40 v = ? R = - 10$
$\frac{3}{2 v} - \frac{5}{3 \times 40} = \frac{\frac{3}{2} - \frac{5}{3}}{- 10}$ After solving we get $V = \frac{180}{7} c m$
So focal length $= \frac{180}{7} c m$

A thin equi-convex lens having radius of curvature 10 cm is placed as shown in figure. Calculate focal length of the lens, if parallel rays are incident as shown.

A $\frac{120}{7}$ cm
B $\frac{160}{7}$ cm
C $\frac{180}{7}$ cm
DNone
A plano-convex lens has refractive index 1.6 and radius of curvature 60 cm. What is the focal length of the lens?
A50 cm
B100 cm
C200 cm
D150 cm
If the bio-convex lens is cut as shown in the figure, the new focal length f' is
A2f
Bf
C(f)/(2)
DInfinite