For a spring-mass system spring having spring constant 19.7 N/m is attached to it. What should be the value of mass m (approx.) so that it will give the same period as that of seconds pendulum?
1 kg
2 kg
3 kg
4 kg
The correct Answer is:B
To solve the problem, we need to find the mass m that will give the same period as a seconds pendulum, which has a period of 2 seconds. We will use the formula for the time period of a spring-mass system and set it equal to the period of the seconds pendulum.
1. Understand the time period of a spring-mass system:
The formula for the time period T of a spring-mass system is given by:
T=2π√mk
where m is the mass attached to the spring and k is the spring constant.
2. Set the time period equal to that of a seconds pendulum:
The time period of a seconds pendulum is 2 seconds. Therefore, we can set:
2=2π√mk
3. Simplify the equation:
Divide both sides by 2:
1=π√mk
4. Square both sides:
Squaring both sides gives:
1=π2mk
5. Rearrange to solve for m:
Rearranging the equation gives:
m=kπ2
6. Substitute the value of k:
Given that the spring constant k=19.7N/m:
m=19.7π2
7. Calculate the value of m:
Using π≈3.14:
π2≈9.86
Therefore:
m≈19.79.86≈2.00kg
Thus, the approximate value of mass m is 2 kg.
The formula for the time period T of a spring-mass system is given by:
T=2π√mk
where m is the mass attached to the spring and k is the spring constant.
The time period of a seconds pendulum is 2 seconds. Therefore, we can set:
2=2π√mk
Divide both sides by 2:
1=π√mk
Squaring both sides gives:
1=π2mk
Rearranging the equation gives:
m=kπ2
Given that the spring constant k=19.7N/m:
m=19.7π2
Using π≈3.14:
π2≈9.86
Therefore:
m≈19.79.86≈2.00kg
Topper's Solved these Questions
Similar Questions
A system of springs with their spring constants are as shown in figure. What is the frequency of oscillations of the mass m ?

View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View Solution
Knowledge Check
Two springs of spring constant 6N/m and 4N/m are attached to a block of mass 1 kg and to fixed support. Then time period of the given oscillation is
(consider √10=π)A1sB2sC0.5sD4sA student working with a spring mass system finds the values of mass, spring constant and damping constant in CGS unit as 90,4.5×10+3 and 180 respectively. What will be the time period of the spring mass system ?
A2π/7B14πCπD2π/√50Two springs of spring constants 1000 N/m and 2000 N/m are stretched with same force. They will have potential energy in the ratio of
A2:1B22:12C1:2D12:22
The pendulum of a clock is replaced by a spring mass system with the spring having spring constant 0.1Nm−1. What mass should be attached to the spring?
View SolutionFour springs of constant as shown are attached to a pair of masses m each as shown. The time period will be 2π times
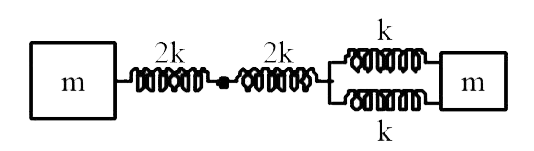
View SolutionA spring having a spring constant k is loaded with a mass m. The spring is cut into two equal parts and one of these is loaded again with the same mass. The new spring constant i
View SolutionA spring mass system has time period of 2 second. What should be the spring constant of spring if the mass of the block is 10grams ?
View SolutionA spring mass system has time period of 2 second. What should be the spring constant of spring if the mass of the block is 10grams ?
View Solution
