A horizontal spring block system ( mass m_2 spring constant k) is oscillating on a smooth surface with amplitude A. If at the mean position an identical block is kept on it so that they move together after what is the new amplitude?
A
A2
A√2
A2√2
To solve the problem, we need to determine the new amplitude of a spring-block system after an identical block is placed on it at the mean position.
1. Understanding the Initial System:
- We have a spring with spring constant k and a block of mass m2 oscillating with an amplitude A.
- The maximum velocity V of the block at the mean position can be expressed as:
V=Aω
where ω=√km2.
2. Adding the Identical Block:
- At the mean position, we place an identical block of mass m2 on the first block.
- The total mass of the new system becomes 2m2.
3. Applying Conservation of Momentum:
- Before adding the second block, the momentum of the first block at the mean position is:
pinitial=m2⋅V=m2⋅(Aω)
- After placing the second block, the new system has a combined mass of 2m2 and a new velocity V′:
pfinal=2m2⋅V′
4. Setting Initial and Final Momentum Equal:
- By conservation of momentum:
m2⋅(Aω)=2m2⋅V′
- We can cancel m2 from both sides (assuming m2≠0):
Aω=2V′
- Therefore, the new velocity V′ is:
V′=Aω2
5. Finding the New Amplitude:
- The new amplitude A′ can be expressed in terms of the new velocity and the new angular frequency ω′:
V′=A′ω′
- The new angular frequency ω′ for the combined mass is:
ω′=√k2m2
- Substituting ω′ into the equation for V′:
Aω2=A′√k2m2
6. Substituting ω:
- We know ω=√km2, so substituting this in:
A√km22=A′√k2m2
7. Solving for A′:
- Rearranging gives:
A′=A√km22√k2m2
- Simplifying:
A′=A√2
Final Answer:
The new amplitude A′ after placing the identical block is:
A′=A√2
- We have a spring with spring constant k and a block of mass m2 oscillating with an amplitude A.
- The maximum velocity V of the block at the mean position can be expressed as:
V=Aω
where ω=√km2.
- At the mean position, we place an identical block of mass m2 on the first block.
- The total mass of the new system becomes 2m2.
- Before adding the second block, the momentum of the first block at the mean position is:
pinitial=m2⋅V=m2⋅(Aω)
- After placing the second block, the new system has a combined mass of 2m2 and a new velocity V′:
pfinal=2m2⋅V′
- By conservation of momentum:
m2⋅(Aω)=2m2⋅V′
- We can cancel m2 from both sides (assuming m2≠0):
Aω=2V′
- Therefore, the new velocity V′ is:
V′=Aω2
- The new amplitude A′ can be expressed in terms of the new velocity and the new angular frequency ω′:
V′=A′ω′
- The new angular frequency ω′ for the combined mass is:
ω′=√k2m2
- Substituting ω′ into the equation for V′:
Aω2=A′√k2m2
- We know ω=√km2, so substituting this in:
A√km22=A′√k2m2
- Rearranging gives:
A′=A√km22√k2m2
- Simplifying:
A′=A√2
The new amplitude A′ after placing the identical block is:
A′=A√2
Similar Questions
A horizontal spring block system of mass M executes simple haormonic motion. When the block is passing through its equilibrium position, an object of mass m is put on it and the two move together. Find the new amplitude and frequency of vibration. Given, k is the spring constant of the system.
View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View Solution
Knowledge Check
A mass m, connected to a horizontal spring performs SHM with amplitude A. While mass m, is passing through mean position, another mass m, is placed on it so that both the masses move together with amplitude A,. The ratio of
A[m1m1+m2]12B[m1+m2m1]12C[m2m1+m2]12D[m1+m2m2]12A horizontal spring-block system of mass 1kg executes SHM of amplitude 10 cm. When the block is passing through its equilibrium position another mass of 1kg is put on it and the two move together.
Aamplitude will remain unchangedBamplitude will become 5√2 cmCthe frequency of oscillations will remain sameDthe frequency of oscillations will decrease.A horizontal spring -block system of mass 2kg executes S.H.M when the block is passing through its equilibrium positiojn an object of mass 1kg is put on it the two move togather The new amplitude of vibration is (A being its initial amplitude)
A√23AB√32AC√2AD√A√2
An object of mass M oscillating with amplitude 'A' mass 'm' is added at mean position. Find new amplitude of oscillation.
View SolutionA block of mass M attached to the free end of a spring of force constant k is nounted on a smooth horizontal table as shown in figure.
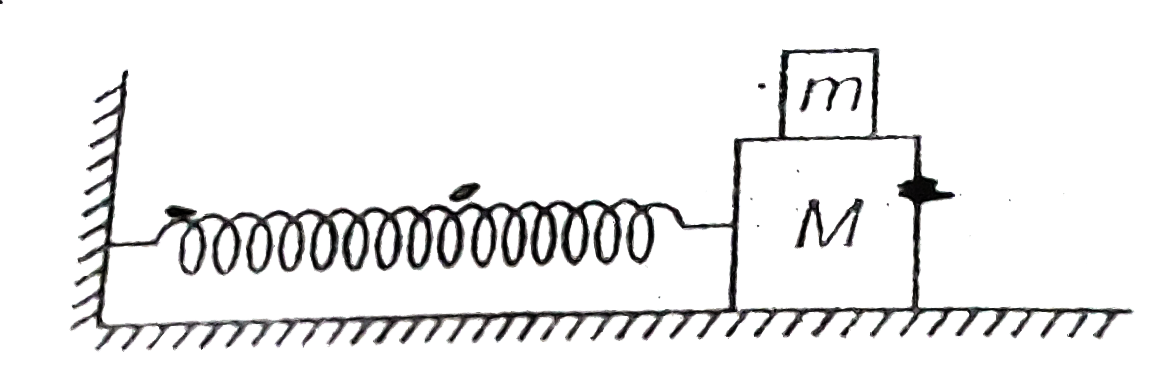
The block executes SHM with amplitude A and frequency f . If an object of mass m is put on it, when the block is passing through its equilibium position and the two move together ,then what is the new amplitude and frequency of vibration?
View SolutionTwo blocks A and B of masses m and 2m are connected by a massless spring of natural length L and spring constant k. The blocks are intially resting on a smooth horizontal floor with the spring at its natural length , as shown . A third identical block C of mass m moves on the floor with a speed v0 along the line joining A and B and collides elastically with A. FInd (a) the velocity of c.m. of system (blockA+B+spring) and (b) the minimum compression of spring.

View SolutionIn the given figure, a mass M is attached to a horizontal spring which is fixed on one side to a rigid support. The spring constant of the spring is k. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass is in equilibrium position, as shown in the figure, another mass m is gently fixed upon it. The new amplitude of oscillation will be :

View SolutionPassage III A block of mass m =1/2 kg is attached with two springs each of force contant k=10N/m as shown. The block is oscillating vertically up and down with amplitude A=25cm. When the block is at its mean position one of the spring breaks without changing momentum of block.
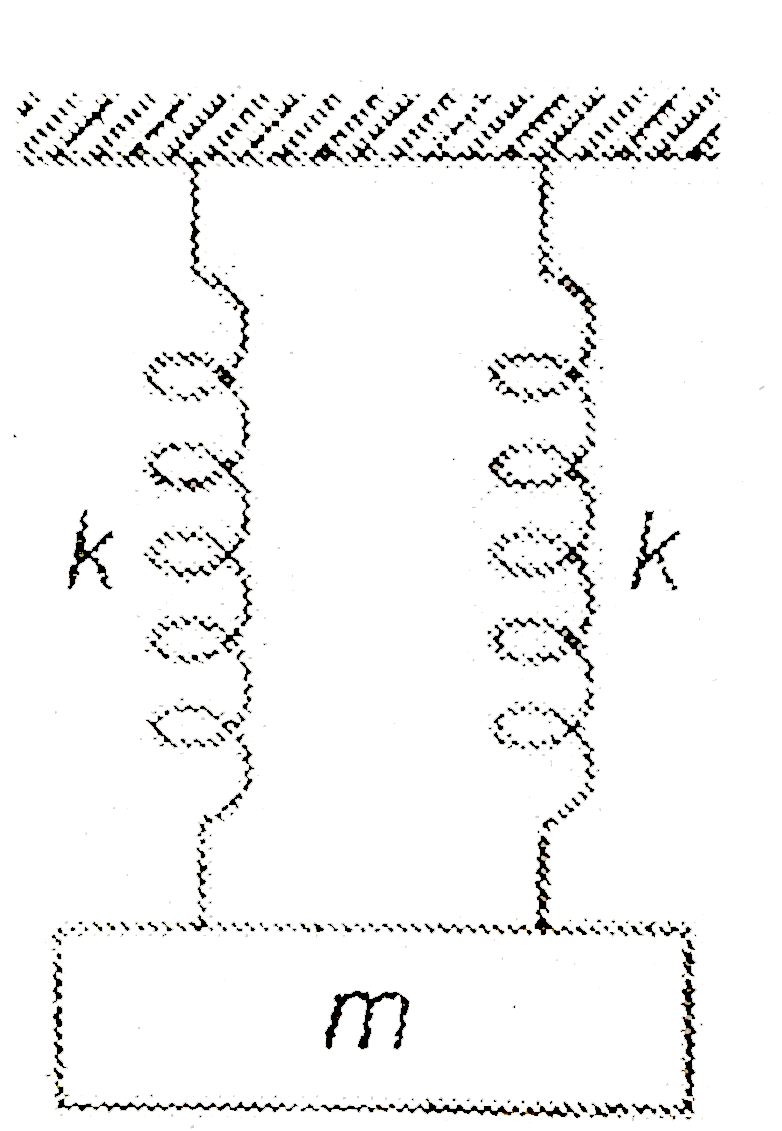
What is the new amplitude of osciallation of the block.
View Solution
