An object of mass M oscillating with amplitude 'A' mass 'm' is added at mean position. Find new amplitude of oscillation.
To solve the problem of finding the new amplitude of oscillation when an additional mass m is added to an object of mass M that is oscillating with an initial amplitude A, we can follow these steps:
1. Identify Initial Conditions:
- The initial mass is M.
- The initial amplitude is A.
- The system is oscillating with a total mechanical energy given by:
E=12kA2
where k is the spring constant.
2. Kinetic Energy at Mean Position:
- At the mean position, the potential energy is zero, and the total mechanical energy is equal to the kinetic energy:
E=12Mv2
where v is the velocity of the mass M just before adding mass m.
3. Conservation of Momentum:
- Just before the mass m is added, the momentum of the system is:
pinitial=Mv
- After adding mass m, the new mass of the system becomes M+m and let the new velocity be v′:
pfinal=(M+m)v′
- By conservation of momentum:
Mv=(M+m)v′
4. Expressing New Velocity:
- From the momentum conservation equation, we can express v′:
v′=MvM+m
5. New Total Mechanical Energy:
- The total mechanical energy after adding mass m at the mean position is:
E′=12kA′2
- The kinetic energy at this point is:
E′=12(M+m)(v′)2
6. Equating Energies:
- Set the expressions for total mechanical energy before and after equal:
12kA2=12(M+m)(MvM+m)2
7. Simplifying the Equation:
- Cancel 12 from both sides and simplify:
kA2=(M+m)M2v2(M+m)2
- Rearranging gives:
A2=M2v2(M+m)⋅1(M+m)
8. Finding New Amplitude:
- Substitute v2 from the initial energy equation:
v2=kA2M
- Substitute this into the equation for A′2:
A′2=A2⋅M+mM
- Taking the square root gives:
A′=A√M+mM
Final Result:
The new amplitude of oscillation after adding mass m is:
A′=A√M+mM
- The initial mass is M.
- The initial amplitude is A.
- The system is oscillating with a total mechanical energy given by:
E=12kA2
where k is the spring constant.
- At the mean position, the potential energy is zero, and the total mechanical energy is equal to the kinetic energy:
E=12Mv2
where v is the velocity of the mass M just before adding mass m.
- Just before the mass m is added, the momentum of the system is:
pinitial=Mv
- After adding mass m, the new mass of the system becomes M+m and let the new velocity be v′:
pfinal=(M+m)v′
- By conservation of momentum:
Mv=(M+m)v′
- From the momentum conservation equation, we can express v′:
v′=MvM+m
- The total mechanical energy after adding mass m at the mean position is:
E′=12kA′2
- The kinetic energy at this point is:
E′=12(M+m)(v′)2
- Set the expressions for total mechanical energy before and after equal:
12kA2=12(M+m)(MvM+m)2
- Cancel 12 from both sides and simplify:
kA2=(M+m)M2v2(M+m)2
- Rearranging gives:
A2=M2v2(M+m)⋅1(M+m)
- Substitute v2 from the initial energy equation:
v2=kA2M
- Substitute this into the equation for A′2:
A′2=A2⋅M+mM
- Taking the square root gives:
A′=A√M+mM
The new amplitude of oscillation after adding mass m is:
A′=A√M+mM
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
An iron ring of mass m1 oscillates in its an plane with time period of π sec. Assume that the amplitude of oscillation is small. When it is passing through the mean position, a magnet of mass m is attached to the lowest point. If the amplitude of oscillation decreases by factor of 7, find mm1.
View SolutionLength of a stretched wire is 2m. It is oscillating in its fourth overtone mode. Maximum amplitude of oscillations is 2mm. Find amplitude of oscillation at a distance of 0.2m from one fixed end.
View Solution
Knowledge Check
At resonance the amplitude of forced oscillations is
AminimumBmaximumCzeroDNone of theseAt resonance, the amplitude of forced oscillations is
AminimumBmaximumCzeroDnone of theseIn the case of sustained force oscillations the amplitude of oscillations
Adecreases linearlyBdecreases sinusodiallyCdereases exponentiallyDalways remains constant
A uniform rod of length 1.00 m is suspended through an end and is set into oscillation with small amplitude under gravity. Find the time period of oscillation.
View SolutionA block of mass m attached to a massless spring is performing oscillatory motion of amplitude A on a frictionless horizontal plane. If half of the mass of the block off when it is passing through its mean position, the amplitude of oscillation for the remaining system become fA. The value of f is .
View SolutionIn the previous question, the amplitude of the oscillation is
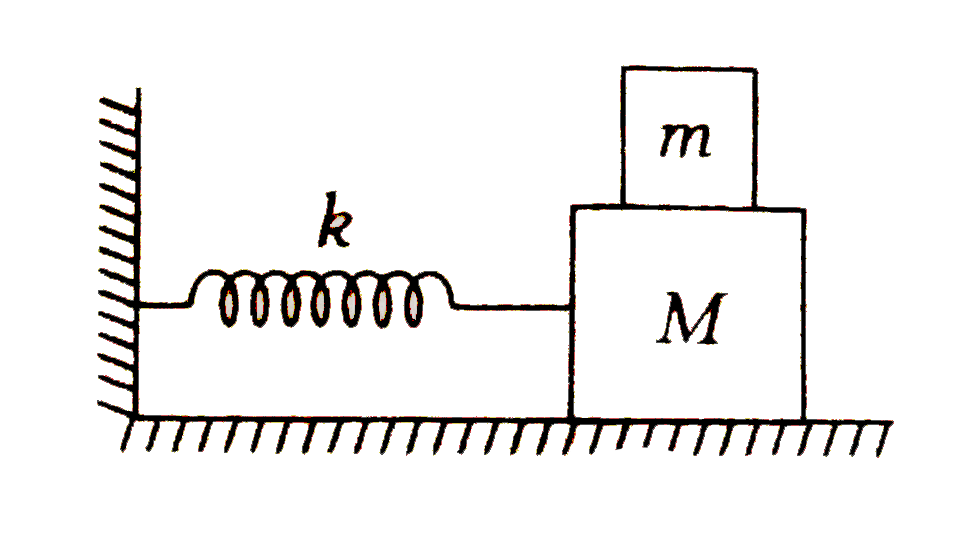
View SolutionIn the given figure, a mass M is attached to a horizontal spring which is fixed on one side to a rigid support. The spring constant of the spring is k. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass is in equilibrium position, as shown in the figure, another mass m is gently fixed upon it. The new amplitude of oscillation will be :

View SolutionA mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
View Solution
