In a photoelectric effect experiment, the stopping potential is 0.71 V and corresponding wavelength of incident photon was 491 nm. Now the stopping potential of battery is increased to 1.4 V. Find new wavelength of incident photon ?
The correct Answer is:B
To solve the problem, we will use the concept of the photoelectric effect, which relates the energy of the incident photons to the stopping potential and the work function of the material.
1. Understanding the Photoelectric Effect:
The energy of the incident photon can be expressed as:
E=hcλ
where h is Planck's constant (6.626×10−34Js), c is the speed of light (3×108m/s), and λ is the wavelength of the incident light.
2. Relating Energy to Stopping Potential:
The stopping potential V0 is related to the kinetic energy of the emitted electrons:
E=eV0+ϕ
where e is the elementary charge (1.6×10−19C) and ϕ is the work function of the material.
3. Setting Up the Equations:
For the first condition (with V0=0.71V and λ=491nm):
hc491×10−9=e⋅0.71+ϕ
For the second condition (with V0=1.4V and unknown λ2):
hcλ2=e⋅1.4+ϕ
4. Substituting Values:
We can substitute h and c:
hc=1240eV nm
Thus, the first equation becomes:
1240491=0.71+ϕe
And the second equation becomes:
1240λ2=1.4+ϕe
5. Subtracting the Equations:
Subtract the first equation from the second:
1240λ2−1240491=1.4−0.71
Simplifying gives:
1240λ2−1240491=0.69
6. Finding λ2:
Rearranging gives:
1240λ2=0.69+1240491
Calculate 1240491:
1240491≈2.53
Thus:
1240λ2=0.69+2.53=3.22
Therefore:
λ2=12403.22≈385.09nm
7. Final Answer:
The new wavelength of the incident photon is approximately:
λ2≈385nm
The energy of the incident photon can be expressed as:
E=hcλ
where h is Planck's constant (6.626×10−34Js), c is the speed of light (3×108m/s), and λ is the wavelength of the incident light.
The stopping potential V0 is related to the kinetic energy of the emitted electrons:
E=eV0+ϕ
where e is the elementary charge (1.6×10−19C) and ϕ is the work function of the material.
For the first condition (with V0=0.71V and λ=491nm):
hc491×10−9=e⋅0.71+ϕ
For the second condition (with V0=1.4V and unknown λ2):
hcλ2=e⋅1.4+ϕ
We can substitute h and c:
hc=1240eV nm
Thus, the first equation becomes:
1240491=0.71+ϕe
And the second equation becomes:
1240λ2=1.4+ϕe
Subtract the first equation from the second:
1240λ2−1240491=1.4−0.71
Simplifying gives:
1240λ2−1240491=0.69
Rearranging gives:
1240λ2=0.69+1240491
Calculate 1240491:
1240491≈2.53
Thus:
1240λ2=0.69+2.53=3.22
Therefore:
λ2=12403.22≈385.09nm
The new wavelength of the incident photon is approximately:
λ2≈385nm
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
In a photoelectric effect experiment, the graph of stopping potential V versus reciprocal of wavelength obtained is shown in the figure. As the intensity of incident radiation is increased :

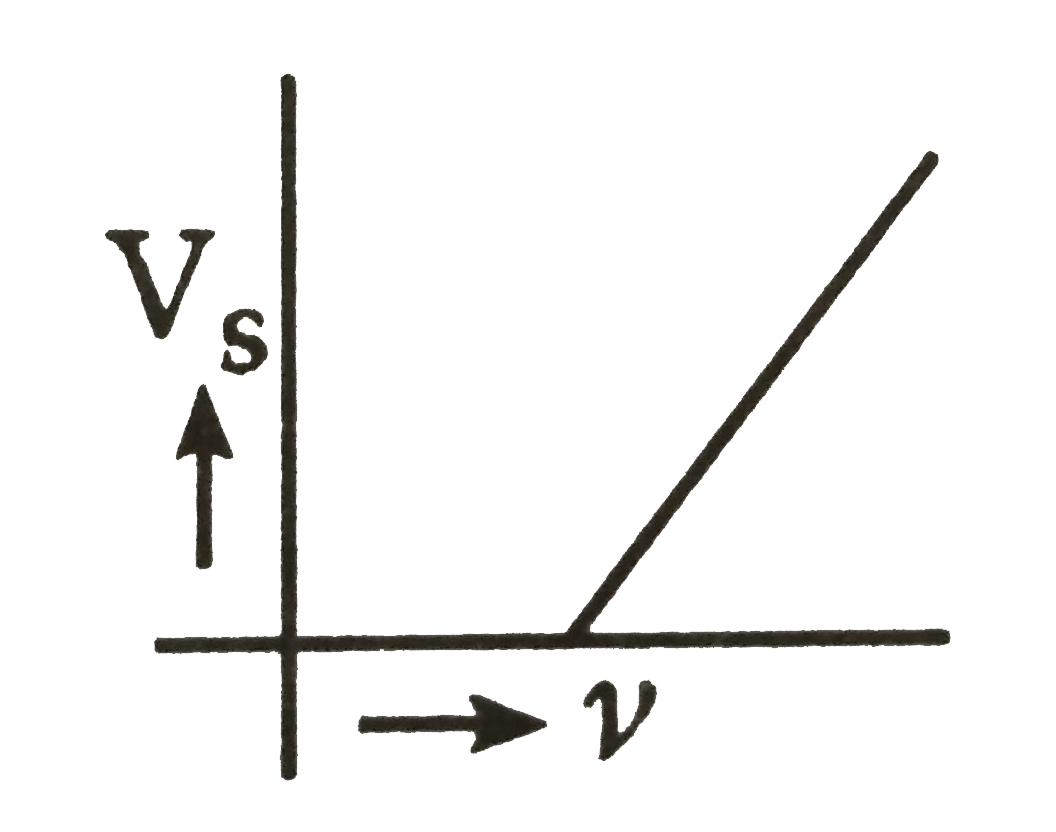
View SolutionIn photoelectric effect the slope of straight line graph between stopping potential (Vs) and frequency of incident light (v) gives
View Solution
Knowledge Check
In photoelectric effect experiment, the stopping potential for incident light of wavelength 4000 Å, is 3V. If the wavelength is changed to 2500 Å, the stopping potential will be
A3 VBmore than 3 VCless than 3 VDZero voltFor a photocell, the work function is ϕ and the stopping potential is Vs . The wavelength of the incident radiation is
AhcϕBhcϕ−eVsChcϕ+eVsDhceϕ+VsIn a photoelectric experiment the stopping potential for the incident light of wavelength 4000Å is 2 volt. If the wavelength be changed to 3000 Å, the stopping potential will be
A2VBzeroCless than 2 VDmore than 2 V
In a photoelectric effect experiment, the slope of the graph between the stopping potential and the incident frequency will be :
View SolutionIn a photoelectric effect experiment, the slope of the graph between the stopping potential and the incident frequency will be :
View SolutionIn photoelectric effect the slope of stop of stopping potential versus frequency of incident light for a given surface will be
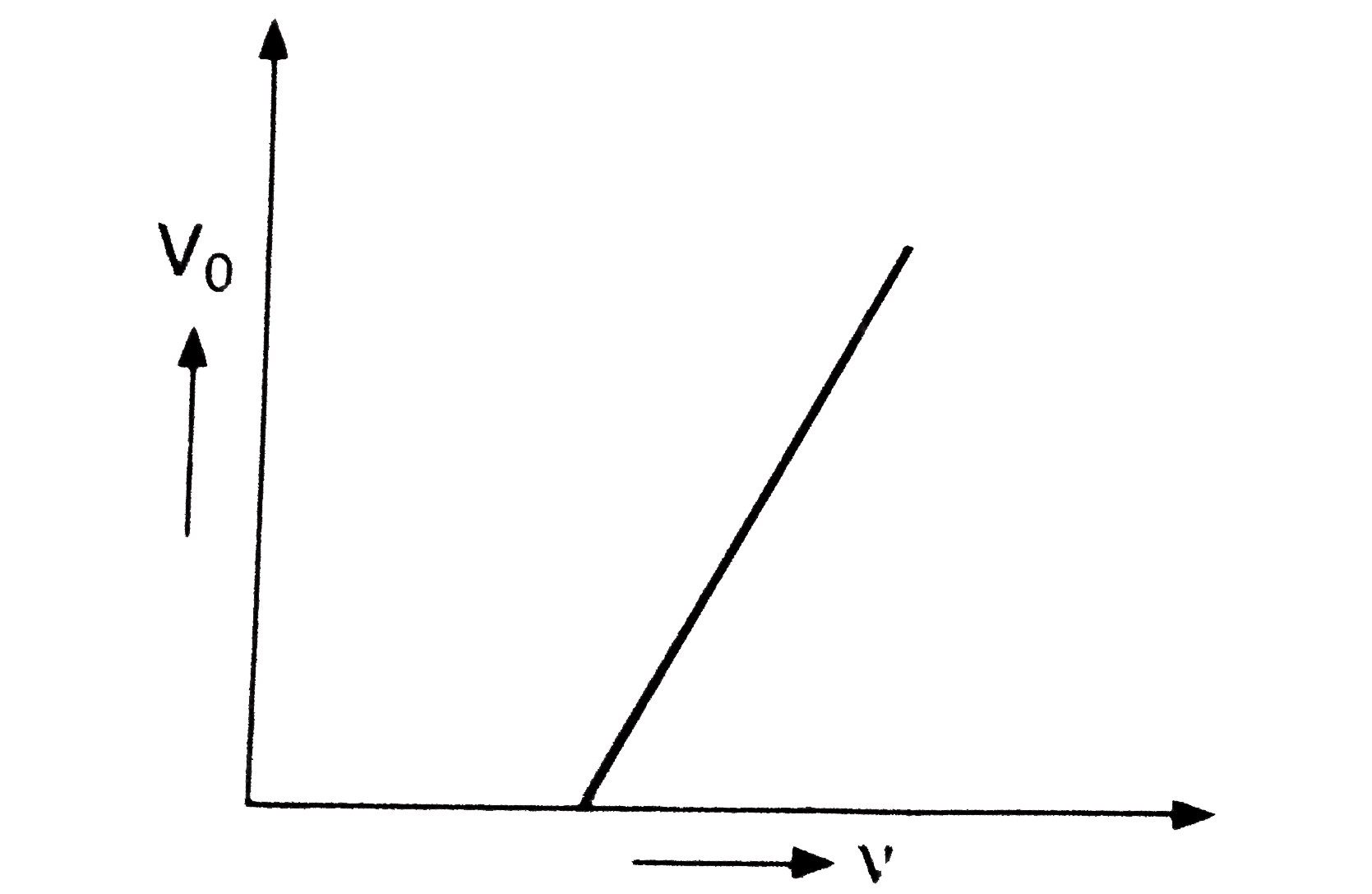
View SolutionIn photoelectric effect the slope of straight line graph between stopping potential (V0) and freqency of incident light (v) gives:
View SolutionIn photoelectric effect the slope of stop of stopping potential versus frequency of incident light for a given surface will be
View Solution
