Find the equivalent resistance of the circuit of the previous problem between the ends of an edge such as a and b in figure.
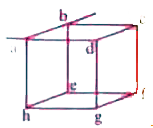
Suppose a current enters the circuit at the point a and the same current leaves the circuit at the point b. The current distribution is shown is figure.
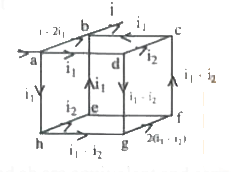
The paths through .ad. and ah are equivalent and carry equal current . The current through ab is then .
The same distribution holds at the junction b. Currents in eb and cb are each. The current in ah is divided into a part in he and in hg. Similar is the division of current in ad into dc and dg. The rest of the currents may be written easily using Kirchhoff.s junction law. The potential difference V between a and b may be written from the paths ab, aheb and ahgfcb as
and
Which may be written as
Eliminating from these equations,
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IA
101 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - IB
44 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL -II PRACTICE SHEET (ADVANCED) (More than One correct answer Type Questions))
2 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
44 Videos
Similar Questions
Find the equivalent resistance of the circuit of the previous problen between the ends of an edge such as a and b in figure.

View SolutionFind the equivalent resistance of the circuit of the previous problen between the ends of an edge such as a and b in figure.

View SolutionFind the equivalent resistance of the circuit between A and B
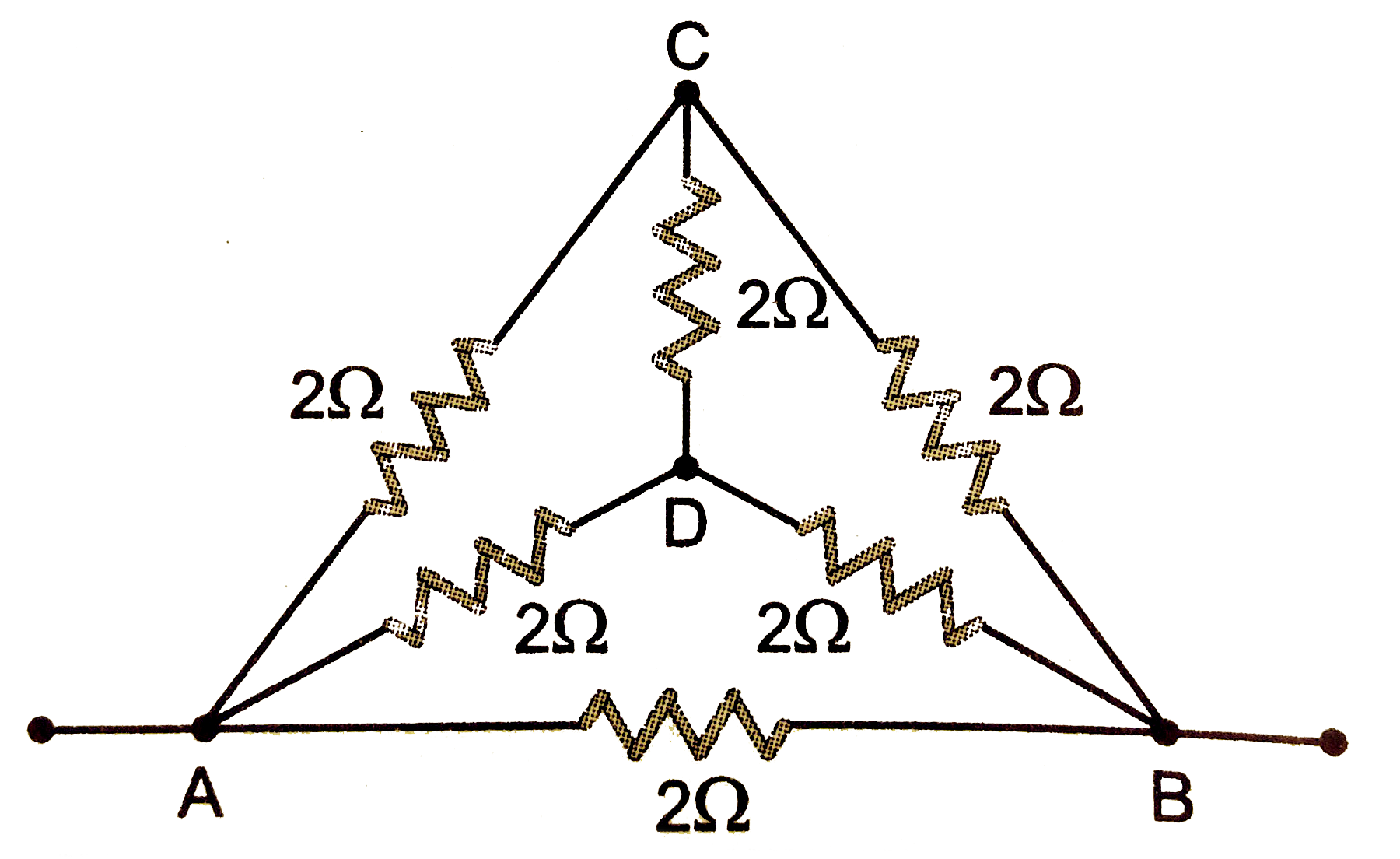
View SolutionFind the equivalent resistance of the circuit between the points A and B

View SolutionCalculate the equivalent resistance of the circuit shown in figure between the points a and b.
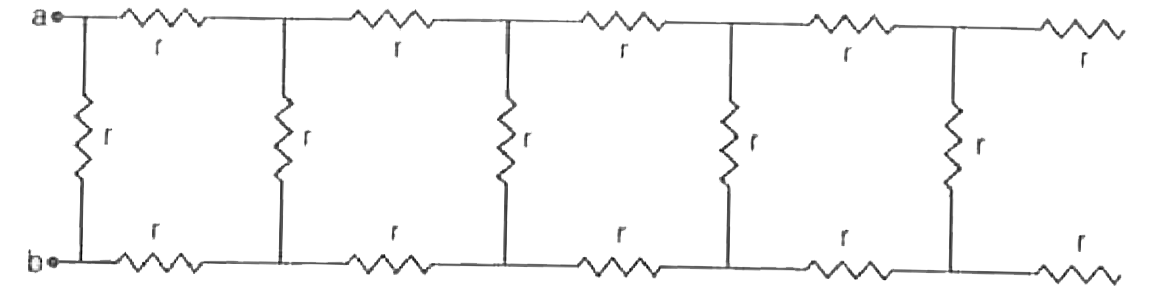
View SolutionFind equivalent resistance of the circuit between the terminals A and B.
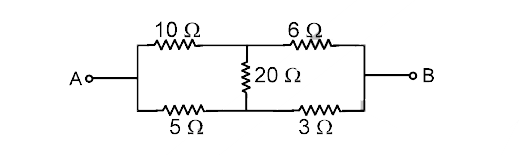
View SolutionFind equivalent resistance of the circuit between the terminals A and B.
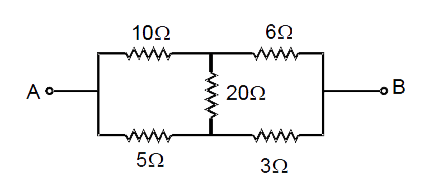
View SolutionFind the equivalent resistance of the circuit shown in figure between the points a and b.Each resistor has a resistances r.

View SolutionFind the equivalent resistance of the circuit shown in figure between the points a and b.Each resistor has a resistances r.

View SolutionFind the equivalent resistance of the circuits shown in figure between the points a and b. Each resistor has a resistance r.
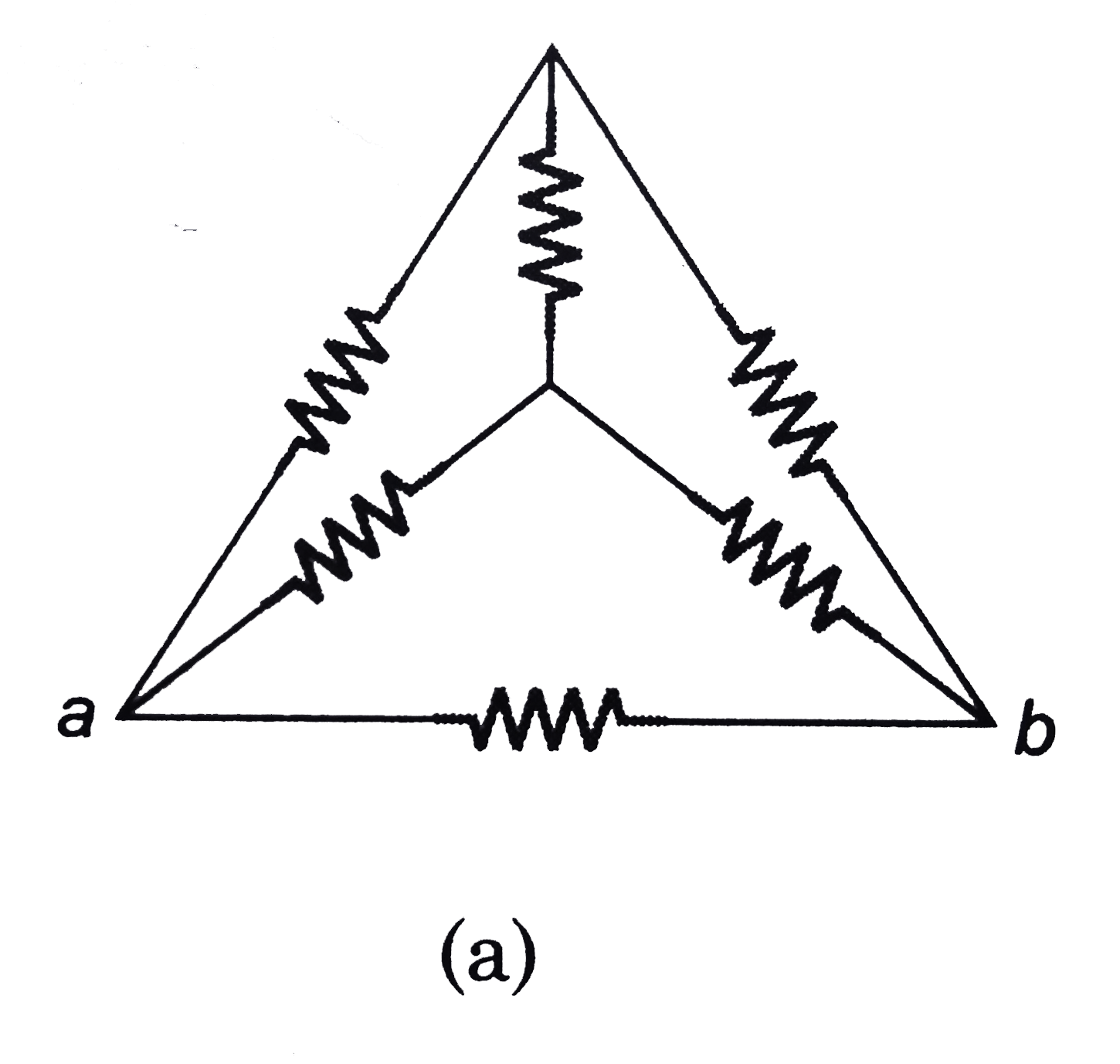

View Solution
