Find the equation of the straight line passing through the points (−1,2) and (5,−1) and also find the area of the triangle formed by it with the axes of coordinates.Solution in Telugu
The correct Answer is:94 sq. units
Step by step video, text & image solution for Find the equation of the straight line passing through the points (-1,2) and (5,-1) and also find the area of the triangle formed by it with the axes of coordinates. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - ADDITIONAL SOLVED EXAMPLES12videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 3.1 (VERY SHORT ANSWER QUESTIONS)11videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II (ADVANCED) PRACTICE SHEET (ADDVANCED)) (Comprehension Type Questions)6videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISEEXERCISE - PROPERTIES OF TRIANGLES57videos
REVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - TANGENT AND NORMAL EXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS48videos
TANGENT AND NORMAL
Similar Questions
Consider the following population and year graph, find the slope of the line AB and using it, find what will be the population in the year 2010?
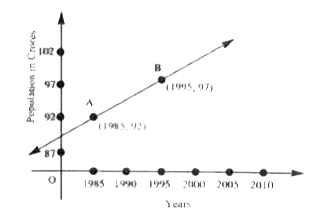 03:23View Solution
03:23View SolutionFind the slope of the line whose inclination is
30∘06:23View SolutionLet A(0,0), B (5,0), C (5,3) and D(0,3) be the vertice of rectiangle ABCD , If P is a variable point lying inside the rectangle ABCD and d (P,L) denotes perpendicular distance of point P from line L
If ≤min{d(P,BC),d(P,CD),d(P,AD)}, then area of the region in which P lies is (in sq. units02:36View SolutionIn a triangle ABC, if A,B, C are in A.P and b:c =√3:√2, then
03:51View SolutionFind the equations of tangent and normal to the curve
(xa)4+(yb)4=2 at (a,b)06:34View SolutionA straight line L is perpendicular to the line 5x−y=1 . The area of the triangle formed by line L, and the coordinate axes is 5. Find the equation of line L.
05:40View SolutionFind the equation of the straight line which passes through the point of intersection of the lines 3x−y=5 and x+3y=1 and makes with the coordinate axes a triangle of area38 sq. units.
View SolutionFind the equation of the straight line passing throught the points (-3,4) and (5,-2) , find also the coordinates of the points where the line cuts the coordinate axes.
03:52View SolutionA straight line passes through the point (2,3) and forms a triangle of area 12 square unit with the coordinate axes . Find the equation of the straight line .
05:17View SolutionFind the equation of the straight line passing through the points (−1,2) and (5,−1) and also find the area of the triangle formed by it with the axes of coordinates.
03:51View SolutionA triangle of area 24 sq. units is formed by a straight line with the coordinate axes in the first quadrant. Find the equation of the straight line, if it passes through (3,4).
06:32View SolutionArea of the triangle formed by the line passing through the points (1,2),(-3,4) with the coordinate axes is
04:37View Solutionएक सरल रेखा L, रेखा 5x−y=1 के लम्बवत है। रेखा L तथा निर्देशांक अक्षों से बनने वाले त्रिभुज का क्षेत्रफल 5 है। रेखा L का समीकरण ज्ञात कीजिए।
03:18View SolutionA straight line xa−yb=1 passes through the point (8,6) and cuts off a triangle of area 12 units from the axes of coordinates . Find the equations of the straight line .
05:40View SolutionA straight line L is perpendicular to the line 5x -y =1 . The area of the triangle formed by the line L and coordinate axes is 5. Find the equation to the line :
05:17View SolutionA straight line L is perpendicular to the line 5x−y=1 . The area of the triangle formed by line L, and the coordinate axes is 5. Find the equation of line L.
03:51View Solution

