Show that the product of the perpendicular from two points to the line
is .Solution in Telugu
The correct Answer is:
Step by step video, text & image solution for Show that the product of the perpendicular from two points (+-sqrt(a^(2)-b^(2)),0) to the line x/a cos alpha+y/b sin alpha=1 is b^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - ADDITIONAL SOLVED EXAMPLES12videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 3.1 (VERY SHORT ANSWER QUESTIONS)11videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II (ADVANCED) PRACTICE SHEET (ADDVANCED)) (Comprehension Type Questions)6videos
STRAIGHT LINES
- BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISEEXERCISE - PROPERTIES OF TRIANGLES57videos
REVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - TANGENT AND NORMAL EXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS48videos
TANGENT AND NORMAL
Similar Questions
Consider the following population and year graph, find the slope of the line AB and using it, find what will be the population in the year 2010?
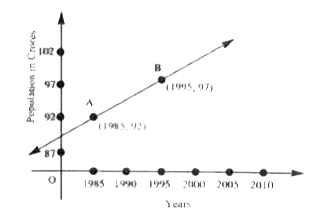 04:53View Solution
04:53View SolutionFind the slope of the line whose inclination is
30∘02:58View SolutionLet A(0,0), B (5,0), C (5,3) and D(0,3) be the vertice of rectiangle ABCD , If P is a variable point lying inside the rectangle ABCD and d (P,L) denotes perpendicular distance of point P from line L
If ≤min{d(P,BC),d(P,CD),d(P,AD)}, then area of the region in which P lies is (in sq. units10:23View SolutionIn a triangle ABC, if A,B, C are in A.P and b:c =√3:√2, then
View SolutionFind the equations of tangent and normal to the curve
(xa)4+(yb)4=2 at (a,b)03:14View SolutionIf (x) / (a) cos alpha + (y) / (b) sin alpha = 1, (x) / (a) cos beta + (y) / (b) sin beta = 1 and (cos alpha cos beta) / (a ^ (2)) + (without alpha without beta) / (b ^ (2)) = 0 then
01:54View SolutionProve that the product of the length of the perpendiculars from the points (√a2−b2,0)and(−√a2−b2,0) to the line xacosθ+yacosθ+ybsinθ=1 is
04:46View Solutionदिखाइए कि (√a2−b2,0) और (−√a2−b2,0) बिन्दुओ से रेखा पxacosθ+ybsinθ=1 पर खींचे गये लम्बो कि लंबाइयाँ का गुणनफल b2 है ।
04:45View SolutionThe product of the perpendiculars drawn from the points ±√a2−b2,0 on the line xacosθ+ybsinθ=1, is
03:36View SolutionThe length of the perpendicular from the origin on the line xsinαb−ycosαa−1=0 is
05:06View SolutionIf the line xcosα+ysinα=p touches the ellipse x2a2+y2b2=1 ,then the point of contact will be
(−a2cosαp,−b2sinαp)
(b2cosαp,a2sinαp)
(b2sinαp,a2cosαp)
(a2cosαp,b2sinαp)06:51View SolutionProve that the product of the lengths of the perpendiculars drawn from the points (√a2−b2,0) and (−√a2−b2,0) to the line xacosθ+ybsinθ=1 is b2
View SolutionProve that the product of the lengths of the perpendiculars drawn from the points (√a2−b2,0) and (−√a2−b2,0) to the line xacosθ+ybsinθ=1 is b2.
03:25View SolutionProve that the product of the lengths of the perpendiculars drawn from the points (√a2−b2,0) and (−√a2−b2,0) to the line xacosθ+ybsinθ=1 is b2.
04:59View SolutionProve that the product of the lengths of the perpendiculars drawn from the points (√a2−b2,0) and (−√a2−b2,0) to the line xacosθ+ybsinθ=1 is b2.
12:23View Solution

