Passage-I :
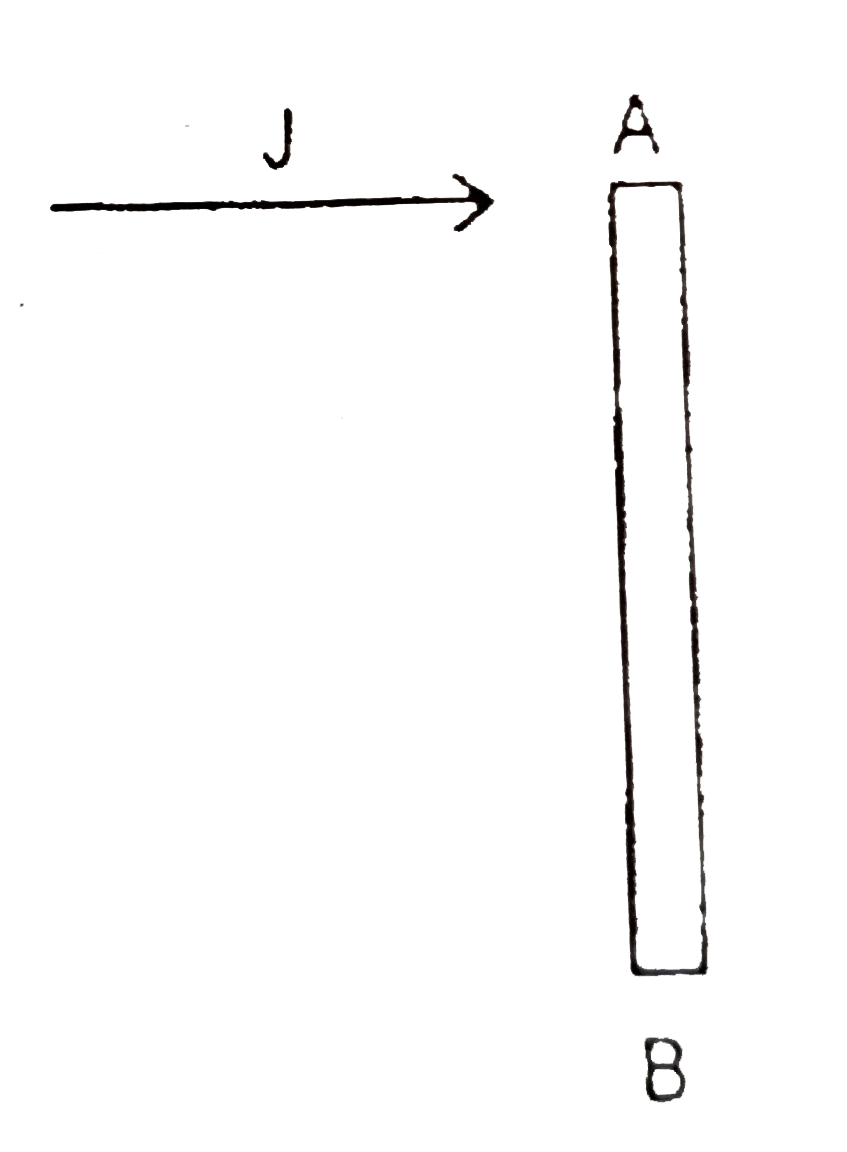
A rod AB length 2m and mass 2kg is lying on a smooth horizontal x-y plane with its centre at origin O as shown in figure. An impulse J of magnitude 10Ns is applied perpendicular to AB at end A.

Find the coordinates of point A of the rod after time t=π/45 of the impact if the centre of mass of the rod is taken as origin at that time.
(√32m,12m)
(√32m,1m)
(34m,32m)
None of these
The correct Answer is:A
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LECTURE SHEET (EXERCISE - VII) Miscellaneous (Integer Type Questions)2videos
ROTATIONAL DYNAMICS
- BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - PRACTICE SHEET (Exercise - I) (LEVEL - I Straight Objective Type Questions)18videos
ROTATIONAL DYNAMICS
- BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LECTURE SHEET (EXERCISE - VII) Miscellaneous (More than One correct answer Type Questions)3videos
ROTATIONAL DYNAMICS
- BOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)6videos
RIVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for Descriptive Answers20videos
ROTATIONAL MECHANICS
Similar Questions
A rod AB of length 2 m and mass 2 kg is lying on a smooth horizontal x-y plane with its centre at origin O as shown in figure. An impulse J of magnitude 10 N-s is applied perpendicular to AB at A.

Co-ordinates of point A of the rod after time t=π45 s will beView SolutionA rod AB of length 2 m and mass 2 kg is lying on a smooth horizontal x-y plane with its centre at origin O as shown in figure. An impulse J of magnitude 10 N-s is applied perpendicular to AB at A.

The distance of point P from centre of the rod which is at rest just after impact isView Solution
Knowledge Check
- Question 1 - Select One
A rod AB of length 2 m and mass 2 kg is lying on a smooth horizontal x-y plane with its centre at origin O as shown in figure. An impulse J of magnitude 10 N-s is applied perpendicular to AB at A.

Co-ordinates of point A of the rod after time t=π45 s will beA[(π9+√32)m,12m]B[34m32m]C[(π6+12)m,12m]D[12m,12m] - Question 2 - Select One
A rod AB of length 2 m and mass 2 kg is lying on a smooth horizontal x-y plane with its centre at origin O as shown in figure. An impulse J of magnitude 10 N-s is applied perpendicular to AB at A.

The distance of point P from centre of the rod which is at rest just after impact isA23 mB13 mC12 mD14m - Question 3 - Select One
A rod AB of mass M and length L lies on smooth horizontal table impulse J is applied to end A as shown in the figure immediately after imparting the impulse :
 AThe radius of curvature of trajectory of A as seen from the ground is 89L.BThe radius of curvature of trajectory of B as seen from the ground is 29L.CThe instantaneous axis of rotation is at a distance of L/6 from the mid point of the rod.Dthe mid point of the rod will move along a straight line.
AThe radius of curvature of trajectory of A as seen from the ground is 89L.BThe radius of curvature of trajectory of B as seen from the ground is 29L.CThe instantaneous axis of rotation is at a distance of L/6 from the mid point of the rod.Dthe mid point of the rod will move along a straight line.
On a smooth horizontal plane, a rod PQ of length 6m and mass 2kg is placed. The centre of the rod is placed at the origin of the coordinate axes. An impulse of 4N−S is applied at P perependicular to the length of the rod. Find the distance of a point form centre of the rod which is at rest after the impact (in metres)
View SolutionA uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View SolutionA uniform rod of mass m and length l is lying on a smooth table. An impulse J acts on the rod momentarily as shown in figure at point R. Just after that:

 View Solution
View SolutionA uniform rod of mass m and length l is lying on a smooth table. An impulse J acts on the rod momentarily as shown in figure at point R. Just after that:

 View Solution
View SolutionA uniform rod of mass m and length l is placed over a smooth horizontal surface along the y-axis and is at rest as shown in Fig. An impulsive force F is applied for a small time △t along x-direction at point A. The x-coordinate of end A of the rod when the rod becomes parallel to x-axis for the first time is [initially, the coordinate of centre of mass of the rod is (0,0)]
 View Solution
View Solution

