The abgular acceleration the toppling pole shown in figure is given , where is te angle between the axis of the pole and the vertical and k is a constant. The pole starts from rest at . Choose the correct options

The tangntial acceleration of the end of the pole is
The centripetal acceleration of the end of the pole is
The tangntial acceleration of the end of the pole is
The centripetal acceleration of the end of the pole is
The correct Answer is:A, B
Topper's Solved these Questions
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LECTURE SHEET (EXERCISE - VII) Miscellaneous (Linked Comprehension Type Questions)
7 Videos
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LECTURE SHEET (EXERCISE - VII) Miscellaneous (Integer Type Questions)
2 Videos
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LECTURE SHEET (EXERCISE - VII) Miscellaneous (Straight Objective Type )
6 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
- ROTATIONAL MECHANICSBOOK - AAKASH SERIESCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for Descriptive Answers
20 Videos
Similar Questions
The angular acceleration of the toppling pole shown in fig is given by a=ksinθ, where θ is the angle between the of the pole and the vertical, and k is aconstant. The pole starts from rest at θ=0. Find (a) the tan gential and (b) the centripetal acceleration of the upper end of the pole in terms of k,θ, and (the length of the pole.)
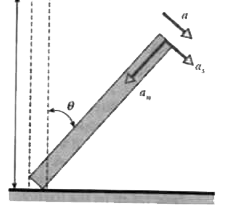
View SolutionThe angular acceleration of a particle moving along a circle is given by a=ksin (theta),where "theta" is the angle turned by particle and k is constant.The centripetal acceleration of the particle in terms of k ,theta and R (radius of circle) is given by
View Solution
Knowledge Check
Three identical conducting rods are connected as shown in figure. Given that θa=40∘C, θc=30∘C and θd=20∘C. Choose the correct option.
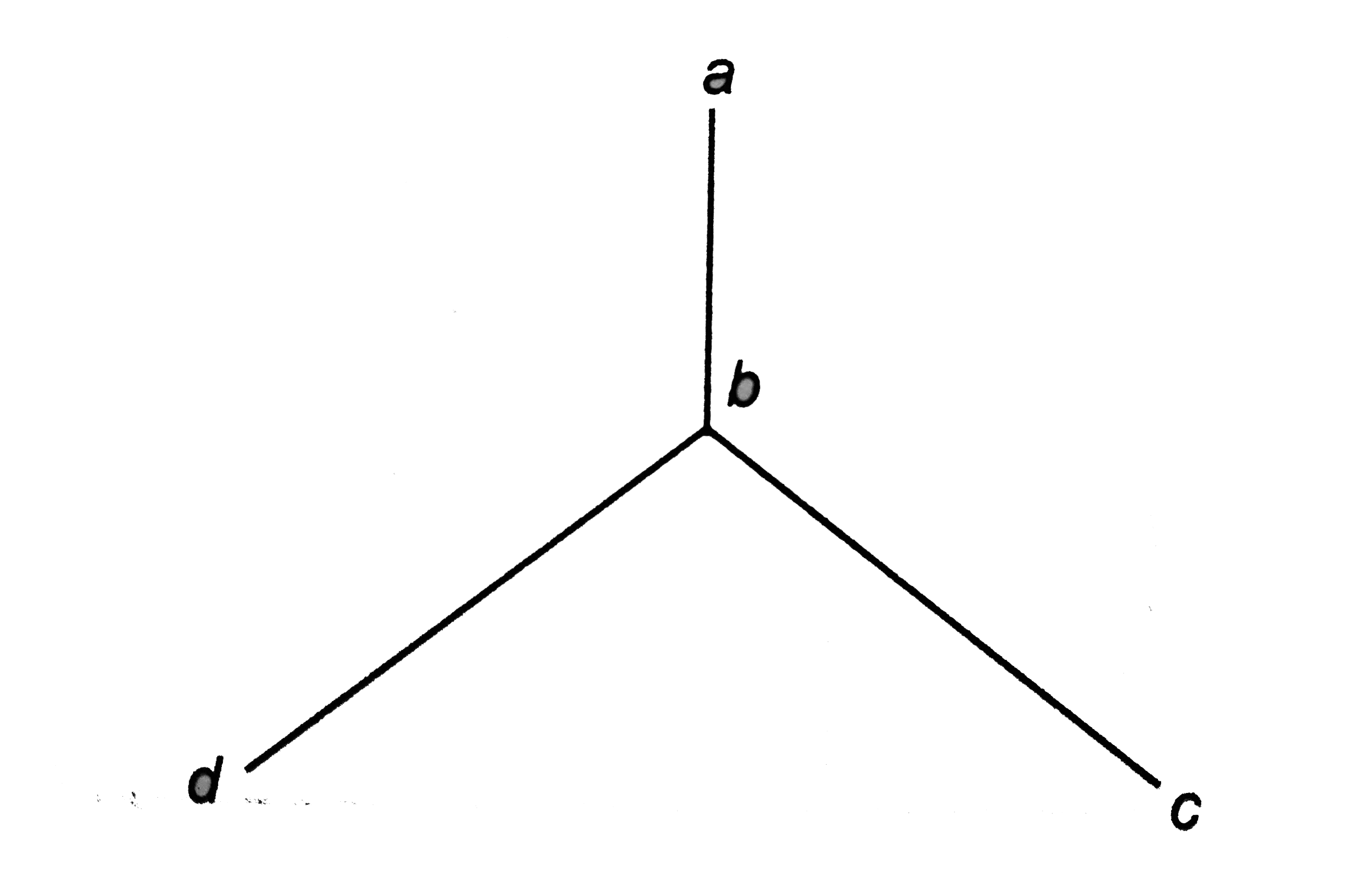 Atemperature of junction b is 15∘C.Btemperature of junction b is 30∘CCheat will flow from b to dDboth (b) and (c)
Atemperature of junction b is 15∘C.Btemperature of junction b is 30∘CCheat will flow from b to dDboth (b) and (c)The ring shown in fig. is given a constant horizontal acceleration (a0=g√3). The maximum deflection of the string from the vertical is θ0. Then
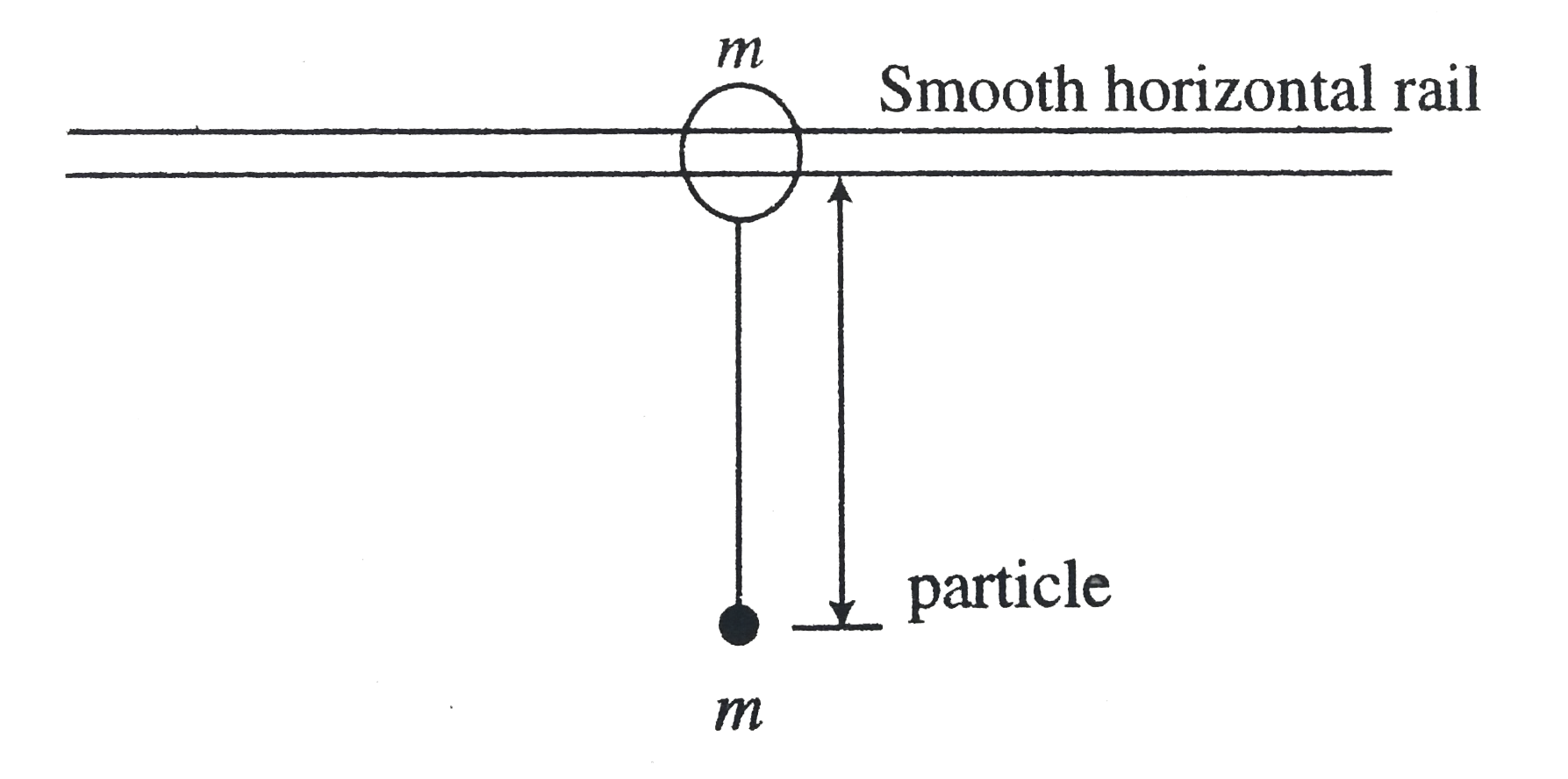 Aθ0=30∘Bθ0=60∘CAt maximum deflection, tension in string is equal to mg.DAt maximum deflection, tension in string is equal to 2mg√3
Aθ0=30∘Bθ0=60∘CAt maximum deflection, tension in string is equal to mg.DAt maximum deflection, tension in string is equal to 2mg√3If the angle θ between Z-axis and the plane 3x−4y+5z+7=0 is cos−1(k),then k=
A12B1√2C2D√2
[" The angular acceleration "],[" of a particle moving along "],[" a circle is given by "a=k],[" sin "theta" ,where "theta" is the angle "],[" turned by particle and "k" is "],[" constant.The centripetal "],[" acceleration of the "],[" particle in terms of "k" ,"theta],[" and "R" (radius of circle) is "],[" given by: "]
View SolutionThree identical conducting rods are connected as shown in figure. Given that θa=40∘C, θc=30∘C and θd=20∘C. Choose the correct options.

View SolutionThe ring shown in fig. is given a constant horizontal acceleration (a0=g√3). The maximum deflection of the string from the vertical is θ0. Then

View SolutionA stationary horizontal disc is free to rotate about its axis. When a torque is applied on it its, kinetic energy as a function of θ, where θ is the angle by which it has rotated, is given as kθ. If its moment of inertia is I then the angular acceleration of the disc is :
View SolutionThe position of paint P is r=a cos θˆi+bsinθˆj, where a and b ar constants and θ is angle between r and x axis . If the rate of increasing of θ Is ω Find the equation of path of particle.
View Solution
