The velocity of river grows in proportion to the distance from it's bank and reaches maximum value V0 in the middle. Near the banks the velocity is zero. A boat is driven perpendicular to river flow, speed of boat in still water is u ( width of river is w)
time taken to cross river wu
time taken to cross river w√V204+u2
Drift is V0w2u
Drift is V0wu
The correct Answer is:A, C
Topper's Solved these Questions
- KINEMATICSBook:AAKASH SERIESChapter:KINEMATICSExercise:PRACTICE SHEET (EXERCISE-VII)(Relative Motion) (LEVEL-II) (Matrix Matching Type Questions)
Explore 1 Video
- KINEMATICSBook:AAKASH SERIESChapter:KINEMATICSExercise:PRACTICE SHEET (EXERCISE-VII)(Relative Motion) (LEVEL-II) (Integer Type Questions)
Explore 4 Videos
- KINEMATICSBook:AAKASH SERIESChapter:KINEMATICSExercise:PRACTICE SHEET (EXERCISE-VII)(Relative Motion) (LEVEL-II) (Straight Objective Type Questions)
Explore 3 Videos
- HORIZONTAL CIRCULAR MOTIONBook:AAKASH SERIESChapter:HORIZONTAL CIRCULAR MOTIONExercise:ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) Integer Type Questions)
Explore 3 Videos
- KINETIC THEORY OF GASESBook:AAKASH SERIESChapter:KINETIC THEORY OF GASESExercise:Practice Exercise
Explore 15 Videos
Similar Questions
The current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View SolutionThe current velocity of river grows in proportion to the distance from its bank and reaches the maximum value v0 in the middle. Near the banks the velocity is zero. A boat is moving along the river in such a manner that the boatman rows his boat always perpendicular to the current. The speed of the boat in still water is u. Find the distance through which the boat crossing the river will be carried away by the current, if the width of the river is c. Also determine the trajectory of the boat.
View Solution
Knowledge Check
A boat crosses a river of width 1 km by shortest path in 15 min. if the speed of boat in still water is 5 kmh−1, then what is the speed of the river?
A5 kmh−1B12 kmh−1C3 kmh−1D4 kmh−1A river of width 80m is flowing at 6 m/s. A motorboat crosses the river in 10s and reaches a point directly across on the other river bank. The velocity of motorboat in still water is :
A8 m/sB10 m/sC6 m/sD5 m/sThe width of a river is 2√3km.A boat is rowed in direction perpendicular to the banks of river.If the drift of the boat due to flow is 2km,the displacement of the boat is.
A3kmB6kmC5kmD4km
The speed of river current close to banks is nearly zero. The current speed increases linearly from the banks to become maximum (=V0) in the middle of the river. A boat has speed ‘u’ in still water. It starts from one bank and crosses the river. Its velocity relative to water is always kept perpendicular to the current. Find the distance through which the boat will get carried away by the current (along the direction of flow) while it crosses the river. Width of the river is l.
View SolutionThe width of a river is 2√3 km. A boat is rowed in direction perpendicular to the banks of river. If the drift of the boat due to flow is 2 km, the displacement of the boat is
View SolutionA river of width 80m is flowing at 6 m/s. A motorboat crosses the river in 10s and reaches a point directly across on the other river bank. The velocity of motorboat in still water is :
View SolutionA man in a boat crosses a river from point A. If he rows perpendicular to the banks he reaches point C (BC=120m) in 10 min. If the man heads at a certain angle α to the straight line AB (AB is perpendicular to the banks) against the current he reaches point B in 12.5 min. Find the width of the river w, the rowing velocity u, the speed of the river current v and the angle α. Assume the velocity of the boat relative to water to be constant and the same magnitude in both cases.
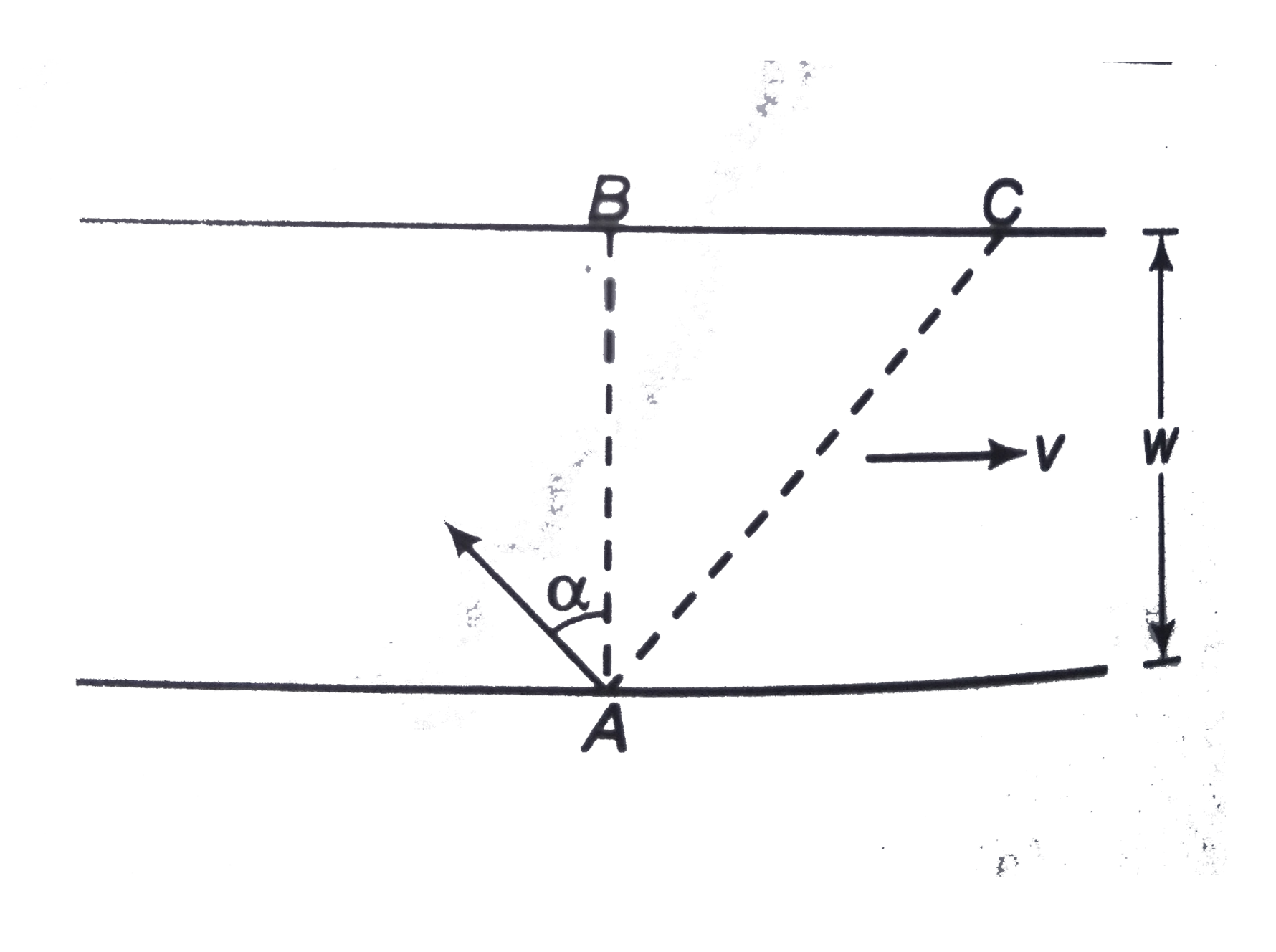
View SolutionA man in a boat crosses a river from point A. If he rows perpendicular to the banks he reaches point C (BC=120m) in 10 min. If the man heads at a certain angle α to the straight line AB (AB is perpendicular to the banks) against the current he reaches point B in 12.5 min. Find the width of the river w, the rowing velocity u, the speed of the river current v and the angle α. Assume the velocity of the boat relative to water to be constant and the same magnitude in both cases.

View Solution
