If a,b,c,d,e,f are in A.P. then d−b= ……Solution in Gujrati
2(c−a)
2(f−c)
2(d−c)
2(f−b)
The correct Answer is:C
Step by step video, text & image solution for If a,b,c,d,e,f are in A.P. then d-b= …… by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SEQUENCE AND SERIESEXERCISE - Textbook based MCQs (Latest Exam MCQs)3videos
SEQUENCE AND SERIES
- BOOK - KUMAR PRAKASHANCHAPTER - SEQUENCE AND SERIESEXERCISE - Testbook Illustrations for Practice Work25videos
SEQUENCE AND SERIES
- BOOK - KUMAR PRAKASHANCHAPTER - SEQUENCE AND SERIESEXERCISE - Miscellaneous Exercise33videos
SEQUENCE AND SERIES
- BOOK - KUMAR PRAKASHANCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Solutions of NCERT Exemplar Problems (Question of Module)16videos
RELATIONS AND FUNCTIONS
- BOOK - KUMAR PRAKASHANCHAPTER - SETSEXERCISE - QUESTION OF MODULE28videos
SETS
Similar Questions
Suppose a1,a2,a3,….,a49 are in A.P and 12Σk=0a4k+1=416anda9+a43=66. If a21+a22+….+a217=140m then m= ……..
02:10View SolutionWrite the first three terms in each of the following sequences defined by the following
an=2n+503:25View SolutionShow that the sum of (m+n)thand(m–n)th terms of an A.P. is equal to twice the mth term.
02:07View SolutionA={−1,0},B={−1,0,1}, then find A×BandB×A
05:38View SolutionA{x∈R∣x2+3=0} is a set of………type.
02:22View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
03:59View SolutionD, E, F are the mid-point of the sides BC, CA and AB respectively of ABC. Then DEF is congruent to triangle. ABC (b) AEF (c) BFD, CDE (d) AFE, BFD, CDE
Given : D, E, F are the mid-point of the sides BC, CA and AB .
02:19View SolutionIn Figure, AB⊥BEandFE⊥BE.If BC=DEandAB=EF, then ABD is congruent to:
(a)EFC
(b) ECF
(c) CEF
(d) FEC
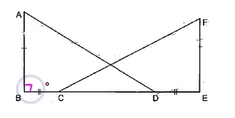04:50View SolutionIf ABC and DEF are two triangles such that AB, BC are respectively equal and parallel to DE, EF, then show that Quadrilateral ABED is a parallelogram Quadrilateral BCFE is a parallelogram AC=DF (iv) ABC ≅ DEF
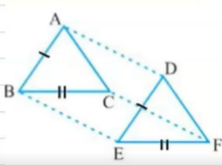02:06View SolutionIf a,b,c,d,e are in H.P., then ab+c+d+e,ba+c+d+e,ca+b+d+e,da+b+c+e,ea+b+c+d are in (A) A.P. (B) G.P. (C) H.P. (D) none of these
04:58View SolutionIn Figure, DE∣∣AC and DF∣∣AE . Prove that EFBF=ECBE .
03:05View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=34 , then write Area (ABC):Area(DEF) .
02:18View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=25 , then Area(ABC):Area(DEF)=
02:54View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
04:19View SolutionD, E, F are the mid-point of the sides BC, CA and AB respectively of △ABC. Then DEF is congruent to triangle.
(a)ABC
(b) AEF
(c) BFD, CDE
(d) AFE, BFD, CDE02:45View Solution