An aeroplane, when 3000 m high, passes vertically above another aeroplane at an instant when the angles of elevation of the two aeroplanes from the same point on the ground are 60∘ and 45∘ respectively. Find the vertical distance between the two aeroplanes.Solution in Punjabi
Step by step video & image solution for An aeroplane, when 3000 m high, passes vertically above another aeroplane at an instant when the angles of elevation of the two aeroplanes from the same point on the ground are 60^@ and 45^@ respectively. Find the vertical distance between the two aeroplanes. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
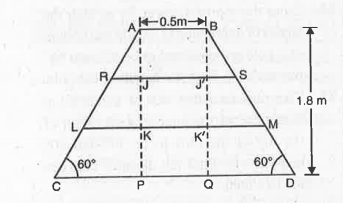
In the given figure, ABCD is a trapezium in which ABIICD. Line-segments RS and LM are drawn parallel to AB such that AJ = JK = KP. If AB = 0.5 m and AP = BQ = 1.8 m, find the length of AC, BD, RS and LM.
View SolutionUse Euclid's division algorithm to find HCF of : 36,84
View SolutionIn a city, the following weekly observations were made in a study of cost of living index for the year 1980-81.
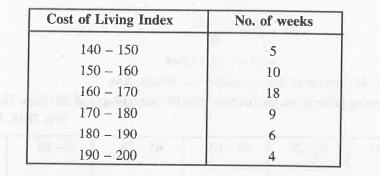 Calculate the mean weekly cost of living index by a suitable method.
Calculate the mean weekly cost of living index by a suitable method.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 600&450 respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionAn aeroplane when 3500m high passes vertically above another aeroplane at an instant when the angles of elevation of the two aeroplanes from the same point on the ground are 45 and 30 respectively.Find the vertical distance 35. between the two aeroplanes.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 60o and 45o respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionAn aeroplane, when 3000 m high, passes vertically above anthoer aeroplane at an instant when the angles of elevation of the two planes from the same point on the ground are 45∘ and 60∘ respectively. Find the vertical distance between the tow planes.
View SolutionAn aeroplane flying at a height of 3000 m above the ground passes vertically another place at an instant when the angle of elevation of the two planes from the same point on the ground are 60∘and45∘ respectively. The height of the lower plane from the ground is :
View SolutionAn aeroplane when 3000 m high passes vertically above another aeroplane at an instant when their angles of elevation at the same observing point are 60∘ and 45∘ respectively. How many metres higher is the one than the other?
View SolutionThe angles of elevation of an aeroplane flying vertically above the ground,as observed from the two consecutive stones,I km apart, are 45∘ and60∘aeroplane from the ground .The height of the aeroplane above the ground in km is: ]
View SolutionThe angles of elevation of an aeroplane flying vertically above the ground as observed from two consecutive milestones 1 km apart are 45∘ and 60∘. The height of the aeroplane from the ground is
View SolutionThe angle of elevation of an aeroplane from a point on the ground is 60∘. After a flight of 30 seconds the angle of elevation becomes 30∘. If the aeroplane is flying at a constant height of 3000 √3 m. Find the speed of the aeroplane.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 600&450 respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 60∘ and 45∘ respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionThe angle of elevation of an aeroplane from a point on the ground is 45∘ . After a flight of 15 seconds, the elevation changes to 30∘ . If the aeroplane is flying at a height of 3000 metres, find the speed of the aeroplane.
View Solution