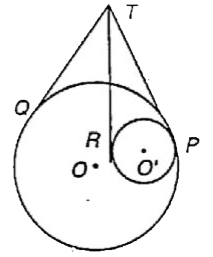
Two circles touch externally at a point P. From a point T on the tangent at P, tangents TQ and TR are drawn to the circles with points of contact Q and R respectively. Prove that TQ = TRSolution in Punjabi
Step by step video & image solution for Two circles touch externally at a point P. From a point T on the tangent at P, tangents TQ and TR are drawn to the circles with points of contact Q and R respectively. Prove that TQ = TR by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Fill in blanks-
A secant drawn to a circle intersect the circle in ____points.
View SolutionComplete the prime factor tree :
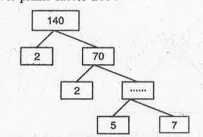
View SolutionGive the justification of the construction also. Draw a line segment of length 7.6 cm and divide it in the ratio 5 : 8. Measure the two parts.
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T . Prove that ∠PTQ=2∠OPQ .
View SolutionTwo circles touch externally at a point P . From a point T on the tangent at P , tangents TQ and TR are drawn to the circles with points of contact Q and R respectively. Prove that TQ=TR . (FIGURE)
View SolutionTP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. Prove that (TP')/(TP)+(TQ')/(TQ)=1
View Solutionदो वृत्त जिंसके केंन्द्र O और O' है, एक -दूसरे को अन्तः बिन्दु P पर स्पर्श करते है। उभयनिष्ट स्पर्श रेखा PT के बिन्दु T से वृत्तों पर रेखाएँ क्रमशः TQ और TR खींची जाती है। सिद्ध कीजिए कि
रेखाखण्ड TR = रेखाखण्ड TQ.
View SolutionO केंन्द्र वाले वृत्त के बाहर स्थित बिन्दु T से वृत्त पर दो स्पर्श रेखाएँ TP और TQ खींची गई है, जो वृत्त को P और Q बिन्दुओं पर स्पर्श करती है, तो सिद्ध कीजिए कि ∠PTQ=2∠OPQ.
View Solutionबिंदु T से वृत्त C(O,r) पर दो स्पर्श रेखाएँ TP और TQ खींची गई हैं । सिद्ध करो कि ∠PTQ=2∠OPQ
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T . Prove that ∠PTQ=2∠OPQ
View SolutionIn the given figure, there are two circles with the centres O and O' touching each other internally at P. Q Tangents TQ and TP are drawn to the larger circle and tangents TP and TR are drawn to the smaller circle. Find TQ:TR :
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ⌊PTQ=2⌊OPQ.
View SolutionTwo circles touch externally at a point P. From a external point T on the tangent at P, tangents TQ and TR are drawn to the circles with points of contact Q and R respectively. Prove that TQ=TR.
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ=2∠OPQ.
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ=2∠OPQ.
View Solution
