A block of mass m is arranged on the wedge as shown in figure . The wedge angle is θ. If the masses of pulley and thread are negligible and friction is absent , find the acceleration of the wedge .
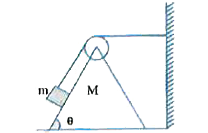
It is obvious that when block m moves downward along the incline of wedge, the wedge moves to the right . As the length of thread is constant, the distance traversed by wedge along the incline is equal to distance traversed by wedge to the right . This implies that acceleration of wedge to the right is equal to downward acceleration of wedge .
Let a be the acceleration of wedge to the right .
Then the force acting on the block m are
(i) weight mg acting vertically downward
(ii) normal reaction R1
(iii)tension T (up the incline )
(iv)Fictitious force ma to the left .
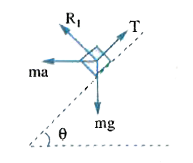
The free body diagram of mass m is shoen in figure .
For motion of block .m. on inclined plane
mgsinθ+macosθ−T=ma ....(1)
As mass .m. does not breaks off the inclined plane , therefore for forces on .m. normal to inclined plane
R1+masinθ=mgcosθ ....(2)
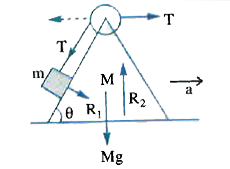
The forces acting on the wedge are
(i) weight Mg downward
(ii) normal reactionof ground on wedge =R2
(iii) normal reaction of block on wedge =R1
(iv) tension (T,T) in string .
The free body diagram of wedge is shown in figure .
For motion of wedge in horizontal direction
R1sinθ+T−Tcosθ=mg ....(4)
From (1),
T=mgsinθ+macosθ−ma ....(5)
From (2), R1=mgcosθ−masinθ ....(6)
substituting these values in (3) , we get
(mgcosθ−masinθ)sinθ+(mgsinθ+macosθ−ma)(1−cosθ)=Ma
or {M+msin2θ+m(1−cosθ)2}a
=mgcosθsinθ+mgsinθ(1−cosθ)
a=mgsinθM+msin2θ+m(1−2cosθ+cos2θ)=mgsinθM+m(sin2θ+1−2cosθ+cos2θ)
∴a=mgsinθM+2m(1−cosθ)
Similar Questions
A block of mass m is arranged on the wedge as shown in figure . The wedge angle is θ. If the masses of pulley and thread and negligible and friction is absent , find the acceleration of the wedge
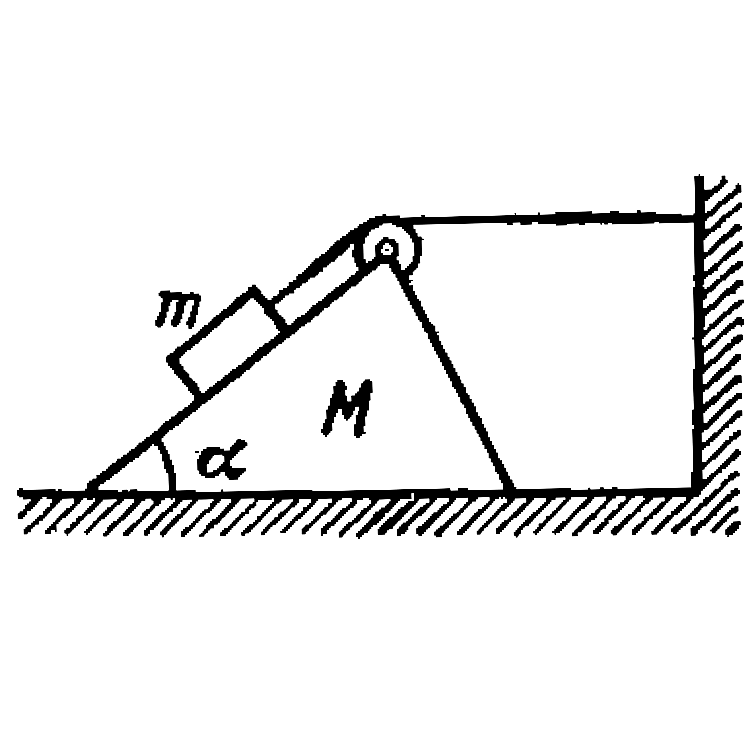
View SolutionIn the arrangement shown in figure the masses m of the bar and M of the wedge, as well as the wedge angle α, are known. The masses of the pulley and the thread are negligible. The friction is absent. Find the acceleration of the wedge M.
View SolutionA block of mass m is at rest on a rought wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rought wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

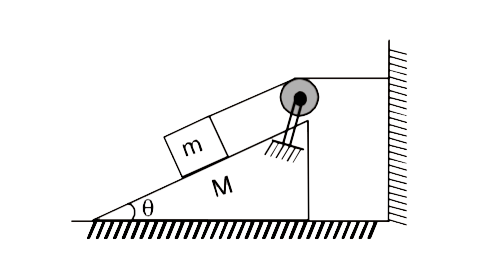
View SolutionFor the arrangement shown in figure when the system is released, find the acceleration of wedge. Pulley and string are ideal and friction is absent.
View SolutionA block of mass m is released from a wedge of mass m as shown in figure . Find the time taken by the block to reach the end of the wedge.
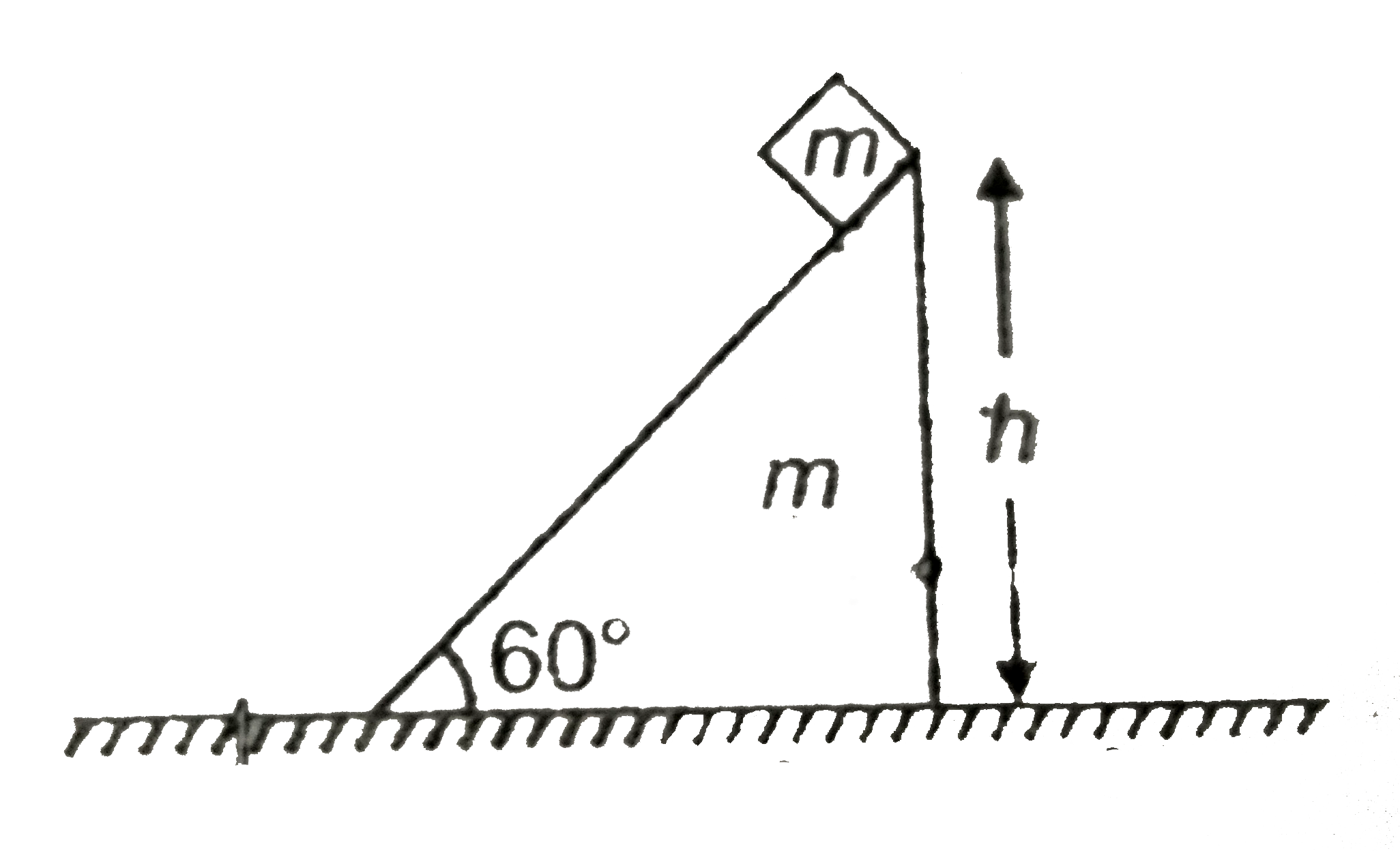
View SolutionA block of mass m is released from a wedge of mass m as shown in figure . Find the time taken by the block to reach the end of the wedge.

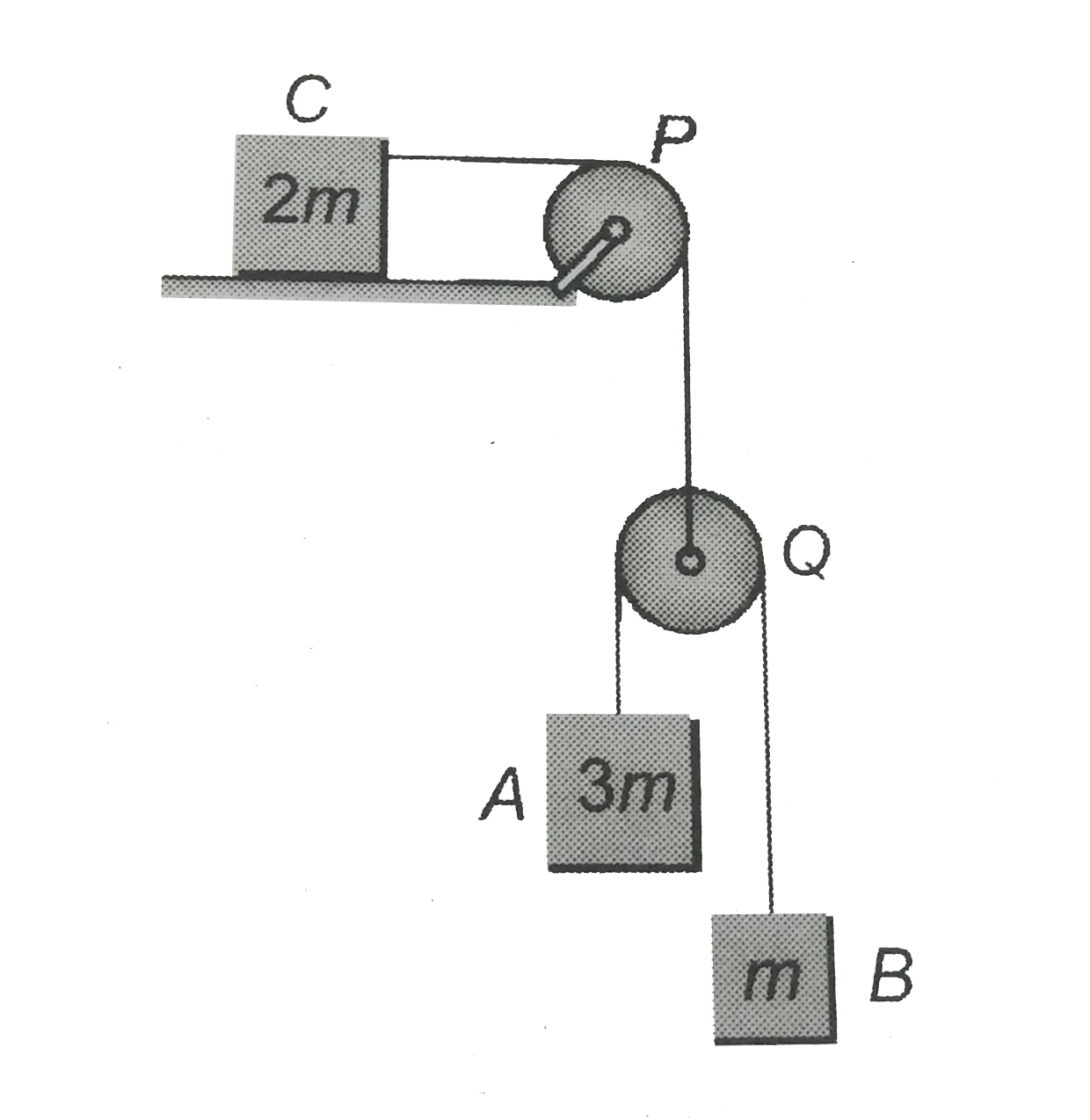
View SolutionIn the arrangement shown in the figure, the blocks have masses 3m,m and 2m, the friction is absent, the masses of the pulleys and the threads are negligible. Find the acceleration of mass 3m.
View Solution
