In the pulley system shown in figure . P and Q are fixed pulleys while A, B and C are movable pulleys each of mass 1kg . The strings are vertical and inextensible . Find the tension in the string and acceleration of frictionless pulleys A,B and C
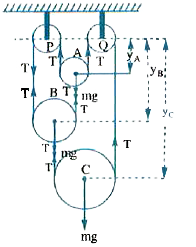
A single string whose ends are tied to centres of A and B, passes over all the pulleys, so tension at each point of string is same equal to T Weight of each pulley A,B andC is
mg=1gNewton.
Let yA,yB and y_(C)bethedistanceofcentresofp≤––––ysA,BandComfixedp≤––––ysatanytimet.<br>Follow∈gthestr∈g⋆t∈gomendAandreaχngup→endB,wehave<br>(y_(B)-y_(A))+y_(B) +2y_(A)+y_(C)+y_(C)-y_(B)=L=constant<br>D⇔erentitat∈gtwicewithrespect→t,we≥t<br>(d^(2)y_(A))/(dt^(2))+(d^(2)y_(B))/(dt^(2))+2(d^(2)y_(C))/(dt^(2))=0<br>i.e.,a_(A)+a_(B)+2a_(C)=0<br>wherea_(A),a_(B),anda_(C)areae≤rationofp≤––––ysA,B,Crespectively.<br>Nowequationsofmotionofp≤––––ysA,BandCare<br>mg+T-2T=ma_(A) rArr mg-T=ma_(A) ....(2)<br>mg+T-2T=ma_(B) rArr mg-T=ma_(B)....(3)and<br>mg-2T=ma_(C)....(4)<br>From(2)and(3)itisobvioustˆ<br>anda_(A)=a_(B) =(g-T/M) .....(5)andom(4),a_(C) =g-(2T)/m.....(6)<br>⊂stitut∈ga_(A),a_(B)anda_(C) ∈(1),we≥t<br>(g−Tm)+(g−Tm)+2(g−2Tm)=0
4g−6Tm=0⇒T=23mg=23×1×9.8=6.5N
∴aA=aB=(g−Tm)=9.8−6.51=3.3m/s2
From (1), ac=−aA=−3.3m/s2
Similar Questions
In the pulley system shown the movable pulleys A,B and C have mass m each, D and E are fixed pulleys. The strings are vertical light and inextensible Then
 .
.
View SolutionIn the pulley system shown in figure the movable pulleys A,B and C are of mass 1 kg each D and E are fixed pulleys are light and inexensible.
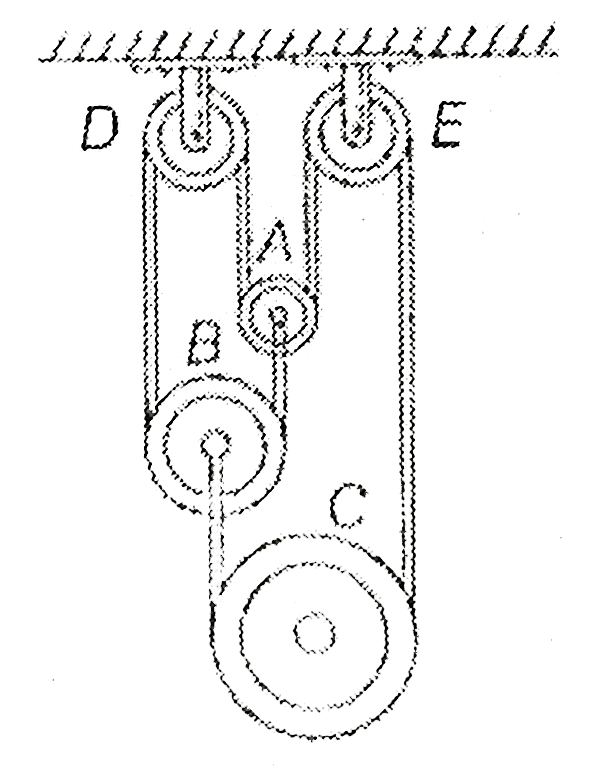
Choose the correct alternative (s).
View Solution
Knowledge Check
In the pulley system shown the movable pulleys A,B and C have mass m each, D and E are fixed pulleys. The strings are vertical light and inextensible Then
 .Athe tension throughout the string is the same and equals T=2mg3 .Bpulleys A and B have acceleration g3 each in downward direaction and pulley C has acceleration g3 in wpward direaction .Cpulleys A,B and C all have accleration g3 in downward direction .Dpulley A has acceleration g3 in downward direction and pulleys B and C have acceleration g3 each in upward direction .
.Athe tension throughout the string is the same and equals T=2mg3 .Bpulleys A and B have acceleration g3 each in downward direaction and pulley C has acceleration g3 in wpward direaction .Cpulleys A,B and C all have accleration g3 in downward direction .Dpulley A has acceleration g3 in downward direction and pulleys B and C have acceleration g3 each in upward direction .In the pulley system shown in fifure the movable pullet=ys A,B and C are of mass 1 kg each D and E are fixed pulleys are light and inexensible.
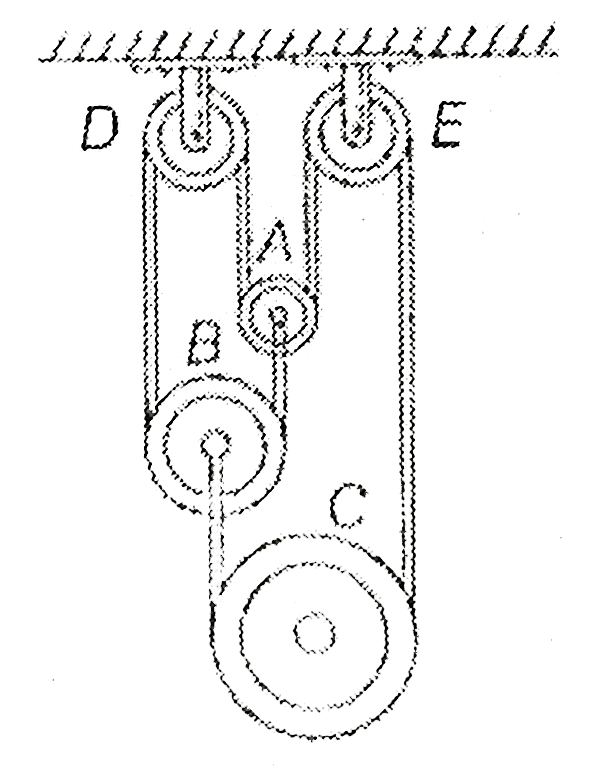
Choose the correct alternative (s).ATension in the string is 6.5 NBAcceleration of pulley A is g/3 downwardCAcceleration of pulley B is g.\6 upwardDAcceleration of pulley C is g/3 upwardAssume that all the pulleys are massless and frictionless and strings to be inextensible.
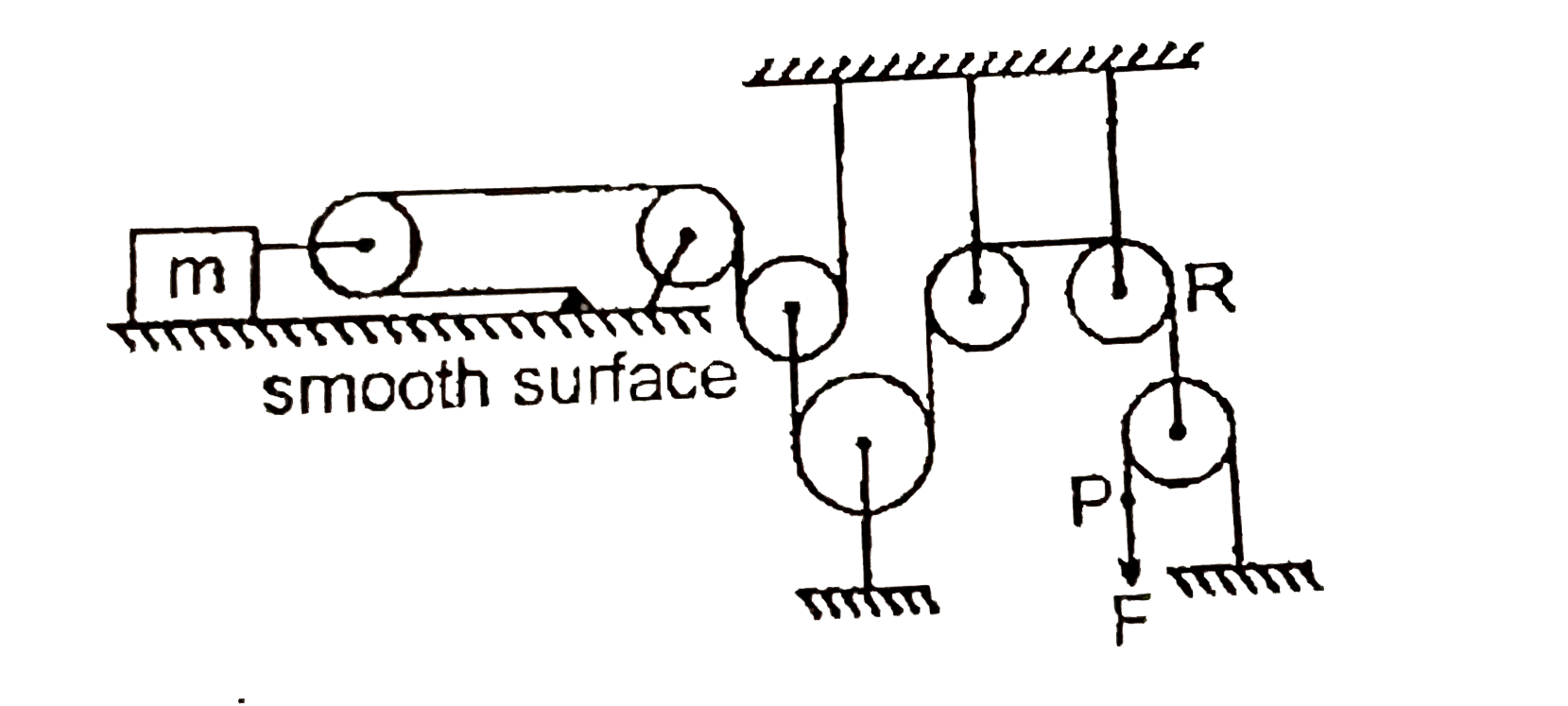 AAcceleration of P is 4 F/mBAcceleration of m is F/mCAcceleration of P is 2F/mDAcceleration of m is F/2m
AAcceleration of P is 4 F/mBAcceleration of m is F/mCAcceleration of P is 2F/mDAcceleration of m is F/2m
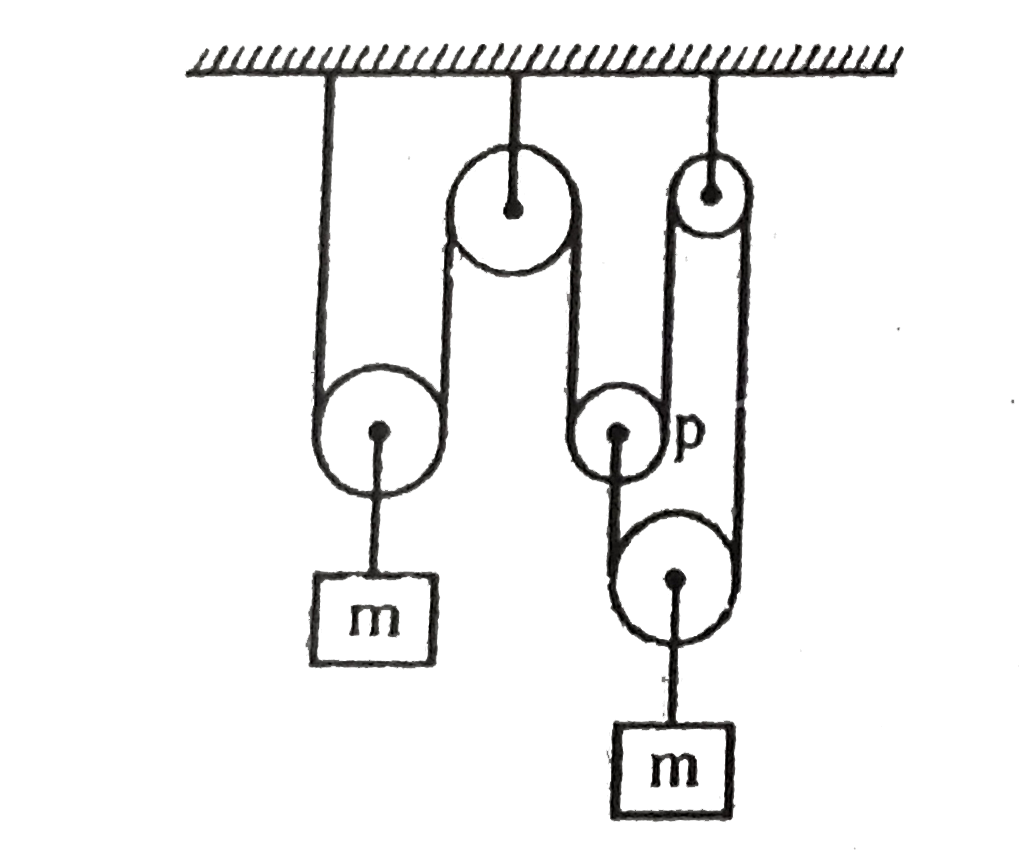
All pulleys are massless The string is light and inextensible Find acceleration of centre of pulley 'p'∈m/s2
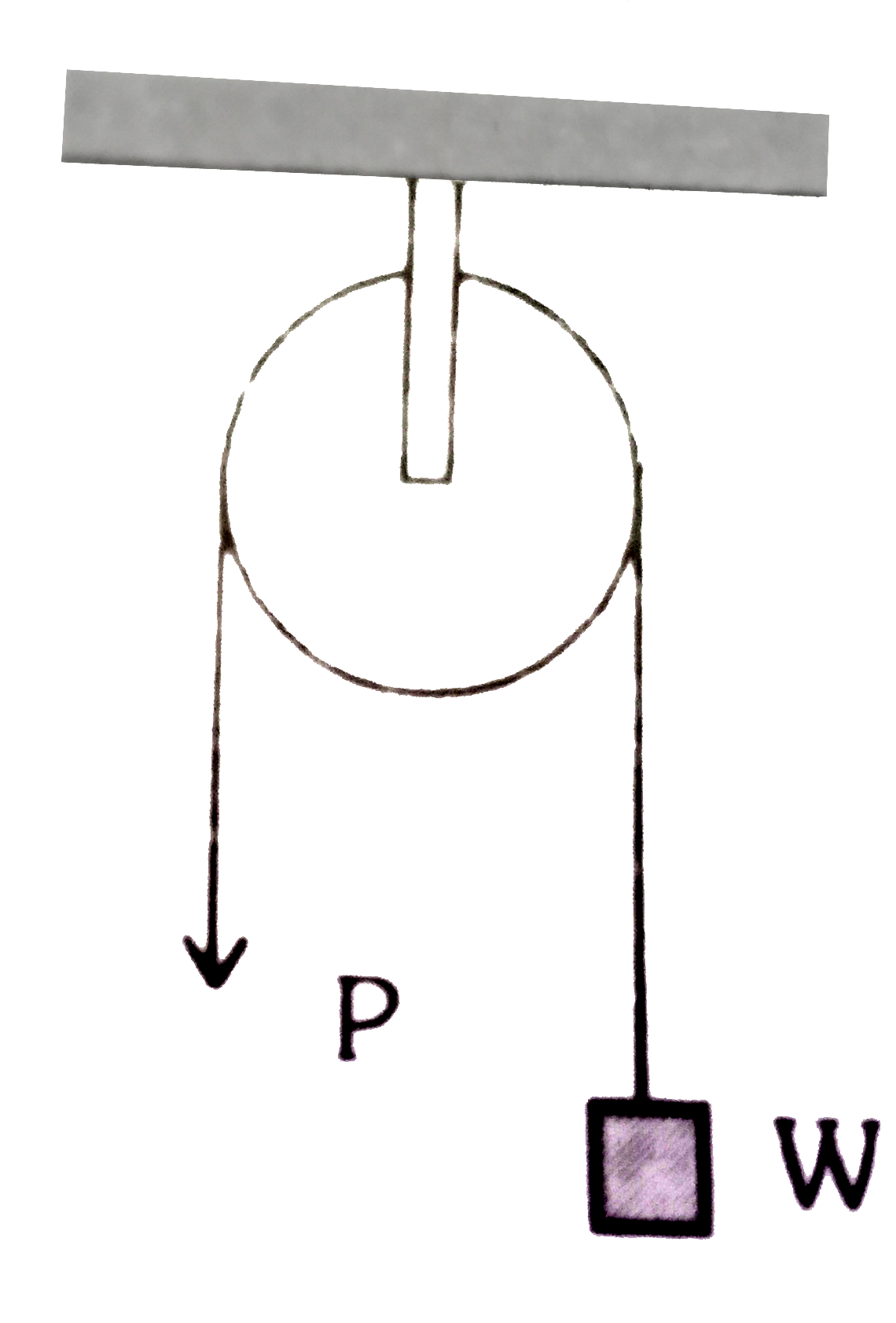
View SolutionWhat is the mechanical advantage of single fixed pulley:-
View SolutionFind the acceleration of two masses as shown in figure . The pulley are light and frictionless and string are light and frictionless and string are light and inextensible.
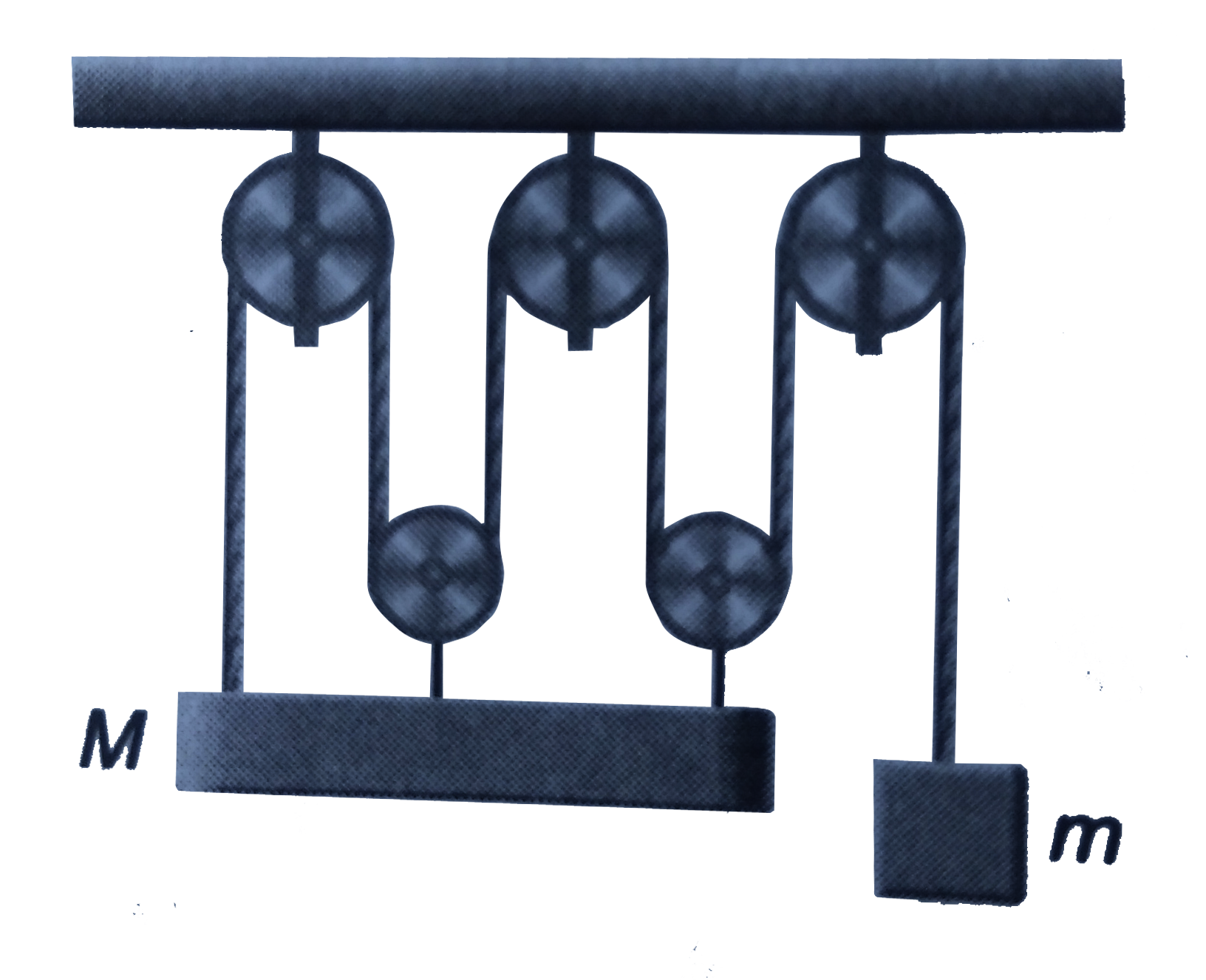
View SolutionFind the acceleration of two masses as shown in figure . The pulley are light and frictionless and string are light and frictionless and string are light and inextensible.

View SolutionConsider4 the situation shown in figure. Bothe the pulleys and the string are light and all the surfaces are friction less. a. Find the acceleration of the mass M. b. Find the tension in the string c. Calculate the force exerted by the clamp on the pulley A in the figure.

View Solution
