In three dimensions there may be more than one point, which are equidistant from three given noncolliner points A,B,C. One of these points will be circumcentre of the triangle ABC
y coordinate of the circumcentre of triangle ABC must be ac
A
aca+b+c
B
a2c2−b4a3+b3+c3
C
(b2c2+a2b2−a2c2a2b2+b2c2+c2a2)
D
b3(a2+c2)2(b2c2+a2c2+a2b2)
Text Solution
The correct Answer is:c
|
Topper's Solved these Questions
- 3D COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 3D COORDINATE SYSTEMEXERCISE - LECTURE SHEET (EXERCISE-I) (Integer Type Questions)
3 Videos
- 3D COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 3D COORDINATE SYSTEMEXERCISE - PRACTICE SHEET (EXERCISE-I) (LEVEL-I) (Straight Objective Type Questions)
22 Videos
- 3D COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 3D COORDINATE SYSTEMEXERCISE - LECTURE SHEET (EXERCISE-I) (Straight Objective Type Questions) (Matrix Matching Type Questions)
1 Videos
- 2D-COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 2D-COORDINATE SYSTEMEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
14 Videos
- 3D-COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 3D-COORDINATE SYSTEMEXERCISE - PRACTICE EXERCISE
40 Videos
Similar Questions
Knowledge Check
In three dimensions there may be more than one point, which are equidistant from three given non-collinear points A, B, C. One of these points will be circumcentre of the triangle ABC.
The y-coordinate of the circumcentre of triangle ABC must beAaca+b+cBa2c2−b4a3+b3+c3Cb2c2+a2b2−a2c2a2b2+b2c2+c2a2.bDnone of theseIn three dimensions there may be more than one point, which are equidistant from three given non-collinear points A, B, C. One of these points will be circumcentre of the triangle ABC.
The y-coordinate of orthocenter of the triangle ABC.A3a2c2−a2b2−b2c2a2b2+b2c2+c2a2.bBab+b2−aca+b+cCb−2(a2c2+b4)a3+b3+c3Dnone of theseThe coordinates of the point which is equidistant from the three vertices of the △AOB as shown in the figure is
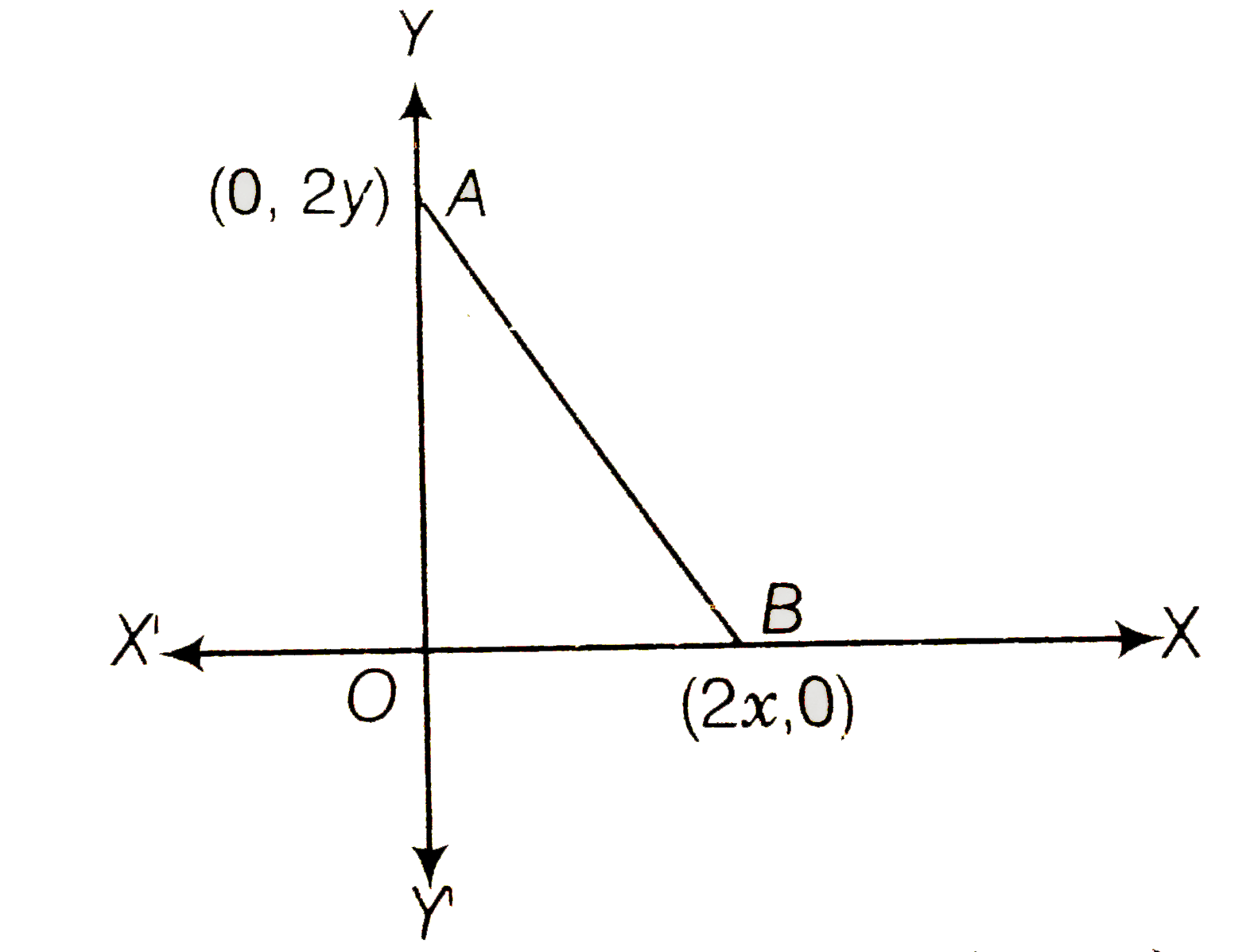 A(x,y)B(y,x)C(x2,y2)D(y2,x2)
A(x,y)B(y,x)C(x2,y2)D(y2,x2)
The equations of three sides of a triangle are x=5,y−2−0andx+y=9. The coordinates of the circumcentre of the triangle are
View SolutionThe equations of the three sides of a triangle are x=2,y+1=0 and x+2y=4 .The coordinates of the circumcentre of the triangle are
View SolutionFind the coordinates of the point equidistant from three given points A(5, 3), B(5, -5) and C(1, -5)
View SolutionFind the coordinates of the point equidistant from three given points A(5,1),B(−3,−7) and C(7,−1).
View SolutionThe coordinates of the point which is equidistant from the three vertices of the △AOB as shown in the figure is

View Solution
