Two identical rings are moving with equal kinetic energy One ring rolls without slipping and other ring is in pure translational motion. The ratio of their respective speeds of centre of mass is
1:√2
√2:√3
1:√3
1:1
The correct Answer is:C
To solve the problem, we need to analyze the kinetic energies of the two identical rings, one rolling without slipping and the other moving with pure translational motion.
1. Identify the Kinetic Energy of Each Ring:
- For the ring that rolls without slipping, the total kinetic energy (KE) can be expressed as:
KErolling=12mv21+12Iω2
where I is the moment of inertia and ω is the angular velocity. For a ring, I=mr2 and ω=v1r.
- Therefore, substituting I and ω:
KErolling=12mv21+12(mr2)(v1r)2
Simplifying this gives:
KErolling=12mv21+12mv21=mv21
2. Kinetic Energy of the Translating Ring:
- For the ring that is in pure translational motion, the kinetic energy is:
KEtranslational=12mv22
3. Setting the Kinetic Energies Equal:
- Since both rings have equal kinetic energies, we set:
mv21=12mv22
- We can cancel m from both sides (assuming m≠0):
v21=12v22
4. Solving for the Ratio of Speeds:
- Taking the square root of both sides gives:
v1=v2√2
- Therefore, the ratio of their respective speeds of the center of mass is:
v1v2=1√2
Final Answer:
The ratio of their respective speeds of center of mass is 1√2.
- For the ring that rolls without slipping, the total kinetic energy (KE) can be expressed as:
KErolling=12mv21+12Iω2
where I is the moment of inertia and ω is the angular velocity. For a ring, I=mr2 and ω=v1r.
- Therefore, substituting I and ω:
KErolling=12mv21+12(mr2)(v1r)2
Simplifying this gives:
KErolling=12mv21+12mv21=mv21
- For the ring that is in pure translational motion, the kinetic energy is:
KEtranslational=12mv22
- Since both rings have equal kinetic energies, we set:
mv21=12mv22
- We can cancel m from both sides (assuming m≠0):
v21=12v22
- Taking the square root of both sides gives:
v1=v2√2
- Therefore, the ratio of their respective speeds of the center of mass is:
v1v2=1√2
The ratio of their respective speeds of center of mass is 1√2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two identical discs are moving with the same kinetic energy. One rolls and the other slides. The ratio of their speeds is:
A1:1B√2:√3C2:3D1:2A cylindrical ring is rolling without slipping. The ration of rotational and translational kinetic energies is
A0.25B0.5C1D1.5When a sphere rolls without slipping the ratio of its kinetic energy of translation to its total kinetic energy is.
A1:7B1:2C1:1D5:7
Two bodies a ring and a solid cylinder of same material are rolling down without slipping an inclined plane. The radii of the bodies are same. The ratio of velocity of the centre of mass of the bottom of the inclined plane of the ring to that of the cylinder is √x2 . Then, the value of x is ……………….
View SolutionIn the given figure a ring of mass m is kept on a horizontal surface while a body of equal mas 'm' attached through a string Which is wounded on the ring when the system is released the ring rolls without slipping consider the following statements and choose the correct option.
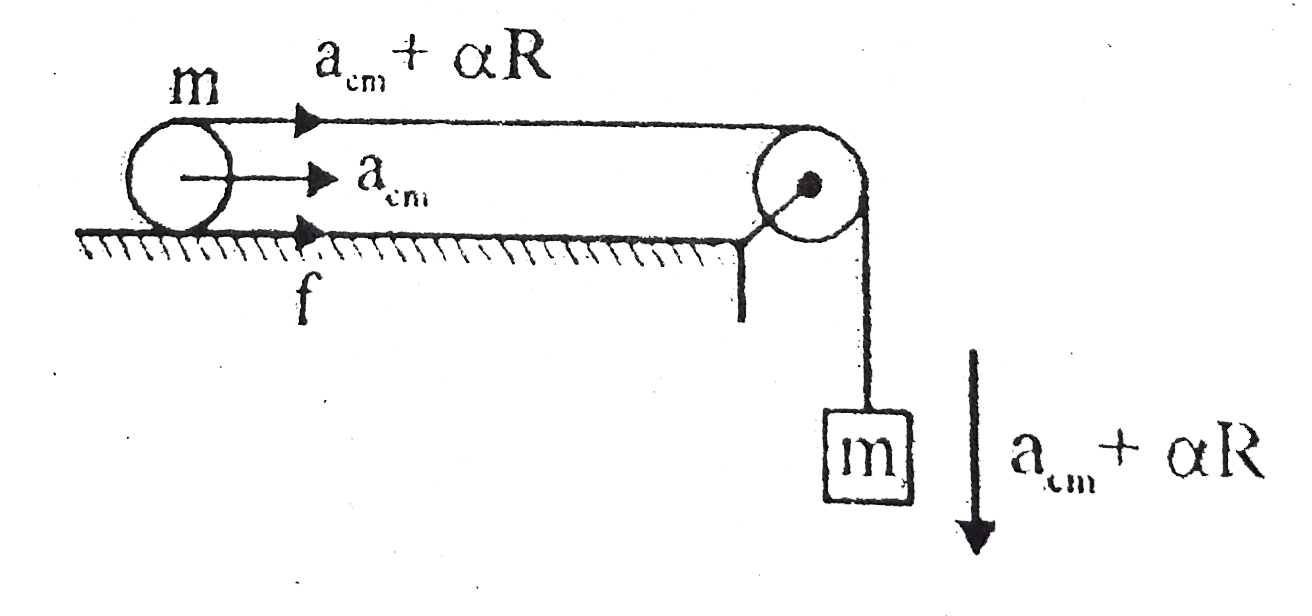
(1)acceleration of the centre of mass of ring is 2g3
(2) acceleration of the hanging particle is 4g3
(3) frictional force (on the ring ) acts along forward direction
(4) Frictional force (on the ring) acts along backward direction
View SolutionA disc is rolling on a surface without slipping. What is the ratio of its translational to rotational kinetic energies ?
View SolutionA ring rotates without slipping on a horizontal smooth surface, the ratio of translational energy to the total energy of the ring is
View SolutionA disk and a ring of the same mass are rolling to have the same kinetic energy. What is ratio of their velocities of centre of mass
View Solution
