माना कि और , जिसमें दीर्घवृत्त (ellipse) की नाभियाँ (Foci) है | माना कि एक परवलय (parabola) जिसका शीर्ष (vertex) मूलबिन्दु (origin) पर और नाभि (focus) पर है, दीर्घवृत को प्रथम चतुर्थाश (first quadrant) में M पर और चतुर्थ चतुर्थाश (fourth quadrant) में N पर प्रतिच्छेदित करता है |
त्रिभुज का लम्बकेन्द्र (orthocenter) है
The correct Answer is:C
Step by step video, text & image solution for माना कि F_(1)(x_(1),0) और F_(2)(x_(2),0), जिसमें x_(1)lt0,x_(2) gt0 दीर्घवृत्त (ellipse) (x^(2))/(9)+(y^(2))/(8)=1 की नाभियाँ (Foci) है | माना कि एक परवलय (parabola) जिसका शीर्ष (vertex) मूलबिन्दु (origin) पर और नाभि (focus) F_(2) पर है, दीर्घवृत को प्रथम चतुर्थाश (first quadrant) में M पर और चतुर्थ चतुर्थाश (fourth quadrant) में N पर प्रतिच्छेदित करता है | त्रिभुज F_(1)MN का लम्बकेन्द्र (orthocenter) है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- शांकव परिच्छेद BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Comprehension type questions) Passage-XII
3 Videos
- शांकव परिच्छेद BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Integer type questions)
17 Videos
- शांकव परिच्छेद BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Comprehension type questions) Passage-X
2 Videos
- वृत्त तथा वृत्तों का निकाय BOOK - ERRORLESS HINDICHAPTER - वृत्त तथा वृत्तों का निकाय EXERCISE - JEE Advanced (Integer type questions)
20 Videos
- श्रेणियाँ BOOK - ERRORLESS HINDICHAPTER - श्रेणियाँ EXERCISE - JEE ADVANCED (MATRIX MATCH TYPE QUESTIONS)
1 Videos
Similar Questions
निचे दी गयी टेबल के तीन कॉलमों में उपलब्ध सूचना का उपयुक्त ढंग से सुमेल कर प्रश्नों के उत्तर दीजिये |
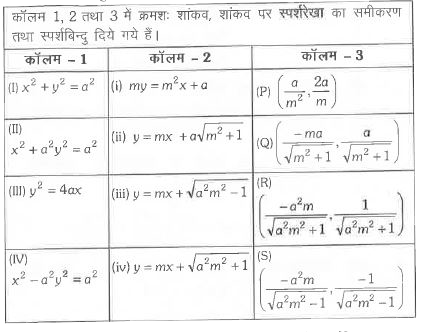
a=√2 के लिए उपयुक्त शांकव (कॉलम 1) के स्पर्शबिन्दु (−1,1) पर एक स्पर्शरेखा खींची जाती है तब निम्न में से केवल कौन सा विकल्प इस स्पर्शरेखा का समीकरण प्राप्त करने का सही संयोजन है
View Solutiony2=8x एक परवलय है। मान लीजिए एक त्रिभुज का क्षेत्रफल है जो नाभिलम्ब जीवा के सिरों और परवलय के बिन्दु P(12,2) द्वारा निर्मित है और Δ2 उस त्रिभुज का क्षेत्रफल है, जो बिन्दु P पर और नाभिलम्ब जीवा के सिरों पर खींची गई स्पर्शियों द्वारा बना है। तब Δ1Δ2 है।
View Solutionमाना कि a,r, s, t शून्येतर वास्तविक संख्याये (non zero real numbers) हैं, P(at2,2at),Q,R(ar2,2ar) तथा S(as2,2as) परवलय y2=4ax पर स्थित विभिन्न बिन्दु है। माना PQ नाभीय जीवा (focal chord) है एवम् रेखायें QR तथा PK समानान्तर हैं, जहाँ K बिन्दु (2a, 0) है।
r का मान है: (i) −1t (ii) t2+1t (iii) 1t (iv) t2−1t
View Solutionरेखा 2x−3y=1 वृत्तीय क्षेत्र x2+y2≤6 को दो भागों में विभाजित करती है। यदि
S={(2,34),(52,34),(14,−14),(18,14)}, तो S में स्थित उन बिन्दुओं की संख्या जो लघुत्तर भाग में अन्दर है, निम्न है
View Solutionनिम्नलिखित कॉलम I को कॉलम II से मिलाये

View Solutionlet A(x_(1),0) and B(x_(2),0) be the foci of the hyperbola (x^(2))/(9)-(y^(2))/(16)=1 suppose parabola having vertex at origin and focus at B intersect the hyperbola at P in first quadrant and at point Q in fourth quadrant.
View SolutionLet F1(x1,0)andF2(x2,0) for x1<0andx2>0 the foci of the ellipse x29+y28=1. Suppose a parabola having vertex at the origin and focus at F2 intersects the ellipse at point M in the first quadrant and at a point N in the fourth quardant. The orthocentre of the triangle F1MN, is
View Solutionयदि X के मान x1,x2,x3,……,xn ग्रहण करता है तथा इनके संगत बारम्बारताएँ क्रमशः f1,f2,f3,……,fn है, तब इनका समांतर माध्य है -
View Solutionएक रेखा X- अक्ष तथा Y-अक्ष को बिंदु A तथा B पर मिलती है AB का मध्य बिंदु (x1,y1) है तो रेखा का समीकरण है -
View Solutionएक परवलय की नाभि मूलबिंदु पर है तथा रेखा x=2 परवलय की नियता है , तो परवलय का शीर्ष है
View Solutionमाना कि परवलय x2=8y का शीर्ष O तथा उस पर बिंदु Q है। यदि बिंदु P रेखाखण्ड OQ को 1:3 के आन्तरिक अनुपात में विभाजित करता है, तो P का बिन्दुपथ है :
View Solutionकोई फलन f(x) किसी बिन्दु x2 पर निम्निष्ठ है , तो f''(x2)………..
View SolutionLet F1(x1,0) and F2(x2,0), for x1<0 and x2>0, be the foci of the ellipse x29+y28=1. Suppose a parabola having vertex at the orgin and focus at F2 intersects the ellipse at point M in the first quadrant and at point N in the fourth quadrant.
The orthocentre of the triangle F1MN is
View SolutionLet F1(x1,0) and F2(x2,0), for x1<0 and x2>0, be the foci of the ellipse x29+y28=1. Suppose a parabola having vertex at the orgin and focus at F2 intersects the ellipse at point M in the first quadrant and at point N in the fourth quadrant.
If the tangents to the ellipse at M and N meet at R and the normal to the parabola at M meets the x-axis at Q, then the ratio of area of the triangle MQR to area of the quadrilateral MF1NF2 is
View Solutionमाना कि F1(x1,0) और F2(x2,0), जिसमें x1<0,x2>0 दीर्घवृत्त (ellipse) x29+y28=1 की नाभियाँ (Foci) है | माना कि एक परवलय (parabola) जिसका शीर्ष (vertex) मूलबिन्दु (origin) पर और नाभि (focus) F2 पर है, दीर्घवृत को प्रथम चतुर्थाश (first quadrant) में M पर और चतुर्थ चतुर्थाश (fourth quadrant) में N पर प्रतिच्छेदित करता है |
त्रिभुज F1MN का लम्बकेन्द्र (orthocenter) है
View Solutionमाना एक ऊर्ध्वाधर मीनार AB ऐसी है कि उसका सिरा A भूमि पर है। माना AB का मध्य-बिन्दु C है और भूमि पर स्थित बिन्दु P ऐसा है कि AP=2AB यदि ∠BPC=β है, तो tanβ बराबर है
View Solution
