The instantaneous velocity of a partical at any instant is equal to time derivative of its position vector and the instancous vector. Which of the following options are wrong ?
The instantancous velocity depends on the instantaneous position vector.
Instantaneous acceleration is independent of instantaneous position vector and instantaneous velocity.
Instananeous acceleration is independent of instantaneous position vector but depends on the instantanous velocity.
Instantaneous acceleration depends both on the instantaneous postion vector and instantaneous velocity.
The correct Answer is:A, C, D
Because →v=d→sdtand→a=d→cdt
hence, velocity is independent of instaneous value of the displacement and acceleration does not depend upon the instantaneous value of velocity. Hence, a,c,d, are incorrect
Topper's Solved these Questions
- MODEL TEST PAPER 1 BOOK - MTG-WBJEECHAPTER - MODEL TEST PAPER 1 EXERCISE - PHYSICS (Category) 2 : Single Option correct type (2 mark)
5 Videos
- MAGNETICSBOOK - MTG-WBJEECHAPTER - MAGNETICSEXERCISE - WB JEE Previous Years Questions (Category 3: One or More than one Option)
2 Videos
- MODEL TEST PAPTER BOOK - MTG-WBJEECHAPTER - MODEL TEST PAPTER EXERCISE - MCQs
80 Videos
Similar Questions
The instantaneous velocity of a particle is equal to time derivative of its position vector and the instantaneous acceleration is equal to time derivatives of its velocity vector. Therefore :
View Solution(A):The Instantaneous velocity does not depend on instantaneous position vector.
(R ):The instantaneous velocity and average velocity of a particle are always same.
View Solution
Knowledge Check
The instantaneous velocity of a particle is equal to time derivative of its position vector and the instantaneous acceleration is equal to time derivatives of its velocity vector. Therefore :
Athe instantaneous velocity depends on the instantaneous position vecotyBinstantaneous acceleration is independent of instantaneous position vector and instantaneous velocityCinstantaneous acceleration is independent of instantaneous position vector but depends on the instantaneous velocityDinstantaneous acceleration depends both on the instataneous position vector and the instantaneous velocity.Which one of the following vector equations is wrong ?
A→P+→Q=→RB→P−→Q=→RC→P+Q=→RD→P=→R−→QIf →A and →B are two vectors, then which of the following is wrong?
A→A.→B=→B.→AB→A+→B=→B+→AC→A×→B=→B×→AD→A×→B=−→B×→A
Figure shows path followed by a particle and position of a particle at any instant. Four different students have represented the velocity vectors and acceleration vectors at the given instant. Which vector diagram can not be true in any situation? (In each figure velocity is tangential to the trajectory).
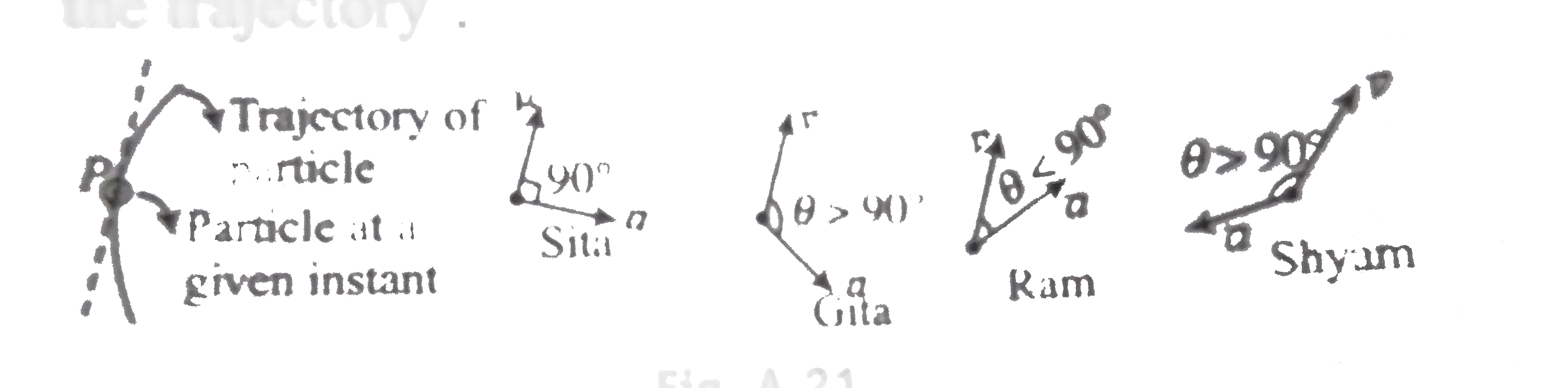
View SolutionFigure shows a path followed by a particle and position of a particle at any instant. Four different students have represented the velocity vectors and acceleration vectors at the given instant. Which vector diagram cannot be true in any situation? (In each figure, velocity is tangential to the trajectory).

View SolutionIf →A and →B are two vectors, then which of the following is wrong?
View SolutionAssertion: The instantaneous velocity does not depend on instantaneous position vector.
Reason: The instantaneous velocity and average velocity of a particle are always same.
View SolutionAssertion : Instantaneous rate of reaction is equal to dx/dt .
Reason : It is the rate of reaction at any particular instant of time
View Solution
