Four particle each of mass 1 kg ar the four corners of a square of side 1m. The workdone to move one of the particles to infinity
2√2 G
(2√2+1√2)G
(2√2+1)G
3G
The correct Answer is:B
Topper's Solved these Questions
- GRAVITATIONBOOK - AAKASH SERIESCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II) LECTURE SHEET (ADVANCED) Straight Objective Type Questions
10 Videos
- GRAVITATIONBOOK - AAKASH SERIESCHAPTER - GRAVITATIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II) LECTURE SHEET (ADVANCED) More than One correct answer Type Questions
1 Videos
- GRAVITATIONBOOK - AAKASH SERIESCHAPTER - GRAVITATIONEXERCISE - PRACTICE SHEET (EXERCISE-IV) Gravitational field, potential & satellite energy (LEVEL-II) Integer Type Questions
4 Videos
- GAUSS.S LAW BOOK - AAKASH SERIESCHAPTER - GAUSS.S LAW EXERCISE - PROBLEMS (LEVEL - II)
12 Videos
- GRAVITATIONALBOOK - AAKASH SERIESCHAPTER - GRAVITATIONALEXERCISE - EXERCISE -3
154 Videos
Similar Questions
Four particle each of mass 1kg are at the four orners of a square of side 1m . The work done to move one of the particles to infinity
View SolutionFour identical particles each of mass 1 kg are arranged at the corners of a square of side length 2√2 m.If one of the particles is removed,the shift In the centre of mass is
View Solution
Knowledge Check
Four particles each of mass 1kg are at the four corners of square of side 1m. The M.I. of the system about a normal axis through centre of square is
A6kgm2B2kgm2C1.25kgm2D2.5kgm2Four particles, each of mass 1kg are placed at the corners of a square of side 1m in the XY plane. If the point of intersecting of the diagonals of the square is taken as the origin, the co-ordinates fo the centre of mass are
A(1,1)B(−1,1)C(1,−1)D(0,0)Four particles A,B, C and D each of mass m are kept at the corners of a square of side L. Now the particle D is taken to infinity by an external agent keeping the other particles fixed at their respective positions . The work done by the gravitational force acting on the particle D During its movement is
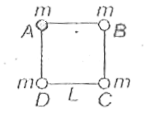 A2Gm2LB−2Gm2LCGm2L(2√2+1√2)D−Gm2L(2√2+1√2)
A2Gm2LB−2Gm2LCGm2L(2√2+1√2)D−Gm2L(2√2+1√2)
Four identical particles each of mass 1 kg are arranged at the corners of a square of side length 2√2m. If one of the particles is removed, the shift in the centre of mass is
View SolutionFour identical particles each of mass 1 kg are arranged at the corners of a square of side length 2√2m. If one of the particles is removed, the shift in the centre of mass is
View SolutionFour particles each of mass 'm' are placed at the four vertices of a square 'a' .Find net force on any one the particle.
View SolutionFour particles each of mass 'm' are placed at the four vertices of a square 'a' .Find net force on any one the particle.
View SolutionFour identical particles each of mass m are arranged at the corners of a square of sie a. If mass of one of th particle is doubled, the shift in the centre of mass of the system is
View Solution
