A swimmer can swim with speed 12 m/s in still water. Speed of river is 6 m/s. Find angle at which he should swim with downstream so that he reaches directly opposite point on other side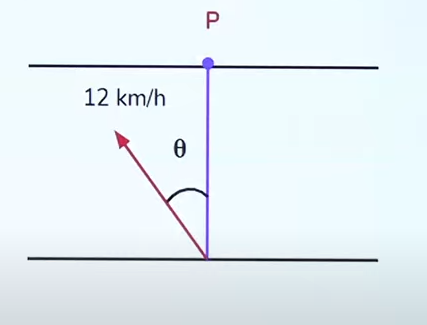 
90 DEGREES
150 DEGREES
60 DEGREES
120 DEGREES
Step by step video & image solution for A swimmer can swim with speed 12 m/s in still water. Speed of river is 6 m/s. Find angle at which he should swim with downstream so that he reaches directly opposite point on other side by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A80videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B40videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions473videos
JEE MAIN
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question492videos
JEE MAIN 2022
Similar Questions
A man can swim at a speed 2ms−1 in still water. He starts swimming in a river at an angle 150∘ with direction of flow of water and reaches at the exactly oppsite point on the opposite bank.
(a) Find the speed of flowing water.
(b) If width of river is 1 km then calculate the time taken alsoView SolutionA swimmer swims with a speed of 10ms at angle of 1200 from direction of river flow. Find velocity of river flow such that swimmer reach exactly opposite point of bank.
View Solution
Knowledge Check
- Question 1 - Select One
A man can swim in still water at 4m/s river is flowing at 2m/s. the angle with downstream at which he should swim to cross the river minimum drift is
A120∘B150∘C30∘D60∘ - Question 2 - Select One
A man swims from a point A on the bank of a river if width 100m. When he swims perpendicular to the water current, he reaches the other bank 50m downstream. The angle to the bank at which he should swim, to reach the directly opposite point B on the other bank is.
.A10∘ upstreamB20∘ upstreamC30∘ upstreamD60∘ upstream - Question 3 - Select One
If speed of water in river is 4 m/s and speed of swimmer with respect to water is 3 m/s, then in which direction the swimmer must swim so that he will reach directly opposite end?
A127∘ with direction of river flowB60∘ with direction of river flowC143∘ with direction of river flowDSwimmer will never reach directly opposite end
A man can walk on the shore at a speed v1 = 6 km/hr & swim in still water with a speed V2 = 5 km/hr. If the speed of water is V3 = 4 km/hr, at what angle should he head in the river in order to reach the exactly opposite point of the other shore in "shortest time including his swimming & walking?
View SolutionA swimmer wishes to reach directly opposite point on the other bank of a river,flowing with velocity 4√2ms.The swimmer can swim with 8ms in still water.The angle with the flow direction he should swim to reach the directly opposite point is (A)450 (B)900 (C)1350 (D)1500
View SolutionA man wishes to cross a river of width 120 m by a motorboat. His rowing speed in still water is 3m/s and his maximum walking speed is 1m/s. The river flows with velocity of 4m/s.
(a) Find the path which he should take to get to the point directly opposite to his starting point in the shortest time.
(b) Also, find the time which he takes to reach his destination.View SolutionA swimmer can swim with velocity of 12 km/h in still water. Water flowing in a river has velocity 6 km/h. The direction with respect to the direction of flow of river water he should swim in order to reach the point on the other bank just opposite to his starting point is. (Round off to the Nearest Integer)
(Find the angle in degrees)View SolutionA river flows with a speed more than the maximum speed with which a person can swim in still water. He intends to cross the river by the shortest possible path (i.e., he wants to reach the point on the opposite bank which directly opposite to the starting point). Which of the following is correct ?
View Solution