A man wishes to cross a river of width 120 m by a motorboat. His rowing speed in still water is 3m/s and his maximum walking speed is 1m/s. The river flows with velocity of 4m/s.
(a) Find the path which he should take to get to the point directly opposite to his starting point in the shortest time.
(b) Also, find the time which he takes to reach his destination.
The correct Answer is:A, B, C, D
(a)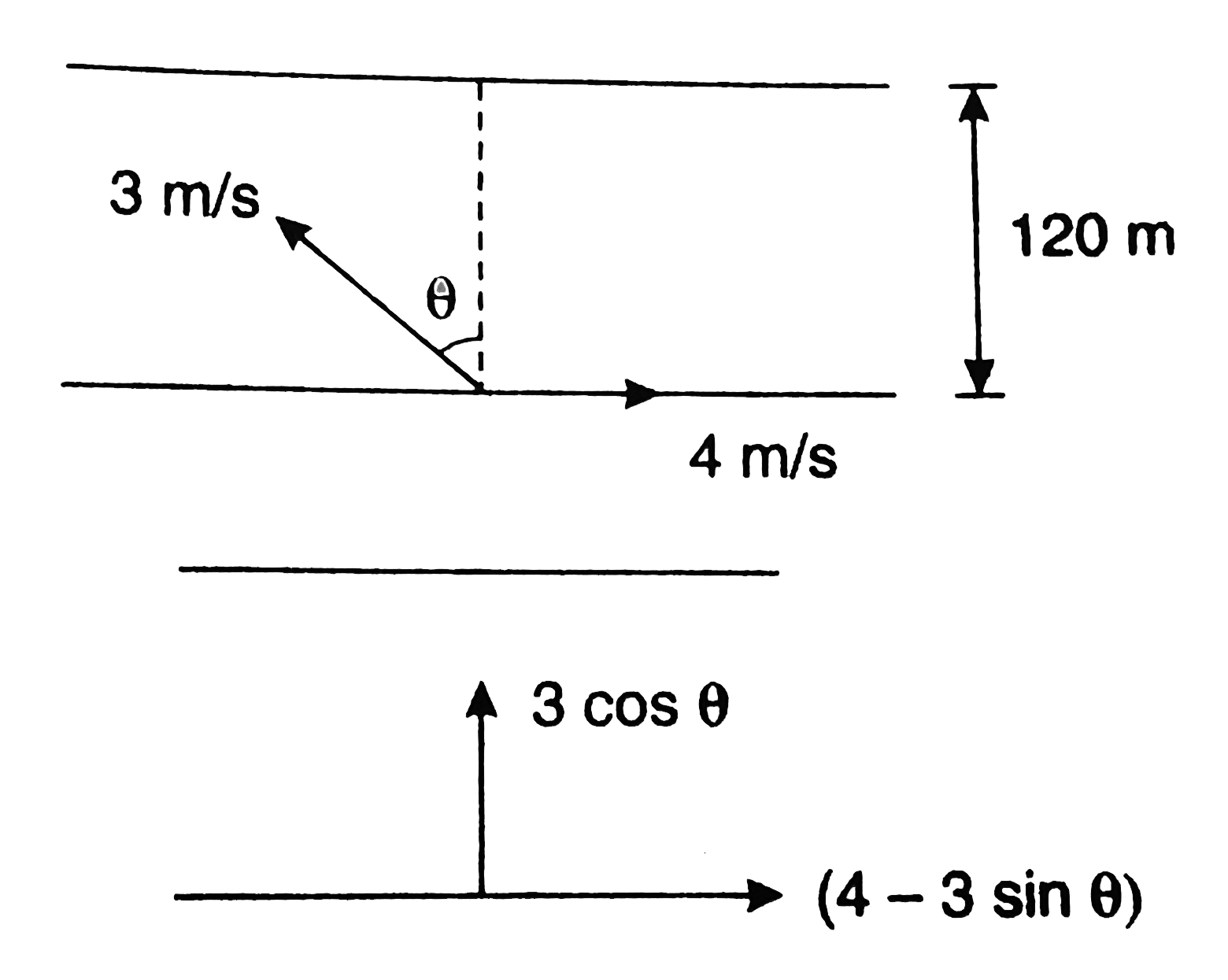 
Time to cross the river
t1=1203cosθ=40cosθ=40secθ
Drift along the river x=(4−3sinθ)(40cosθ)
=(16secθ−120tanθ)
To reach directly oppsite, this drift will be
covered by walking speed.
Time taken in this,
t2=160secθ−120tanθ1
=160secθ−120tanθ
∴ Total time taken
t=t1+t2=(200secθ−120tanθ)
For t to be minimum, dtdθ=0
or 200secθtanθ−120sec2θ=0 or θ=sin−(3/5) (b) tmin=200secθ−120tanθ(where,sinθ=35)
=200×54−120×34
=250−90=160s=2min40s
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - SCQ_TYPE41videos
KINEMATICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - MCQ_TYPE23videos
KINEMATICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - Comprehension7videos
KINEMATICS
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
Similar Questions
A man wants to cross a river 500 m wide. His rowing speed in still water is 3 kmph. The river flows at a speed of 2 kmph. If the man's walking speed on the shore is 5kmph, (a) find the path he should take to get to the point directly opposite to his starting point in the shortest time. (b) How long does it take to get there?
View SolutionA swimmer can swim with speed 12 m/s in still water. Speed of river is 6 m/s. Find angle at which he should swim with downstream so that he reaches directly opposite point on other side
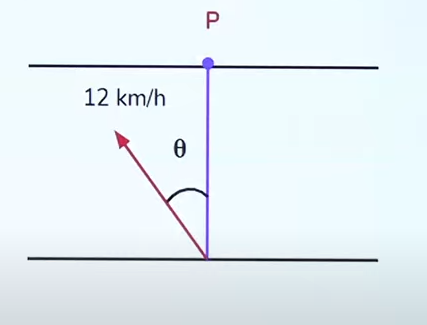View Solution
Knowledge Check
- Question 1 - Select One
The rowing speed of a man relative to water is 5kmh−1 and the speed of water flow is 3kmh−1. At angle to the river flow should he head if he wants to reach a point on the other bank, directly opposite to starting point ?
A127∘B143∘C120∘D150∘ - Question 2 - Select One
The velocity of a boat in still water is 10m/s.If water flows in the river with a velocity of 6m/s what is the difference in times taken to cross the river in the shortest path and the shortest time.The width of the river is 80m.
A1sB10sC√32sD2s - Question 3 - Select One
A swimmer wishes to cross a river 500m width flowing at a rate u. his speed w.r.t. still water is v. for this he makes an angle θ with the vertical as shown in the given figure then:
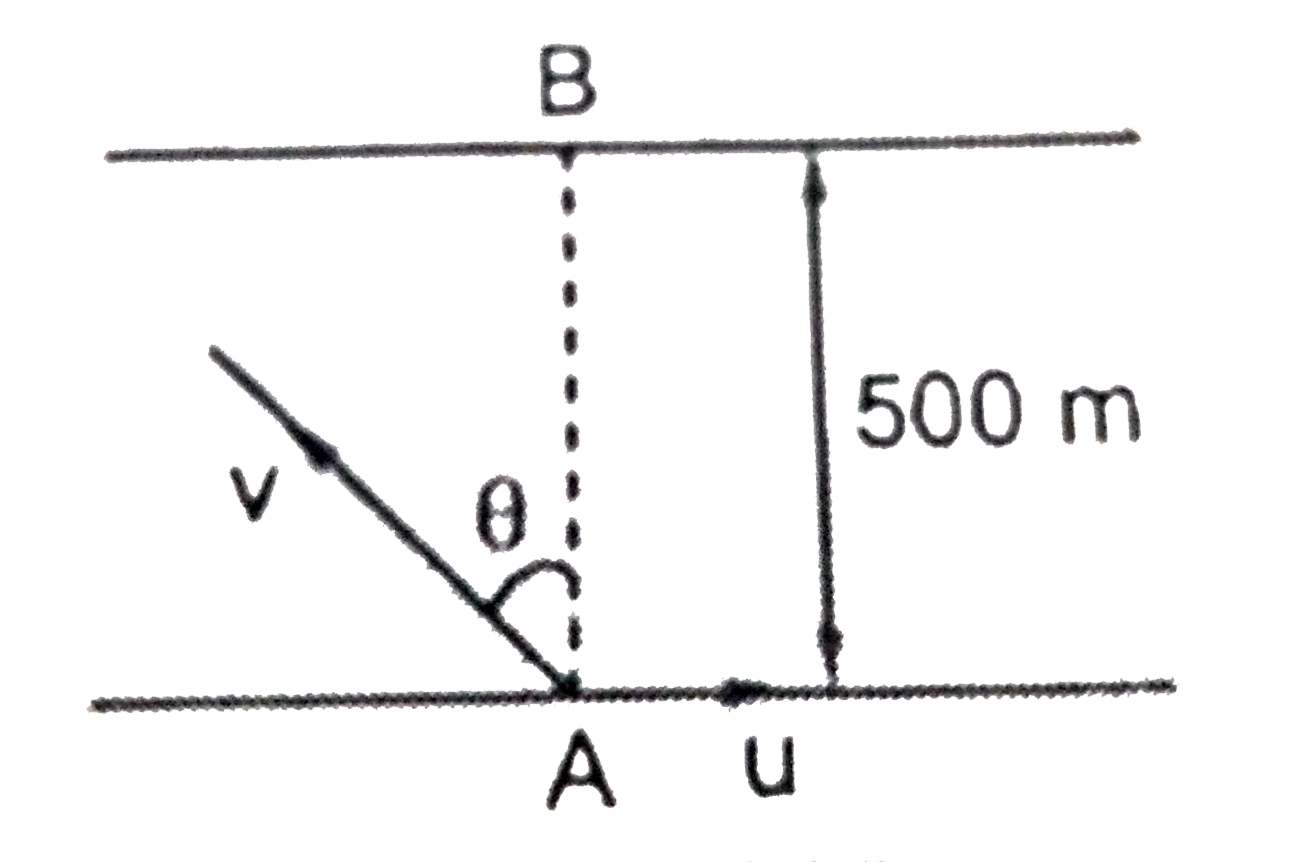Ato cross the river in minimum time θ=0∘Bto cross the river in minumum time, θ=30∘Cfor u=3km/hr and v=5km/hr, the time taken to cross the river in minimum time will be 6min.Dfor u=3 km/hr and v=5km/hr, the time taken to cross the river in minimum time will be 3min.
A boat can swim in still water with speed of 20 km/h . a river of width 500 meters flows with speed of 6 km./h. In what direction . . . . should the boat be pointed , so that it reaches the opposite bank at the point directly opposite the starting point ? Also find the time . . . . . taken. Is this the least time of crossing ?
View SolutionA man can swim in still water with speed v. He wants to cross a river of width d that flows with speed u and reaches the point directely opposite to his starting point. (a) In which direction should he try to swim (i.e., find the angle the makes with the river flow)? (b) much time will he take to cross the river ?
View SolutionA man is crossing a river flowing with velocity of 5m/s. He reaches a point directly across at a distance of 60m in 5 sec. His velocity in still water should be
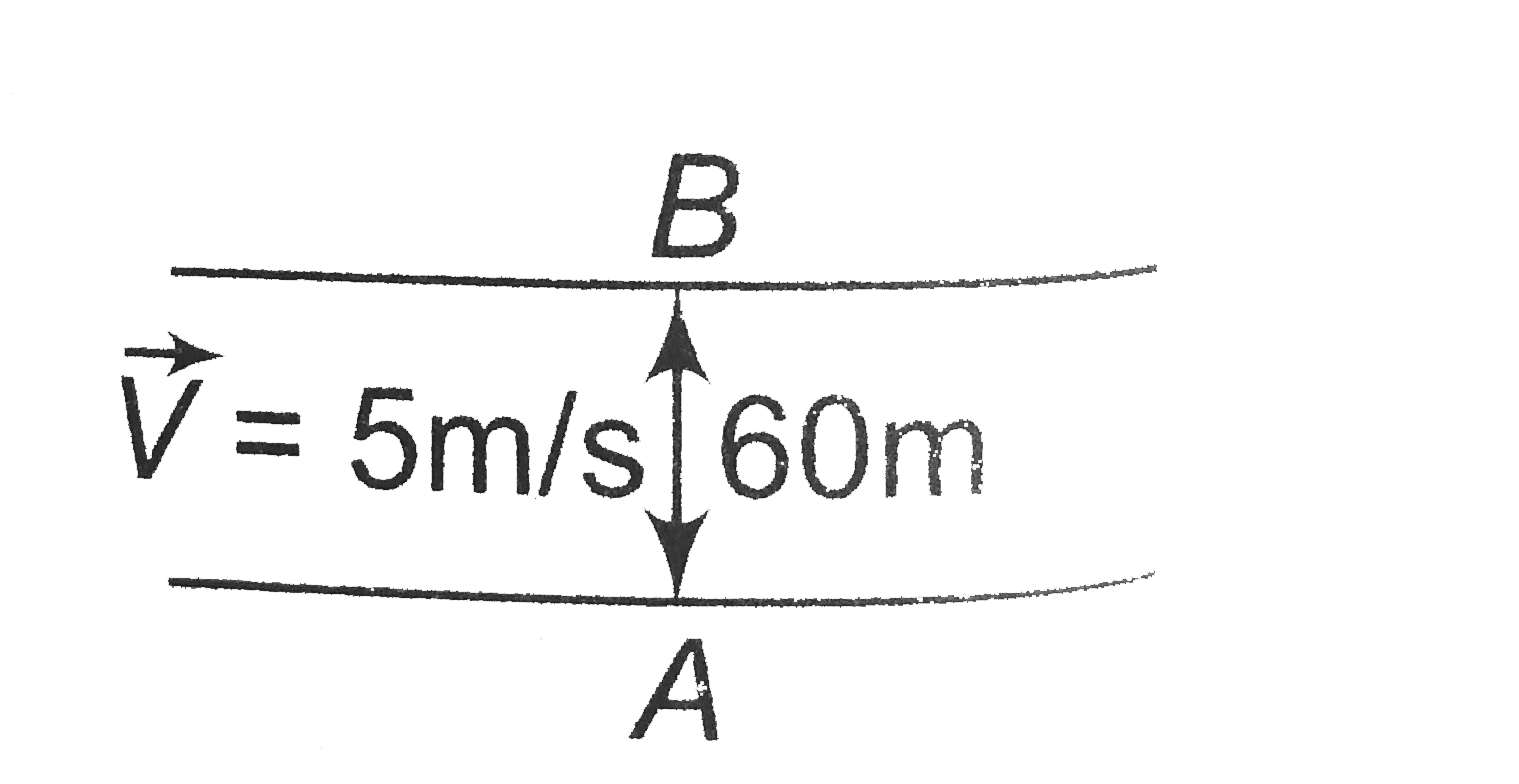View SolutionA man is crossing a river flowing with velocity of 5m/s. He reaches a point directly across at a distance of 60m in 5sec. his velocity in still water should be
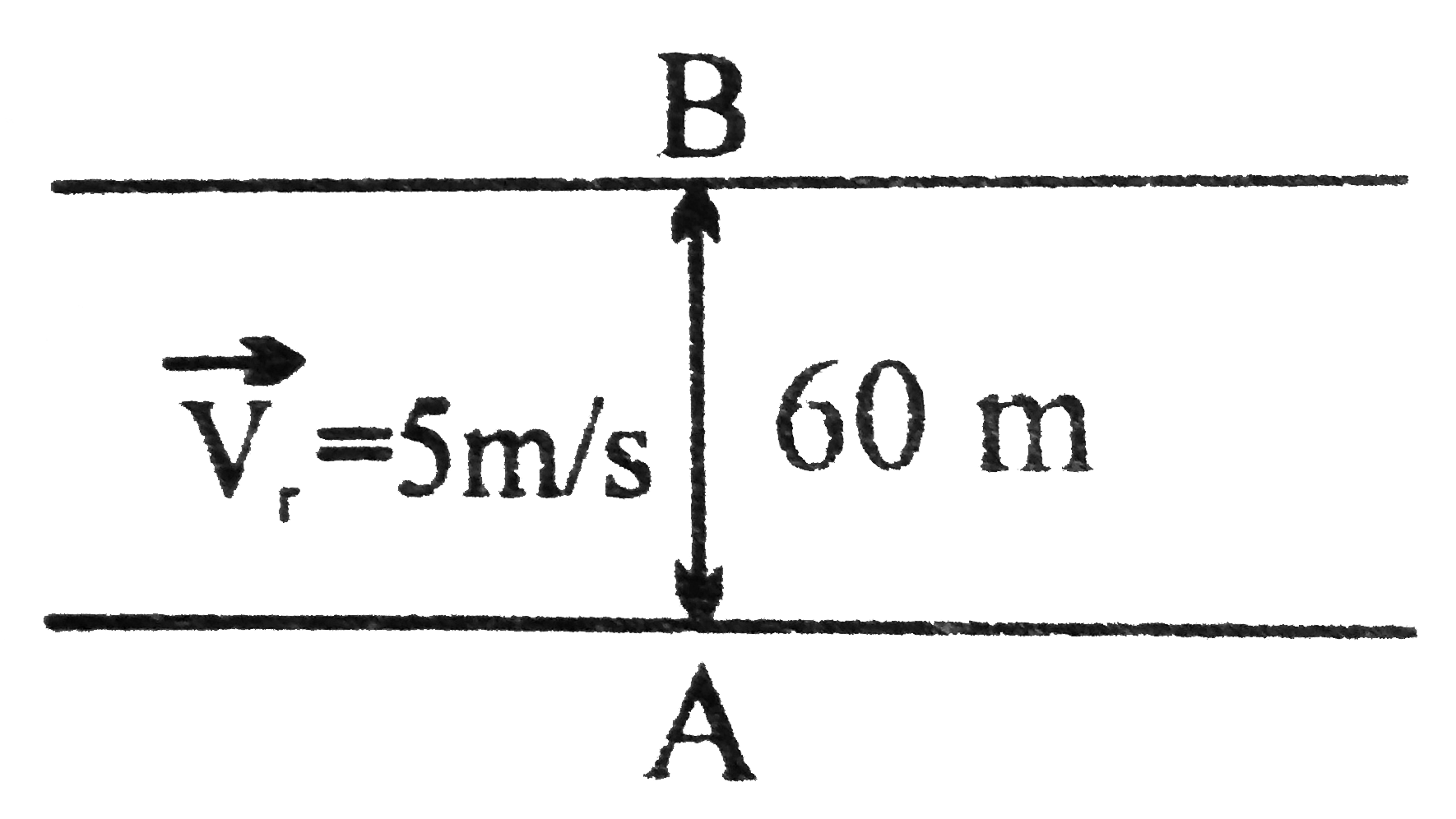View SolutionVelocity of boat in still water is 13 m/s. If water flows ina river with a velocity of 5 m/s. What is the difference in times taken by him to cross the river in the shortest path and in the shortest time. If the width of the river is 10 m.
View Solution