For a polyatomic ideal gas, vibrational degree are 24. CpCv is
1.01
1.03
1.05
1.07
To solve the problem of finding the ratio CpCv for a polyatomic ideal gas with 24 vibrational degrees of freedom, we can follow these steps:
1. Identify Degrees of Freedom:
For a polyatomic ideal gas, the total degrees of freedom f can be expressed as:
f=Ft+Fr+Fv
where:
- Ft = translational degrees of freedom = 3
- Fr = rotational degrees of freedom = 3 (for polyatomic gases)
- Fv = vibrational degrees of freedom = 24
Therefore, we can calculate:
f=3+3+24=30
2. Calculate Cv:
The molar specific heat at constant volume Cv is given by the formula:
Cv=f2R
Substituting the value of f:
Cv=302R=15R
3. Calculate Cp:
Using Mayer's relation, which states:
Cp−Cv=R
We can express Cp as:
Cp=Cv+R
Substituting the value of Cv:
Cp=15R+R=16R
4. Calculate the Ratio CpCv:
Now, we can find the ratio:
CpCv=16R15R=1615
This simplifies to:
CpCv=1.0667≈1.07
Final Answer:
Thus, the ratio CpCv is approximately 1.07.
---
For a polyatomic ideal gas, the total degrees of freedom f can be expressed as:
f=Ft+Fr+Fv
where:
- Ft = translational degrees of freedom = 3
- Fr = rotational degrees of freedom = 3 (for polyatomic gases)
- Fv = vibrational degrees of freedom = 24
f=3+3+24=30
The molar specific heat at constant volume Cv is given by the formula:
Cv=f2R
Substituting the value of f:
Cv=302R=15R
Using Mayer's relation, which states:
Cp−Cv=R
We can express Cp as:
Cp=Cv+R
Substituting the value of Cv:
Cp=15R+R=16R
Now, we can find the ratio:
CpCv=16R15R=1615
This simplifies to:
CpCv=1.0667≈1.07
Thus, the ratio CpCv is approximately 1.07.
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
Knowledge Check
For polyatomic molecules having 'f' vibrational modes, the ratio of two specific heat, CPCV is
A1+f2+fB2+f3+fC4+f3+fD5+f4+fFor polyatomic molecules having 'f' vibrational modes, the ratio of two specific heat, CPCV is
A1+f2+fB2+f3+fC4+f3+fD5+f4+fFor polyatomic molecules having 'f' vibrational modes, the ratio of two specific heats, CPCV is
A1+f2+fB2+f3+fC4+f3+fD5+f4+f
Find out the ratio CpCv of polyatomic gas for 2 vibrational degree of freedom
View SolutionFind CpCv for monatomic ideal gas.
View Solution1 mole of an ideal gas undergo adiabatic process which increases temperature from 270C→370C . Gas is polyatomic has 4 vibrational mode of freedom. Find net work
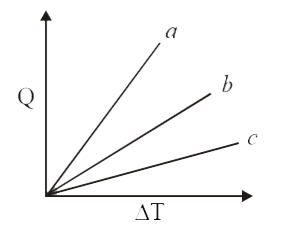
View SolutionFigure shows the variation in temperature (DT) with the amount of heat supplied (Q) in an isobaric process corresponding to a monoatomic (M), diatomic (D) and a polyatomic (P) gas. The initial state of all the gases are the same and the scales for the two axes coincide. Ignoring vibrational degrees of freedom, the lines a, b and c respectively correspond to
View SolutionFor 2 mole of an ideal gas , the relation between Cp&Cv (non-molar) are:
View Solution