Let $P_{1}$ be the plane $x - 2 y + 2 z = 0$ and a point $A (1, 2, 3)$ . Let there be another plane $P_{2}$ which is parrallel to $P_{1}$ and at unit distance from A. If $P_{2}$ is $a x + b y + c z + d = 0$ then +ve value of $(\frac{b - d}{c - a})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

Answer

Step by step video, text & image solution for Let P_1 be the plane x-2y+2z=0 and a point A(1,2,3) . Let there be another plane P_2 which is parrallel to P_1 and at unit distance from A. If P_2 is ax+by+cz+d=0 then +ve value of ((b-d)/(c-a)) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Knowledge Check

Question 1 - Select One
Consider the plane $π_{1} : 2 x - 3 y + 4 z + 9 = 0$ and the point P(1,-2,3).

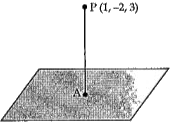
Distance between $π_{1}$ and P is _______ units.
A5
B $\sqrt{29}$
C $\sqrt{13}$
D $2 \sqrt{2}$
Question 2 - Select One
Let P be the image of the point (3, 1, 7) with respect to the plane $x - y + z = 3$ . The equation of the plane passing through P and parallel to $x - 2 y + 3 z = 7$ is
A $x - 2 y + 3 z = 2$
B $2 x - 4 y + 6 z = 7$
C $x + 2 y - 6 z + 2 = 0$
D $x - 2 y + 3 z + 2 = 0$
Question 3 - Select One
Let two planes $p_{1} : 2 x - y + z = 2 and p_{2} : x + 2 y - z = 3$ are given :
equation of the plane through the intersection of $p_{1} and p_{2}$ and the point $(3, 2, 1)$ is :
A $3 x - y + 2 z - 9 = 0$
B $x - 3 y + 2 z + 1 = 0$
C $2 x - 3 y + z - 1 = 0$
D $4 x - 3 y + 2 z - 8 = 0$

Let $P_{1}$ be the plane $x - 2 y + 2 z = 0$ and a point $A (1, 2, 3)$ . Let there be another plane $P_{2}$ which is parrallel to $P_{1}$ and at unit distance from A. If $P_{2}$ is $a x + b y + c z + d = 0$ then +ve value of $(\frac{b - d}{c - a})$

The correct Answer is:4

Similar Questions

Knowledge Check

Consider the plane $π_{1} : 2 x - 3 y + 4 z + 9 = 0$ and the point P(1,-2,3).

Distance between $π_{1}$ and P is _______ units.

Let P be the image of the point (3, 1, 7) with respect to the plane $x - y + z = 3$ . The equation of the plane passing through P and parallel to $x - 2 y + 3 z = 7$ is

Let two planes $p_{1} : 2 x - y + z = 2 and p_{2} : x + 2 y - z = 3$ are given :
equation of the plane through the intersection of $p_{1} and p_{2}$ and the point $(3, 2, 1)$ is :

Recommended Questions

Let P1 be the plane x−2y+2z=0 and a point A(1,2,3) . Let there be another plane P2 which is parrallel to P1 and at unit distance from A. If P2 is ax+by+cz+d=0 then +ve value of (b−dc−a)

The correct Answer is:4

Similar Questions

Knowledge Check

Consider the plane π1:2x−3y+4z+9=0 and the point P(1,-2,3). Distance between π1 and P is _______ units.

Let P be the image of the point (3, 1, 7) with respect to the plane x−y+z=3. The equation of the plane passing through P and parallel to x−2y+3z=7 is

Let two planes p1:2x−y+z=2andp2:x+2y−z=3 are given : equation of the plane through the intersection of p1andp2 and the point (3,2,1) is :

Recommended Questions

Let $P_{1}$ be the plane $x - 2 y + 2 z = 0$ and a point $A (1, 2, 3)$ . Let there be another plane $P_{2}$ which is parrallel to $P_{1}$ and at unit distance from A. If $P_{2}$ is $a x + b y + c z + d = 0$ then +ve value of $(\frac{b - d}{c - a})$

Consider the plane $π_{1} : 2 x - 3 y + 4 z + 9 = 0$ and the point P(1,-2,3).

Distance between $π_{1}$ and P is _______ units.

Let P be the image of the point (3, 1, 7) with respect to the plane $x - y + z = 3$ . The equation of the plane passing through P and parallel to $x - 2 y + 3 z = 7$ is

Let two planes $p_{1} : 2 x - y + z = 2 and p_{2} : x + 2 y - z = 3$ are given :
equation of the plane through the intersection of $p_{1} and p_{2}$ and the point $(3, 2, 1)$ is :