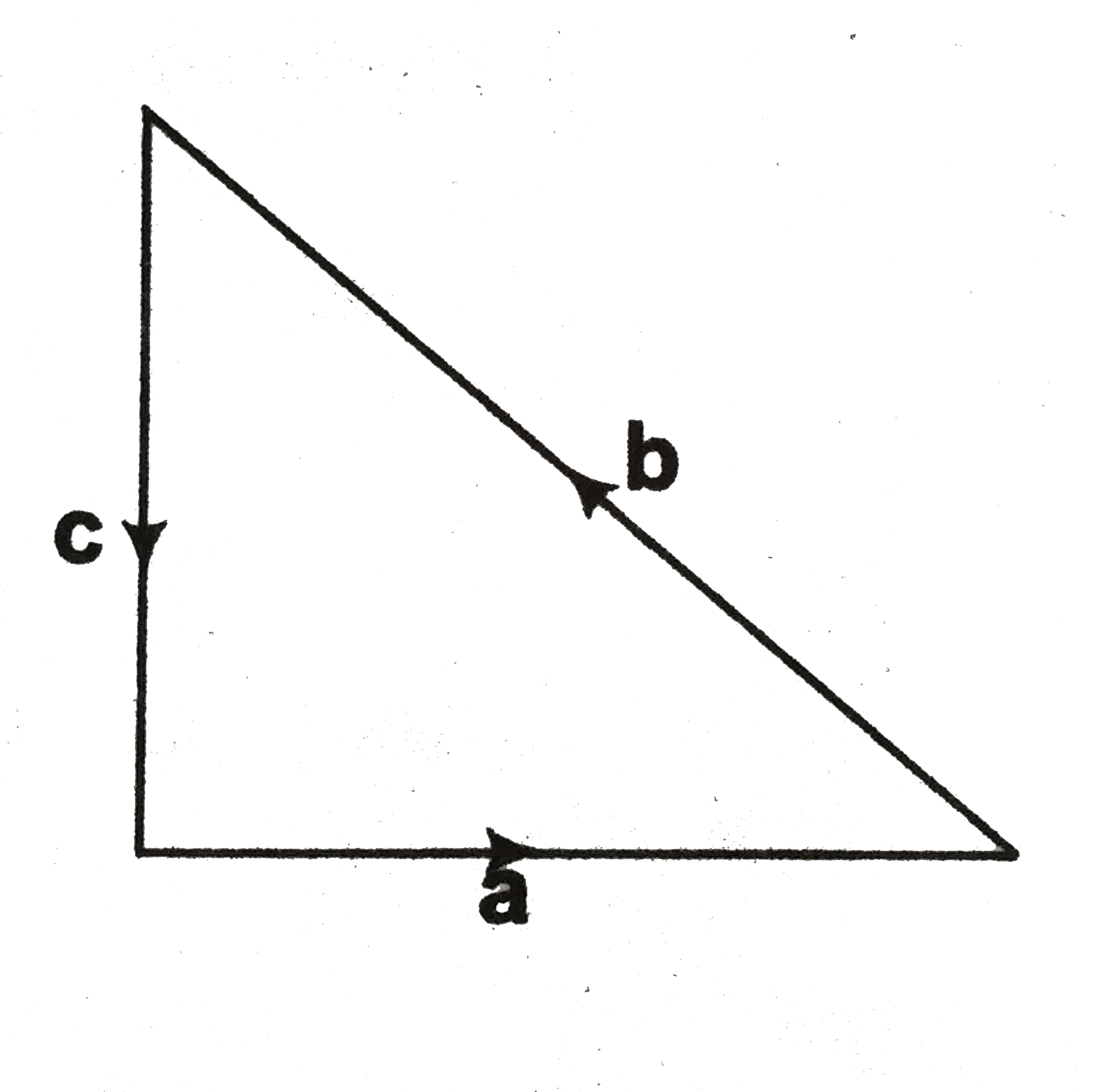
Show that the vectors and form a right angled triangle.
We have
Hence, a,b,c are coplanar.
Also, we observe that no two of theses vectors are parallel.
Further,
Dot product of two non-zero vectors is zero. Hence, they are perpendiuclar
so they form a right angled triangle.
,
 .
.
Topper's Solved these Questions
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 5.1
5 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 5.2
4 Videos
- UNITS, DIMENSIONS & ERROR ANALYSIS BOOK - DC PANDEY ENGLISHCHAPTER - UNITS, DIMENSIONS & ERROR ANALYSIS EXERCISE - Medical entrances gallery
32 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
Similar Questions
Show that the vectors a=3ˆi−2ˆj+ˆk,b=ˆi−3ˆj+5ˆk and c=2ˆi+ˆj−4ˆk form a right angled triangle.
View SolutionUsing dot product of vectors show that the vectors 2ˆi−ˆj+ˆk,ˆi−3ˆj−5ˆkand3ˆi−4ˆj−4ˆk form a righat angled triangle
View SolutionProve that the vectgors 2ˆi−ˆj+ˆk,ˆi−3ˆj−5ˆkand3ˆi−4ˆj−4ˆk form a righat angled triangle.
View SolutionShow that vectors 3ˆi−2ˆj+2ˆk,ˆi−3ˆj+4ˆkand2ˆi−ˆj−2ˆk form a right angled triangle
View SolutionShow that the vectors ˆi−ˆj−ˆk,2ˆi+3ˆj+ˆkand7ˆi+3ˆj−4ˆk are coplanar.
View SolutionProve that the vecotos 3ˆi+5ˆj+2ˆk,2ˆi−3ˆj−5ˆkand5ˆi+2ˆj−3ˆk form the sides of an equlateral triangle.
View SolutionShow that the vectors 2ˆi−ˆj+ˆkandˆi−3ˆj−5ˆk are at righat angles.
View SolutionIf the vectors 6ˆi−2ˆj+3ˆkk,2ˆi+3ˆj−6ˆkand3ˆi+6ˆj−2ˆk form a triangle, then it is
View SolutionProve that the points ˆi−ˆj,4ˆi−3ˆj+ˆkand2ˆi−4ˆj+5ˆk are the vertices of a right angled triangle.
View SolutionShow that the vectors
ˆi−3ˆj+2ˆk,2ˆi−4ˆj−ˆkand3ˆi+2ˆj−ˆk and linearly independent.
View Solution
