Consider the earth as a uniform sphere if mass M and radius R. Imagine a straight smooth tunnel made through the earth which connects any two points on its surface. Show that the motion of a particle of mass m along this tunnel under the action of gravitation would be simple harmonic. Hence, determine the time that a particle would take to go from one end to the other through the tunnel.
The correct Answer is:C
To solve the problem, we need to show that the motion of a particle of mass m along a straight tunnel through the Earth is simple harmonic motion (SHM) and then determine the time taken for the particle to travel from one end of the tunnel to the other.
Step 1: Understanding the gravitational force inside the Earth
When a mass m is at a distance r from the center of the Earth, only the mass of the Earth that is enclosed within that radius contributes to the gravitational force acting on m. The mass M′ of the Earth within radius r can be expressed as:
M′=M(r3R3)
where M is the total mass of the Earth and R is the radius of the Earth.
Step 2: Applying Newton's law of gravitation
The gravitational force F acting on the mass m at distance r from the center of the Earth is given by:
F=GM′mr2=G(Mr3R3)mr2=GMmrR3
Step 3: Expressing the force in terms of displacement
If we let x be the displacement from the center of the Earth, where x=r, then the force can be rewritten as:
F=GMmR3x
Since the force is directed towards the center, we can express it as:
F=−GMmR3x
Step 4: Identifying the motion as simple harmonic motion
The equation F=−kx (where k=GMmR3) is characteristic of simple harmonic motion. Thus, we can identify that the motion of the particle is indeed SHM.
Step 5: Determining the angular frequency
From the SHM relation, we know that:
F=ma=−kx
This gives us the angular frequency ω:
ω2=GMR3
Step 6: Finding the time period of the oscillation
The time period T of the oscillation is given by:
T=2π√R3GM
Step 7: Calculating the time taken to travel from one end to the other
The time taken to travel from one end of the tunnel to the other is half the time period:
Time taken=T2=π√R3GM
Final Answer
Thus, the time taken for the particle to go from one end of the tunnel to the other is:
Time taken=π√R3GM
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Assertion And Reason10videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Single Correct24videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example Type 133videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
Four particles of equal masses M move along a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View SolutionTwo particles of equal mass go round a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
View Solution
Knowledge Check
- Question 1 - Select One
Two particles of equal mass go around a circle of radius R under the action of their mutual gravitational attraction. The speed v of each particle is
A12R√(1Gm)B√(Gm2R)C12√(GmR)D√(4GmR)
Imagine a narrow tunnel between the two diametrically opposite points of the earth. A particle of mass m is released in this tunnel . The time period of oscillation is
View Solution_S01_003_Q01.png)
Two particles of equal mass (m) each move in a circle of radius (r) under the action of their mutual gravitational attraction find the speed of each particle.View SolutionA tunnel is dug along a diameter of the earth. Find the force in on a particle of mass m placed in the tunnel at a distance x from the centre.
View SolutionA ball of mass m is dropped from a height h equal to the radius of the earth above the tunnel dug through the earth as shows in the figure. Choose the correct options. (Mass of earth =M)
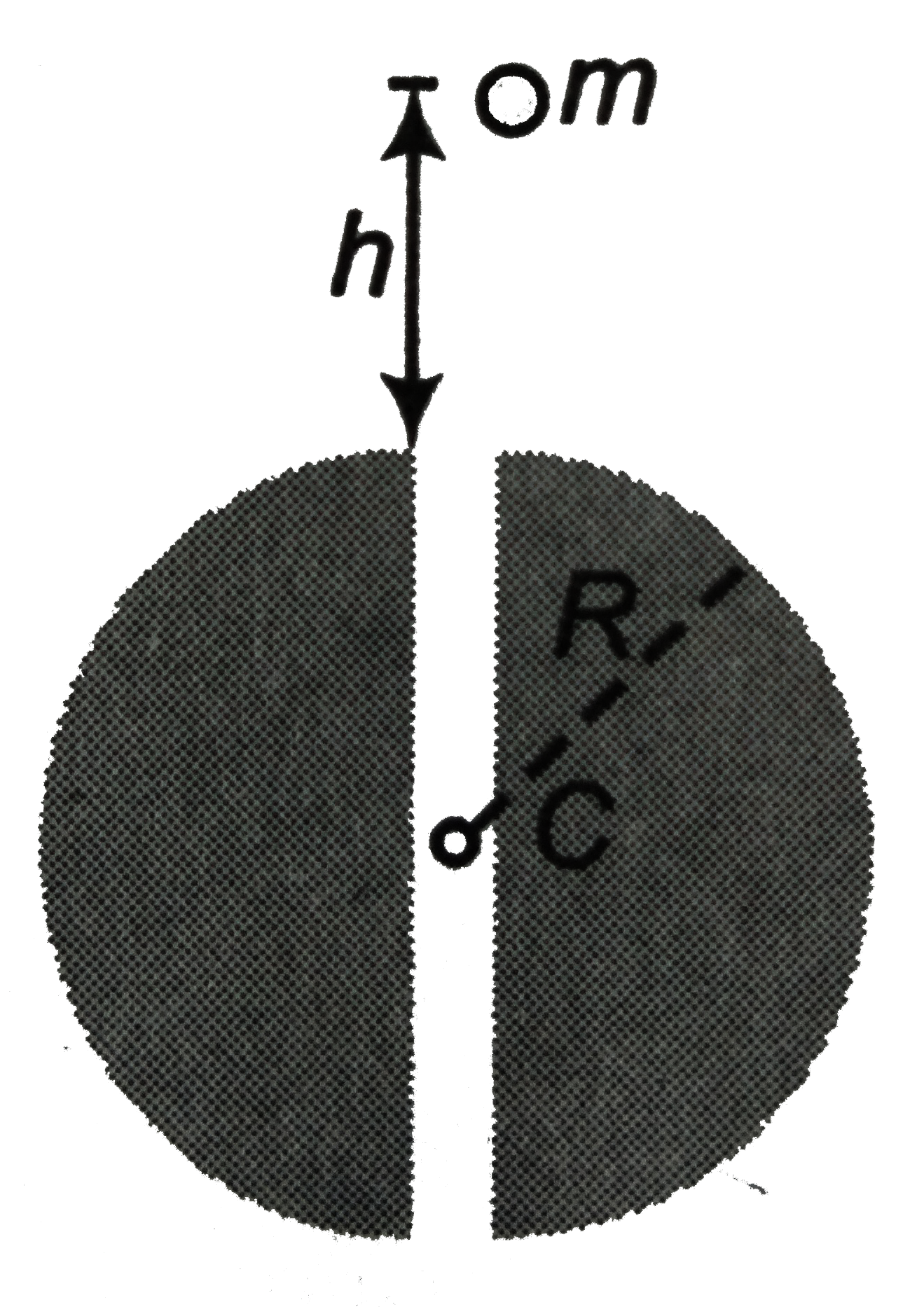 View Solution
View SolutionTwo particles of equal masses m go round a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is v=√GmnR. Find the value of n.
View SolutionIf a smooth tunnel is dug across a diameter of earth and a particle is released from the surface of earth, the particle oscillate simple harmonically along it
Time period of the particle is not equal toView SolutionIf a smooth tunnel is dug across a diameter of earth and a particle is released from the surface of earth, the particle oscillate simple harmonically along it
Maximum speed of the particle isView Solution

