Assertion: At depth h below the water surface pressure is p. Then at depth 2h pressure will be 2p. (Ignore density variation).
Reason: With depth pressure increases linearly.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:D
To solve the question, we need to analyze the assertion and the reason provided.
1. Understanding Pressure at Depth:
The pressure at a depth h in a fluid is given by the formula:
P=P0+ρgh
where:
- P0 is the atmospheric pressure at the surface,
- ρ is the density of the fluid,
- g is the acceleration due to gravity,
- h is the depth.
2. Pressure at Depth h:
According to the assertion, at depth h, the pressure is p. Therefore, we can write:
p=P0+ρgh
3. Pressure at Depth 2h:
Now, we need to find the pressure at depth 2h:
P(2h)=P0+ρg(2h)=P0+2ρgh
4. Relating Pressures:
We already know from step 2 that:
P0+ρgh=p
Therefore, we can substitute P0 from this equation into the pressure at 2h:
P(2h)=(p−ρgh)+2ρgh=p+ρgh
5. Conclusion:
From the above equation, we see that:
P(2h)=p+ρgh≠2p
Thus, the assertion that the pressure at depth 2h is 2p is incorrect.
6. Evaluating the Reason:
The reason states that pressure increases linearly with depth. This is true because the pressure increases by a constant amount ρg for each unit increase in depth. Therefore, the reason is correct.
Final Answer:
- The assertion is false.
- The reason is true.
The pressure at a depth h in a fluid is given by the formula:
P=P0+ρgh
where:
- P0 is the atmospheric pressure at the surface,
- ρ is the density of the fluid,
- g is the acceleration due to gravity,
- h is the depth.
According to the assertion, at depth h, the pressure is p. Therefore, we can write:
p=P0+ρgh
Now, we need to find the pressure at depth 2h:
P(2h)=P0+ρg(2h)=P0+2ρgh
We already know from step 2 that:
P0+ρgh=p
Therefore, we can substitute P0 from this equation into the pressure at 2h:
P(2h)=(p−ρgh)+2ρgh=p+ρgh
From the above equation, we see that:
P(2h)=p+ρgh≠2p
Thus, the assertion that the pressure at depth 2h is 2p is incorrect.
The reason states that pressure increases linearly with depth. This is true because the pressure increases by a constant amount ρg for each unit increase in depth. Therefore, the reason is correct.
- The assertion is false.
- The reason is true.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion and Reason1videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct49videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.84videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
An air bubble of radius r in water is at a depth h below the water surface at some instant. If P is atmospheric pressure, d and T are density and surface tension of water respectively . the pressure inside the bubble will be :
View SolutionThe acceleration due to gravity at a depth R/2 below the surface of the earth is
View Solution
Knowledge Check
- Question 1 - Select One
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth - Question 2 - Select One
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
A small air bubble of radius 'r' is at a depth 'h' below the water surface (density of water=ρ). Surface tension of water is T, atmospheric pressure is p0. Find pressure inside the air bubble for the condition r<<h
View SolutionThe absolute pressure at a depth h below the surface of a liquid of density ρ is [Given Pa = atmospheric pressure, g = acceleration due to gravity]
View SolutionThe diagram below shows the position of two divers A and B in river water at the depth of h1 and h2 respectively from the water surface.
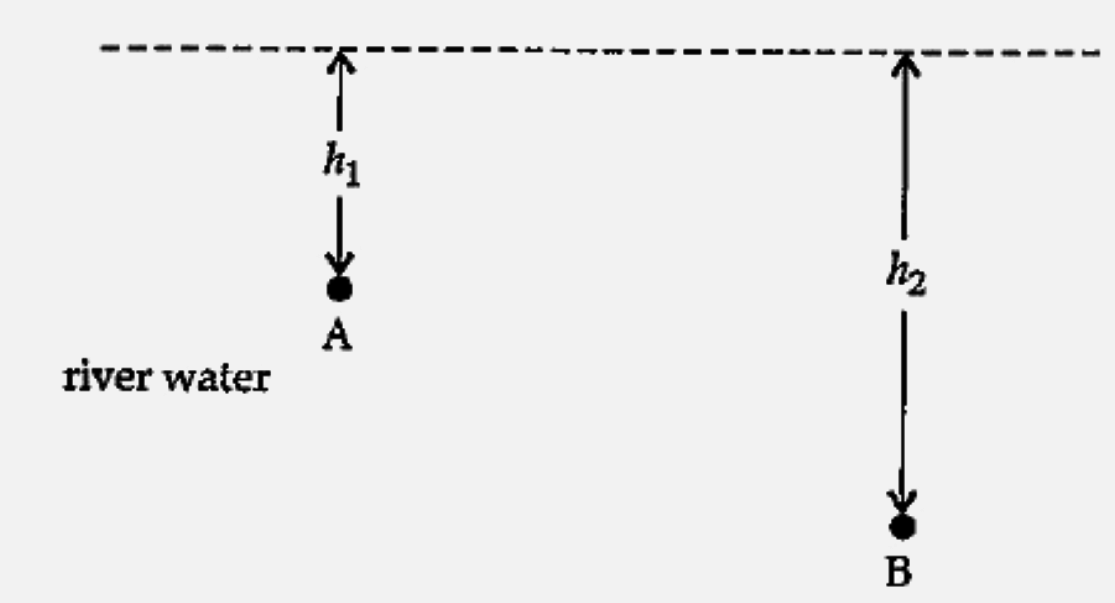
The two divers were later made to dive in a sea. For the same depth, they experience more pressure in the sea compared to that in the river. Why?View SolutionAssertion: In the siphon shown in figure, pressure at P is equal to atmospheric pressure

Reason: Pressure at Q is atmospheric pressure and points P and Q are at same levels.View SolutionAssertion : If pressure is increased , bulk modulus of gases will increase.
Reason : With increase in pressure , temperature of gases also increases .View SolutionDifferent liquids exert the same pressure at a given depth.
View Solution

