For energy density, power and intensity of any wave choose the correct options.
u=energy density=12ρω2A2
P=power=12ρω2A2Sv
I=intensity=12ρω2A2Sv
I=PS
The correct Answer is:A, D
To solve the question regarding the energy density, power, and intensity of any wave, we will analyze each option step by step.
Step 1: Analyze Energy Density
The first option states that energy density U is given by:
U=12ρω2A2
Where:
- ρ is the density of the medium,
- ω is the angular frequency,
- A is the amplitude of the wave.
This formula is indeed correct. Energy density represents the amount of energy stored in a unit volume of the wave.
Conclusion for Step 1: Option A is correct.
Step 2: Analyze Power
The second option states that power P is given by:
P=12ρω2A2SV
Where:
- S is the cross-sectional area,
- V is the wave velocity.
Power can be calculated as the energy transferred per unit time. The energy transferred can be expressed as energy density multiplied by volume, and the volume can be represented as the cross-sectional area multiplied by distance traveled per unit time (which is the wave velocity).
Using the energy density from Step 1, we can express power as:
P=U⋅Volume=U⋅(S⋅V)=(12ρω2A2)(S⋅V)
Thus, this option is also correct.
Conclusion for Step 2: Option B is correct.
Step 3: Analyze Intensity
The third option states that intensity I is given by:
I=12ρω2A2SV
However, intensity is defined as power per unit area:
I=PA
Rewriting this using the expression for power from Step 2:
I=12ρω2A2SVS=12ρω2A2V
This shows that the expression given in option C is incorrect because it does not account for the correct relationship between intensity and power.
Conclusion for Step 3: Option C is incorrect.
Step 4: Verify Intensity Again
The fourth option states:
I=PS
This is indeed the correct definition of intensity, as it states that intensity is power per unit area. Since we have already established that power can be expressed in terms of energy density and area, this option is correct.
Conclusion for Step 4: Option D is correct.
Final Summary of Options
- Option A: Correct
- Option B: Correct
- Option C: Incorrect
- Option D: Correct
The first option states that energy density U is given by:
U=12ρω2A2
Where:
- ρ is the density of the medium,
- ω is the angular frequency,
- A is the amplitude of the wave.
The second option states that power P is given by:
P=12ρω2A2SV
Where:
- S is the cross-sectional area,
- V is the wave velocity.
P=U⋅Volume=U⋅(S⋅V)=(12ρω2A2)(S⋅V)
Thus, this option is also correct.
The third option states that intensity I is given by:
I=12ρω2A2SV
However, intensity is defined as power per unit area:
I=PA
Rewriting this using the expression for power from Step 2:
I=12ρω2A2SVS=12ρω2A2V
This shows that the expression given in option C is incorrect because it does not account for the correct relationship between intensity and power.
The fourth option states:
I=PS
This is indeed the correct definition of intensity, as it states that intensity is power per unit area. Since we have already established that power can be expressed in terms of energy density and area, this option is correct.
- Option A: Correct
- Option B: Correct
- Option C: Incorrect
- Option D: Correct
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Level 2 Subjective
7 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions
2 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Level 2 Single Correct
5 Videos
- VECTORSBOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
Choose the correct option(s)
View SolutionChoose the correct option (S)?
View SolutionRegarding stationary waves, choose the correct options.
View SolutionMatch the column and choose the correct option
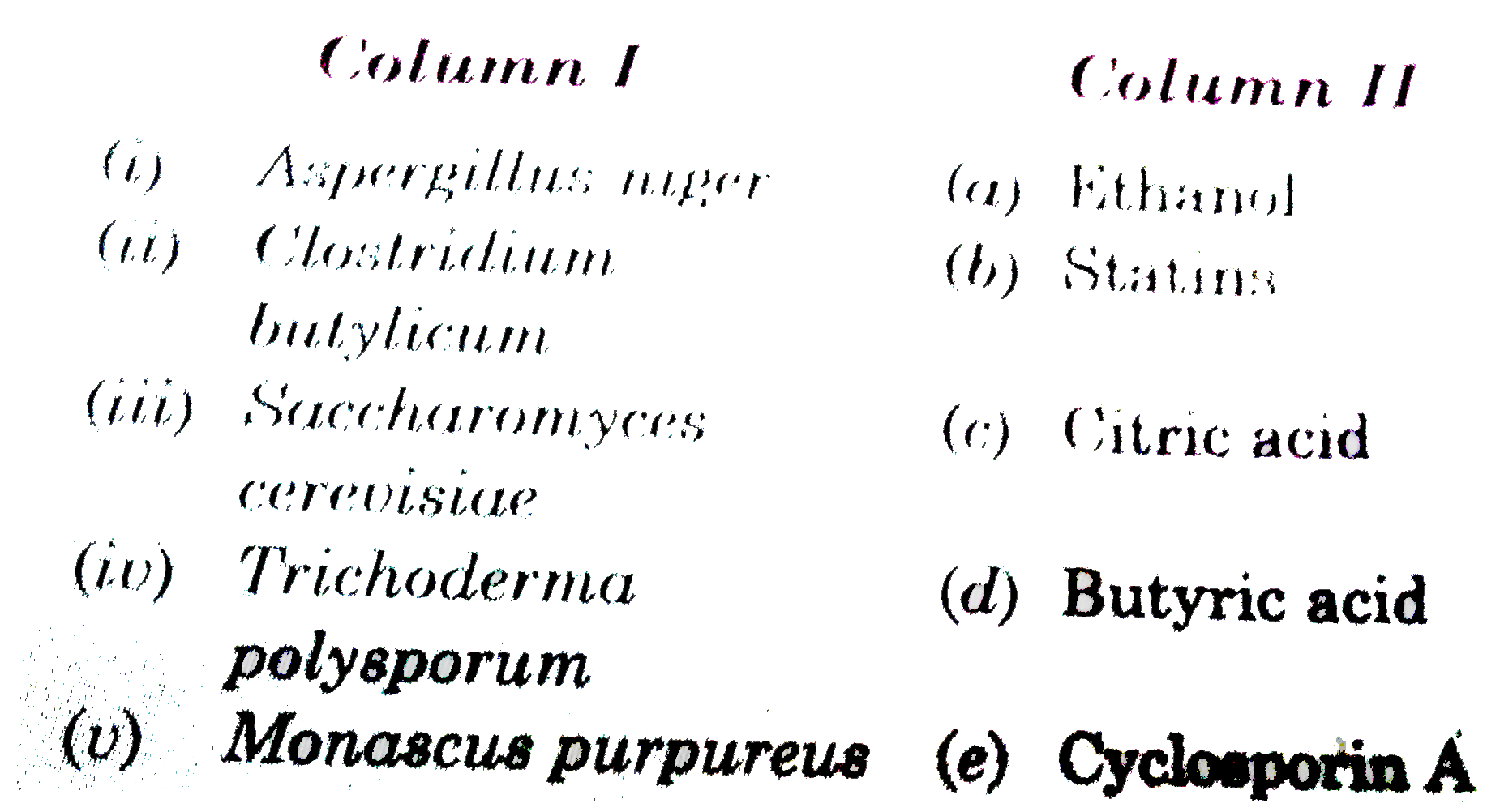
View SolutionObserve figures and choose the correct option.
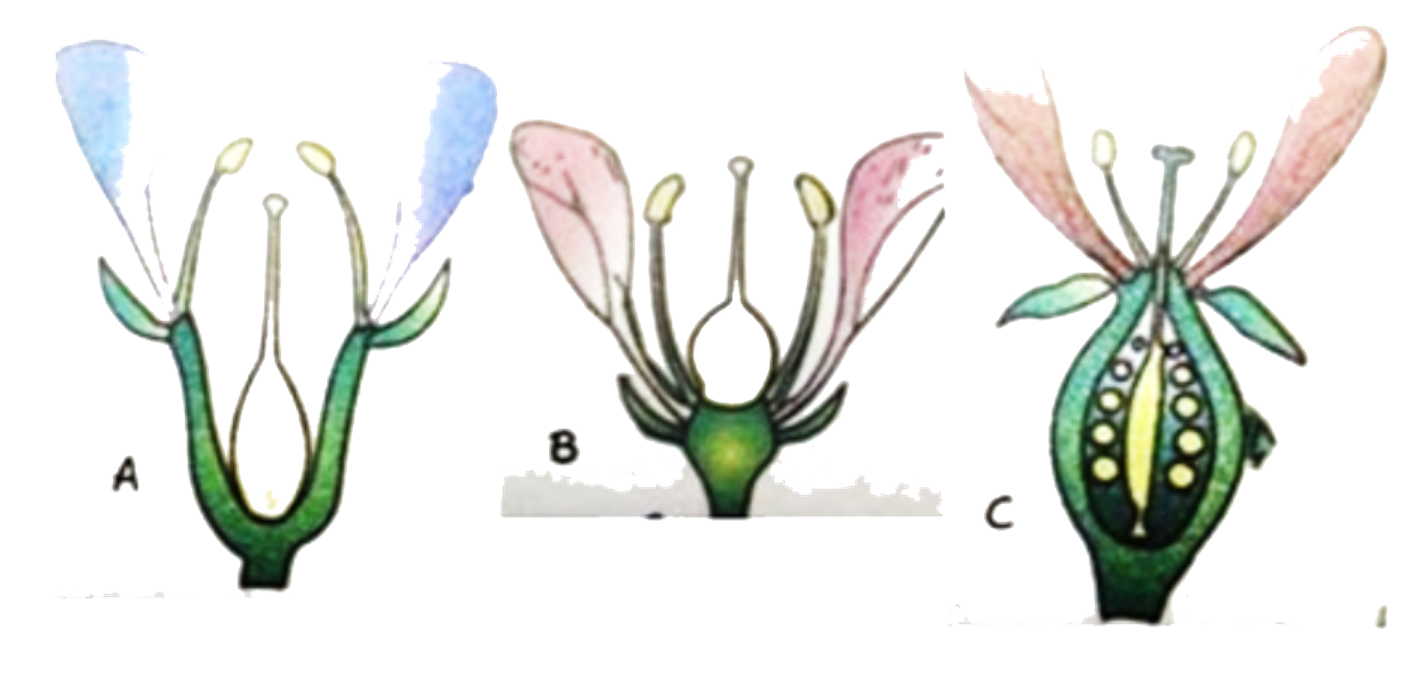
View SolutionRegarding a nucleus choose the correct options.
View SolutionFor all real values of θ, choose the correct options.
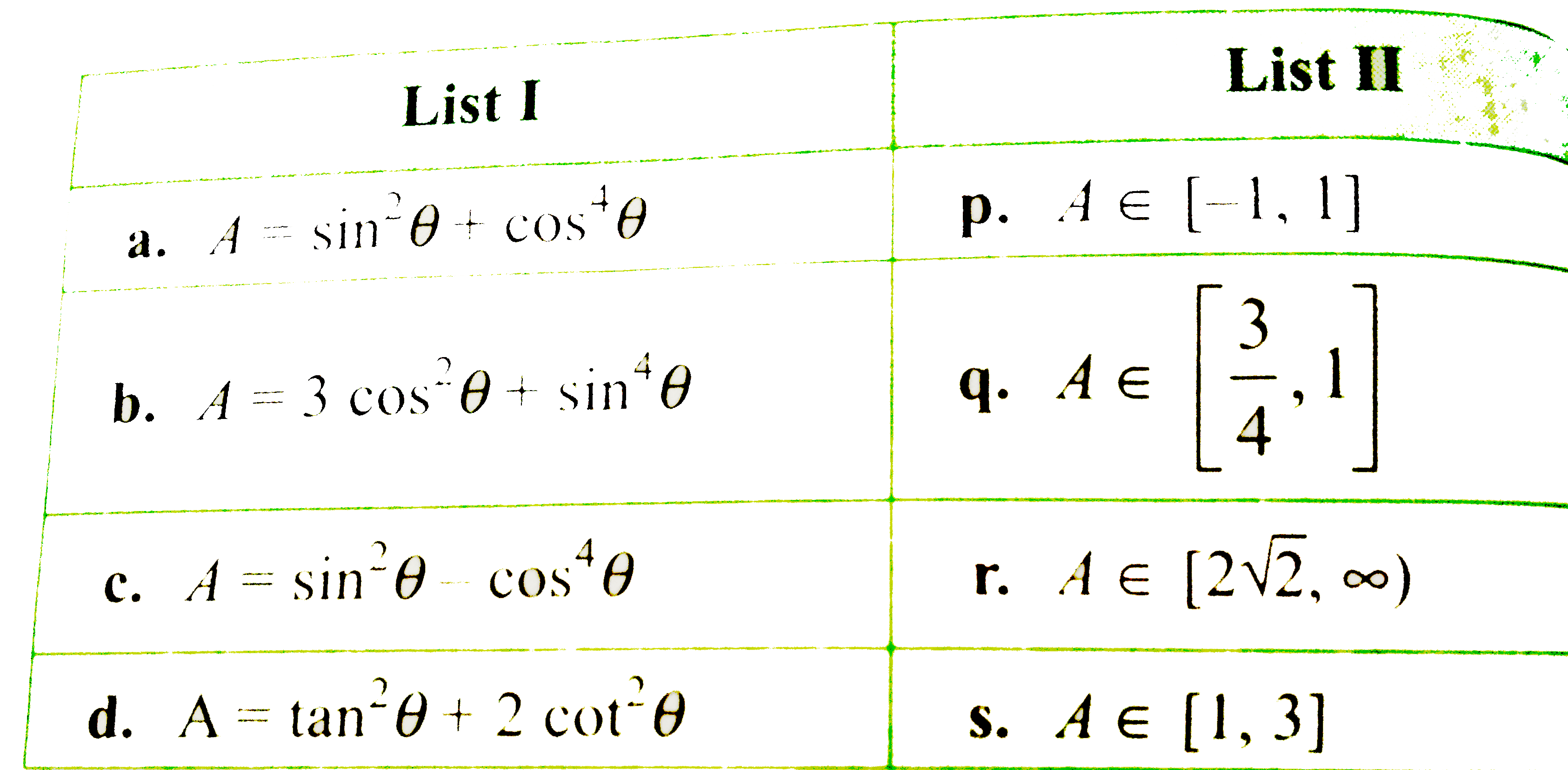
View SolutionMatch the columns and choose the correct option
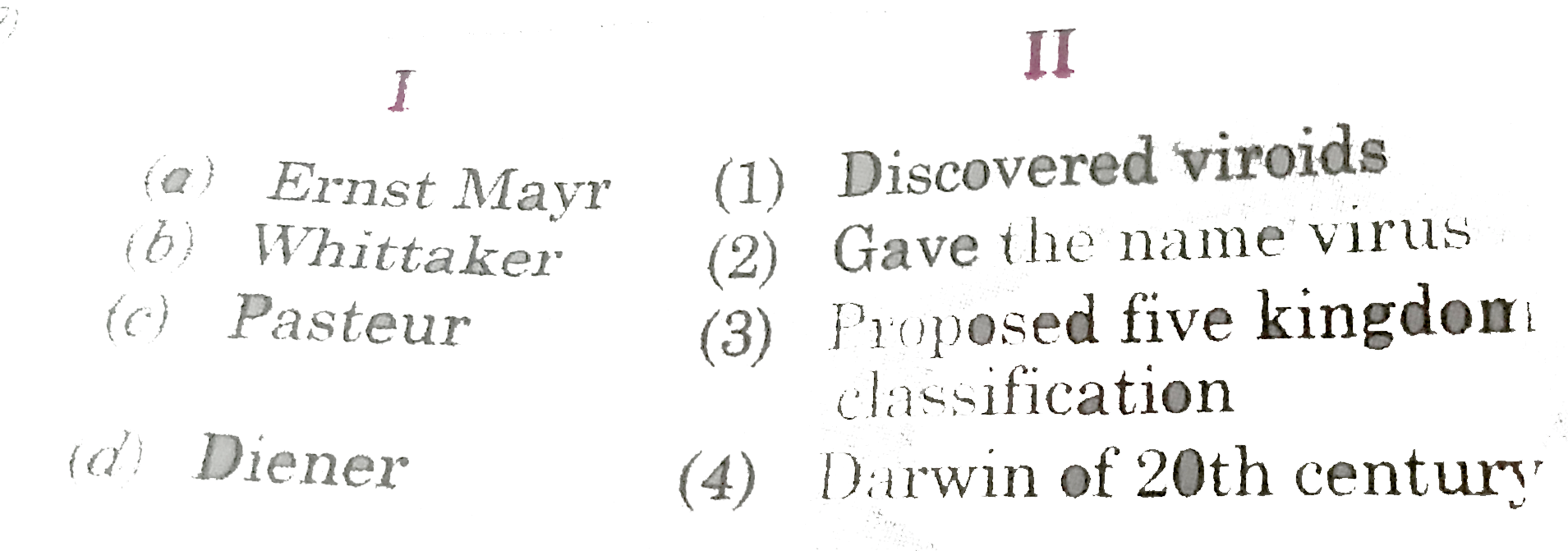
View SolutionMatch the columns and choose the correct option
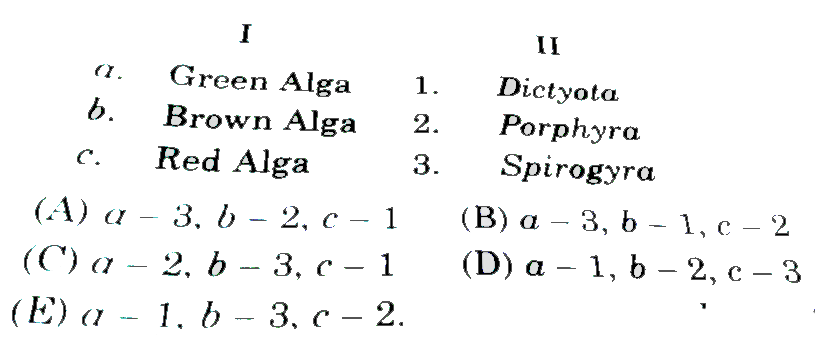
View SolutionMatch the columns and choose the correct option
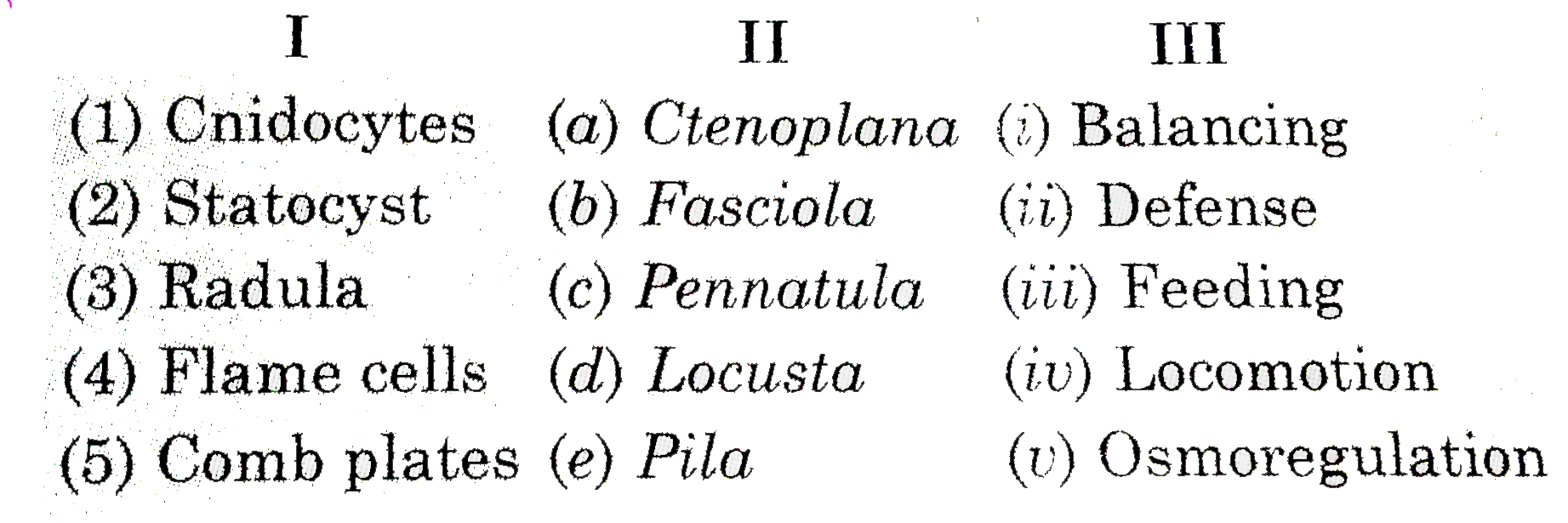
View Solution
