Three rods of the same mass are placed as shown in the figure. Calculate the coordinates of the centre of mass of the system.
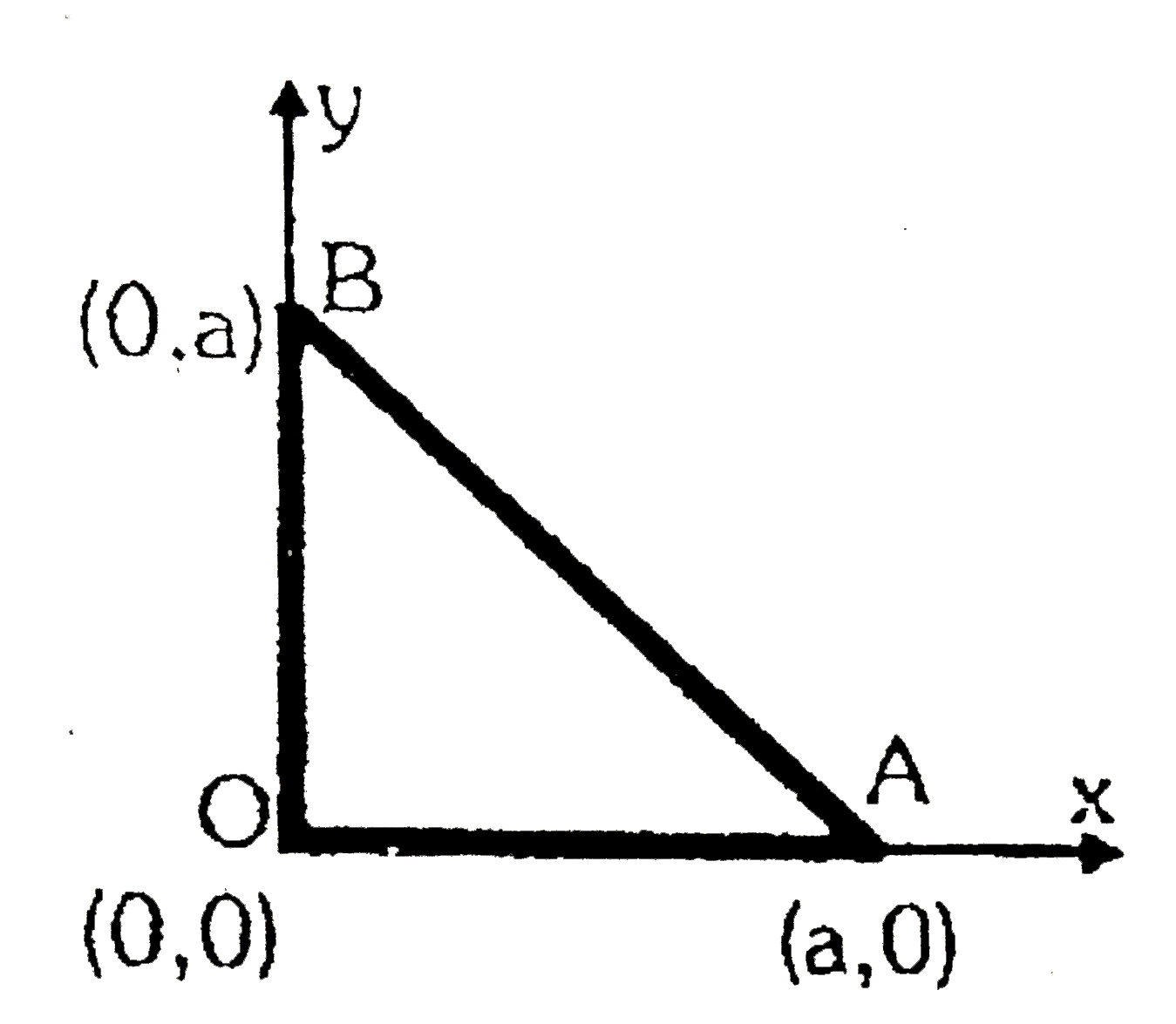
(a2,a2)
(a√2,a√2)
2a/3, 2a/3
a/3, a/3
The correct Answer is:A
xCM=m1+m2+m3m1+m2+m3
=(m)(a/2)+(m)(a2)+m(a2))m+m+m=a2
Similarly, yCM=a2
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Taking it together73videos
CENTRE OF MASS
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason21videos
CENTRE OF MASS
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery27videos
CENTRE OF MASS
- BOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery38videos
CALORIMETRY AND HEAT TRANSFER
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions15videos
CENTRE OF MASS, IMPULSE AND MOMENTUM
Similar Questions
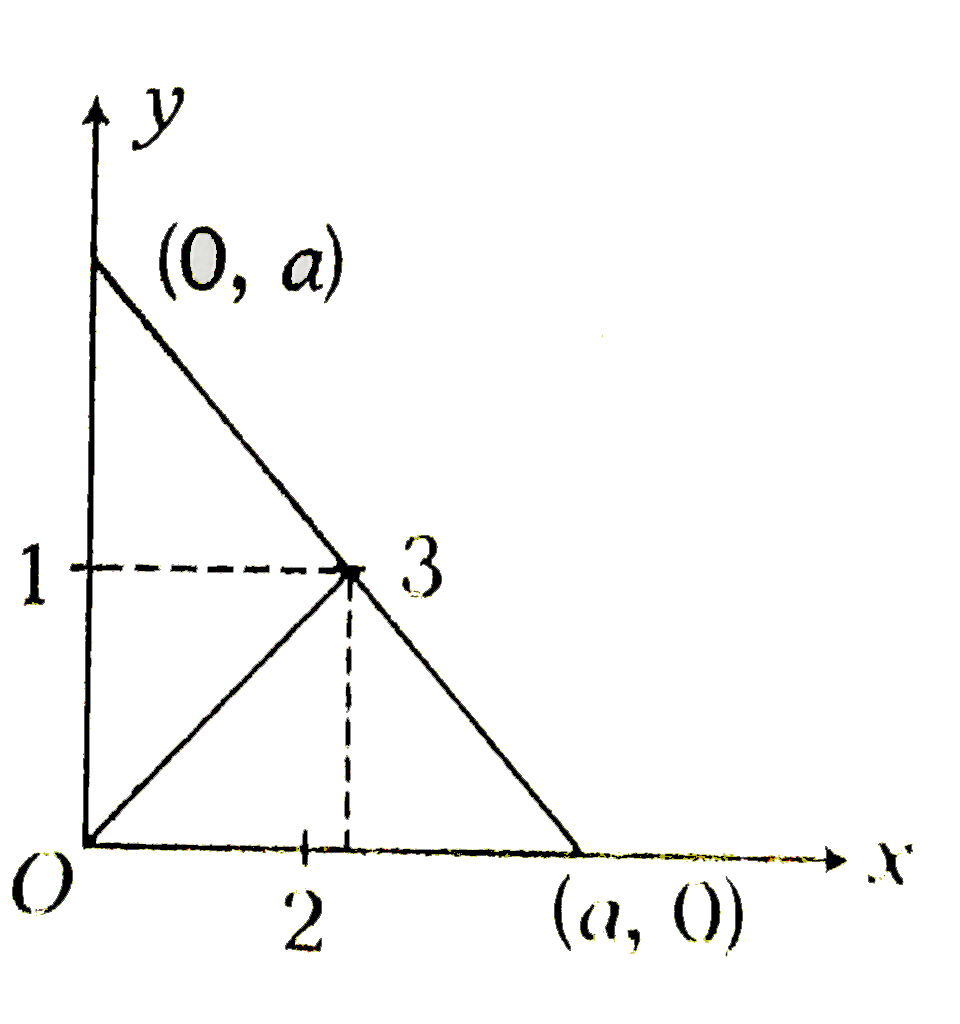
If three rods of same mass are placed as shown in the figure, the co-ordinates of the centre of mass of the system are
 View Solution
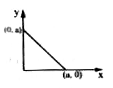
View SolutionCalculate centre of mass of the system
 View Solution
View SolutionFive masses are placed in a plane as shown in figure. The coordinates of the centre of mass are nearest to
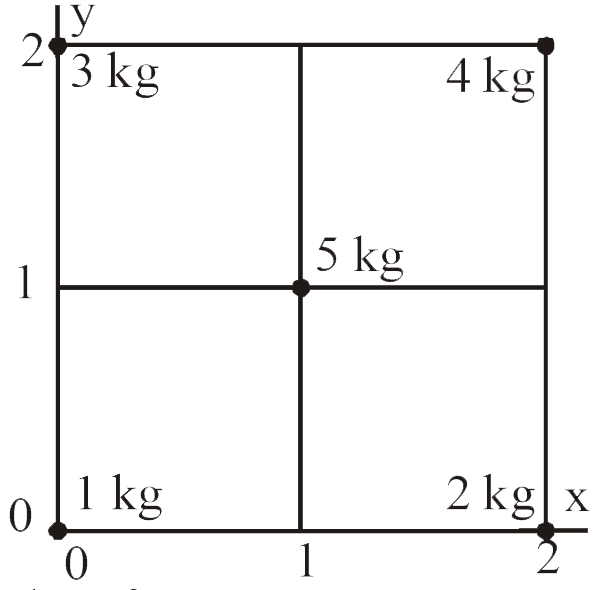 View Solution
View SolutionA uniform thin rod is bent in the form of closed loop ABCDEFA as shown in the figure. The y− coordinate of the centre of mass of the system is
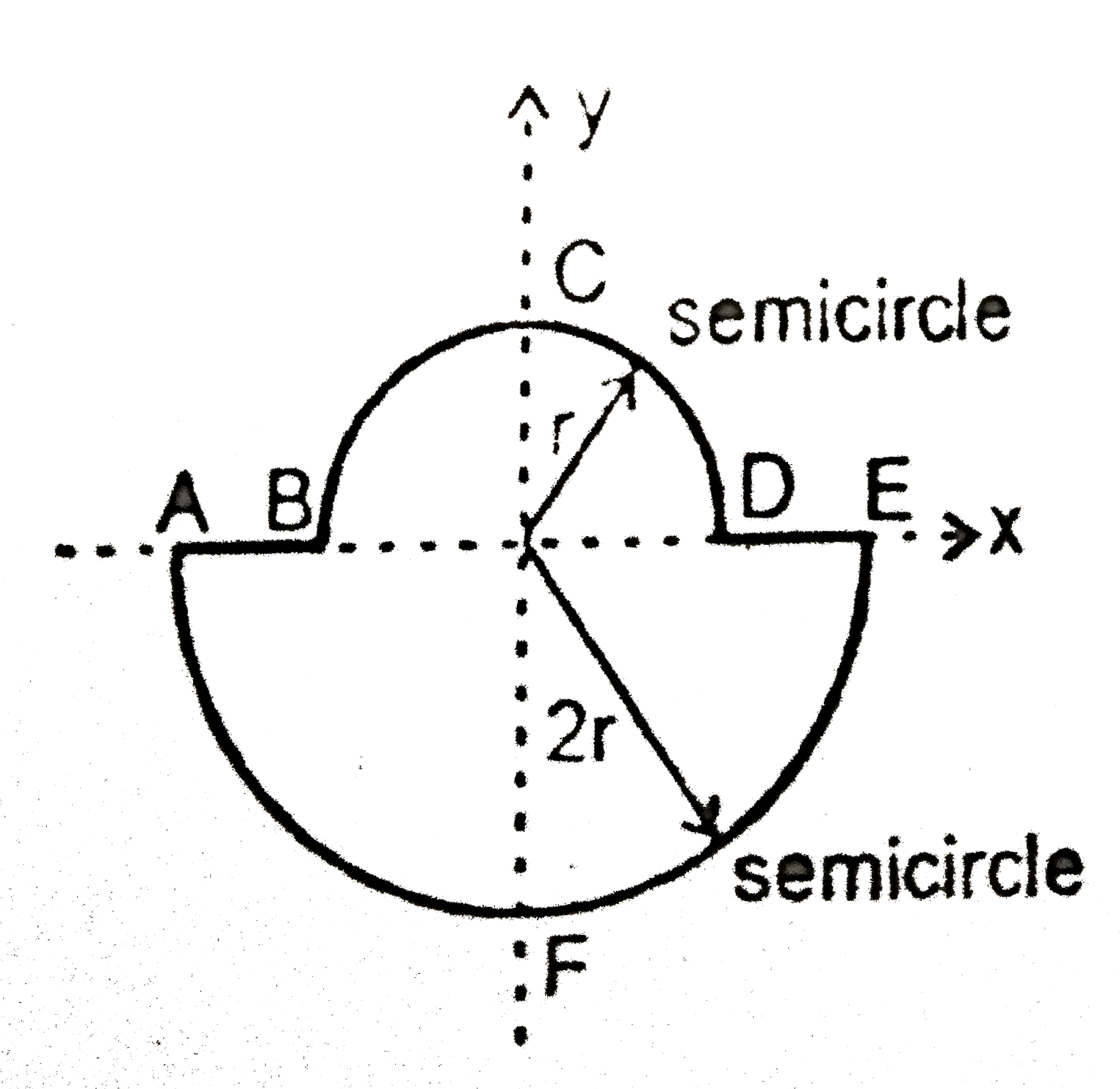 View Solution
View SolutionFrom a 16cm×8cm rectangular uniform plane sheet, exactly one quarter of the sheet is removed as shown in figure. If the origin be taken at the centre of the sheet considered as shown in the figure the coordinates of the centre of mass of the remaining sheet is
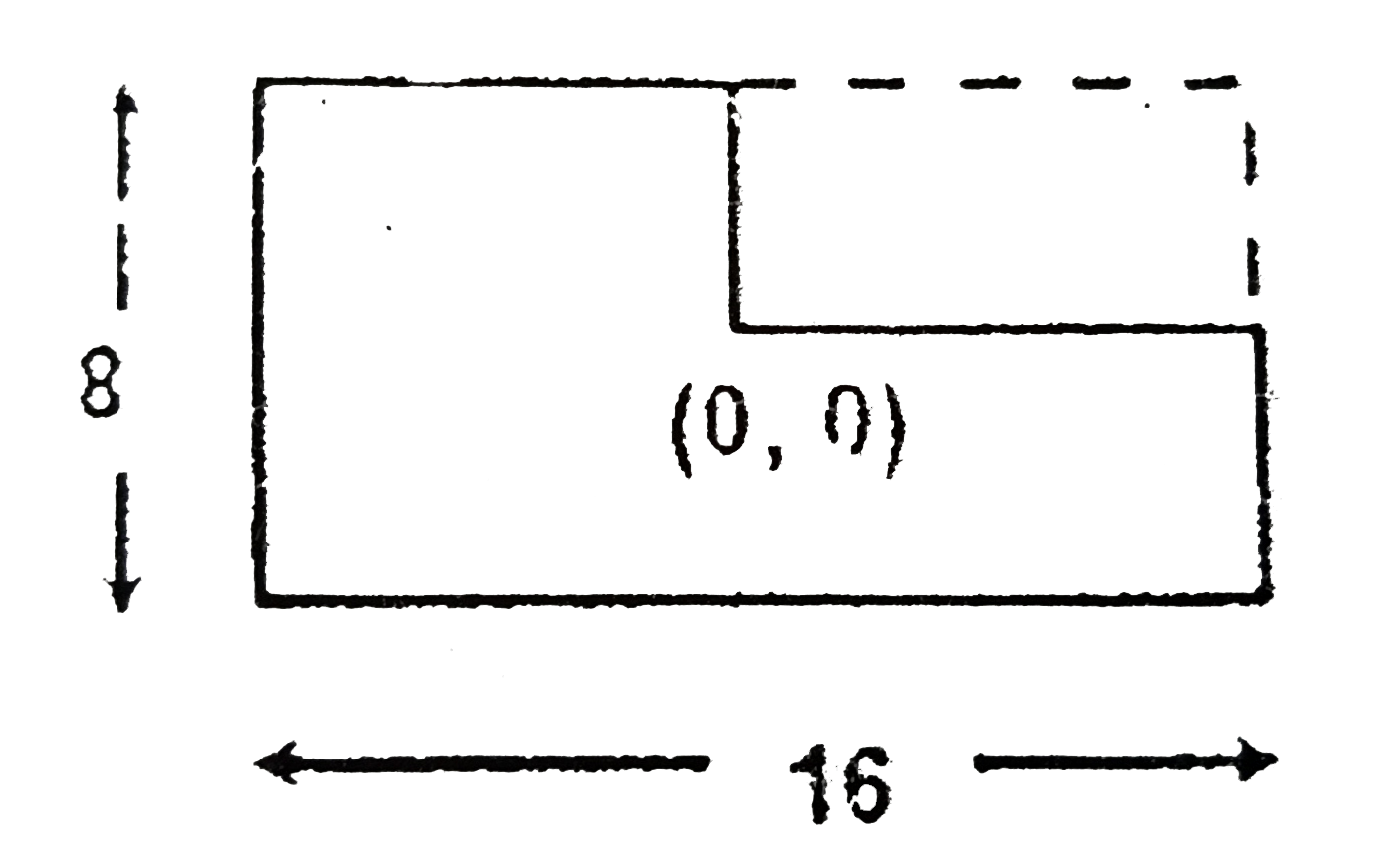 View Solution
View SolutionThe co-ordinates of the centre of mass of the system as shown in figure are
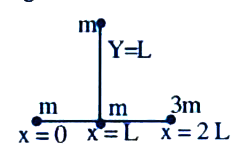 View Solution
View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O
 View Solution
View SolutionSeven particles, each of mass m are placed at the seven corners of a cube of side 'a', but one corner is vacant, as shown in figure. If the x−coordinates of the centre of mass of the system is nam. Where n and m are least integer values. Then find vlaue of m−n.
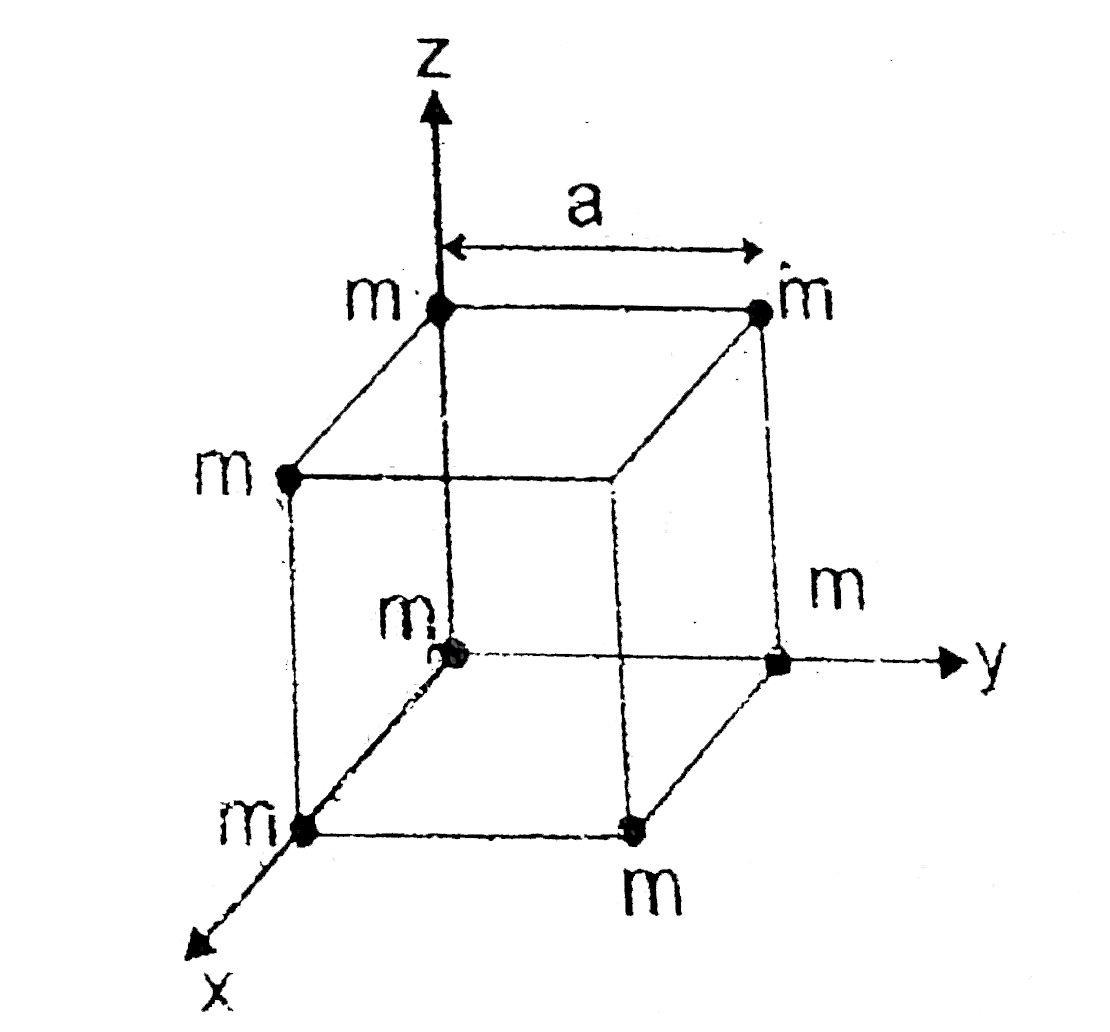 View Solution
View SolutionA rod of length 2l is bent as shown in figure. Coordinates of centre of mass are
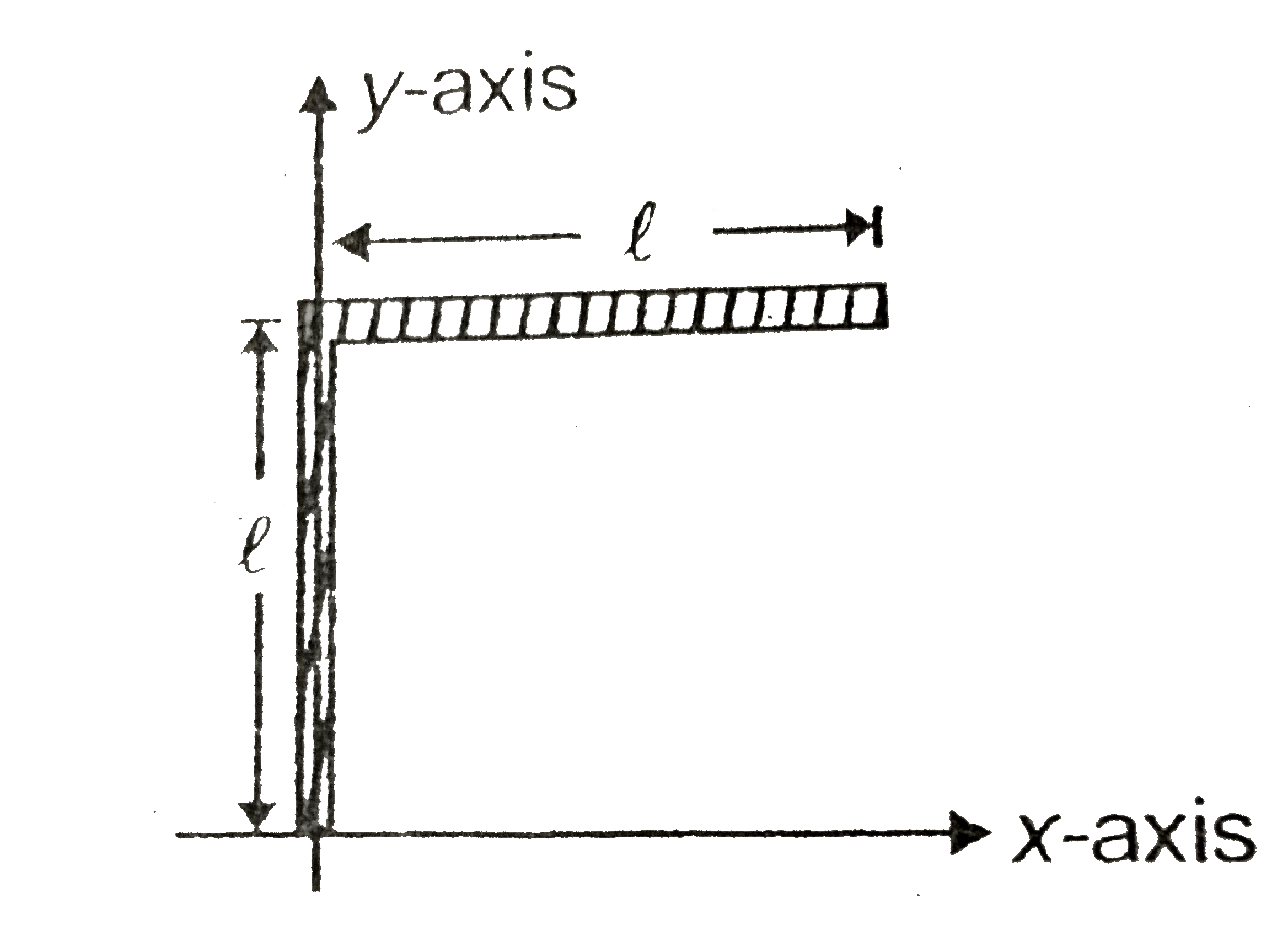 View Solution
View SolutionThree particles A(50g),B(100g) and C(150g) are placed at vertices of an equilateral triangle as shown in figure. Then the coordinates of center of mass of system is :
_2019_CBT8_E01_014_Q01.png) View Solution
View Solution

